Introduction
Introduction
Time and Work – Quiz 4 is one of important topic in Quantitative Aptitude Section. In Time and Work – Quiz 4 article candidates can find questions with an answer. By solving this questions candidates can improve and maintain, speed, and accuracy in the exams. Time and Work – Quiz 4 questions are very useful for different exams such as IBPS PO, Clerk, SSC CGL, SBI PO, NIACL Assistant, NICL AO, IBPS SO, RRB, Railways, Civil Services etc.
 Q1
Q1
A takes twice as much time as B or thrice as much time as C to finish a piece of work. Working together, they can finish the work in 2 days. B can do the work alone in:
- A. 4 days
B. 6 days
C. 8 days
D. 12 days
Suppose A, B and C take x, [latex]\frac{x}{2}[/latex]and [latex]\frac{x}{3}[/latex] days respectively to finish the work.
Then, [latex][\frac{1}{x} + \frac{2}{x} + \frac{3}{x}] = \frac{1}{2}[/latex]
[latex]\Rightarrow \frac{6}{x} = \frac{1}{2}[/latex]
[latex]\Rightarrow x = 12[/latex]
So, B takes [latex][\frac{12}{2}][/latex] = 6 days to finish the work.
 Q2
Q2
A and B together can do a piece of work in 30 days. A having worked for 16 days, B finishes the remaining work alone in 44 days. In how many days shall B finish the whole work alone?
- A. 8 days
B. 10 days
C. 60 days
D. 15 days
Let A's 1 day's work = x and B's 1 day's work = y.
Then, x + y =[latex]\frac{1}{30}[/latex] and 16x + 44y = 1.
Solving these two equations, we get: x = [latex]\frac{1}{60}[/latex] and y = [latex]\frac{1}{60}[/latex]
i.e, B's 1 day's work = [latex]\frac{1}{60}[/latex] .
Hence, B alone shall finish the whole work in 60 days.
 Q3
Q3
A works twice as fast as B. If B can complete a work in 12 days independently, the number of days in which A and B can together finish the work in :
- A. 4 days
B. 6 days
C. 8 days
D. 18 days
Ratio of rates of working of A and B = 2 : 1.
So, ratio of times taken = 1 : 2.
B's 1 day's work = [latex]\frac{1}{12}[/latex]
i.e, A's 1 day's work = [latex]\frac{1}{6}[/latex] ; (2 times of B's work)
(A + B)'s 1 day's work = [latex][\frac{1}{6} + \frac{1}{12}] = \frac{3}{12} = \frac{1}{4}[/latex]
So, A and B together can finish the work in 4 days.
 Q4
Q4
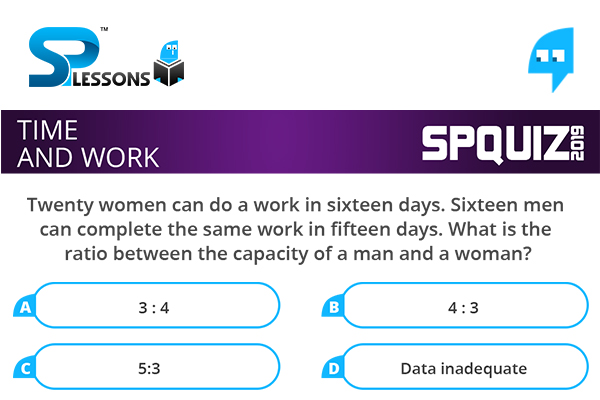
Twenty women can do a work in sixteen days. Sixteen men can complete the same work in fifteen days. What is the ratio between the capacity of a man and a woman?
- A. 3 : 4
B. 4 : 3
C. 5 : 3
D. Data inadequate
(20 x 16) women can complete the work in 1 day.
i.e, 1 woman's 1 day's work = [latex]\frac{1}{320}[/latex]
(16 x 15) men can complete the work in 1 day.
i.e, 1 man's 1 day's work = [latex]\frac{1}{240}[/latex]
So, required ratio = [latex]\frac{1}{240}[/latex] : [latex]\frac{1}{320}[/latex]
= [latex]\frac{1}{3}[/latex] = [latex]\frac{1}{4}[/latex]
= 4 : 3 (cross multiplied)
 Q5
Q5
A and B can do a work in 8 days, B and C can do the same work in 12 days. A, B and C together can finish it in 6 days. A and C together will do it in :
- A. 4 days
B. 6 days
C. 8 days
D. 12 days
(A + B + C)'s 1 day's work = [latex]\frac{1}{6}[/latex]
(A + B)'s 1 day's work = [latex]\frac{1}{8}[/latex]
(B + C)'s 1 day's work = [latex]\frac{1}{12}[/latex]
Therefore (A + C)'s 1 day's work = [latex][2 \times \frac{1}{6}][/latex] - [latex][\frac{1}{8} + \frac{1}{12}][/latex]
= [latex][\frac{1}{3} - \frac{5}{24}][/latex]
= [latex]\frac{3}{24}[/latex]
= [latex]\frac{1}{8}[/latex]
So, A and C together will do the work in 8 days.