Introduction
Introduction
Time and Work is one of important topic in Quantitative Aptitude Section. In Time and Work Quiz 14 article candidates can find a question with an answer. By solving this question candidates can improve and maintain, speed, and accuracy in the exams. Time and Work Quiz 14 questions are very useful for different exams such as IBPS PO, Clerk, SSC CGL, SBI PO, NIACL Assistant, NICL AO, IBPS SO, RRB, Railways, Civil Services etc.
 Q1
Q1
Reema can complete a piece of work in 12 days while Seema can the same work in 18 days. If they both work together, then how many days will be required to finish the work?
- A. 6 days
B. 7.2 days
C. 9.5 days
D. 12 days
A's one day work = [latex]\frac {1}{12}[/latex]
B's one day work = [latex]\frac {1}{18}[/latex]
(A + B)'s one day work = [latex]\frac {1}{12} + \frac {1}{18} = \frac {(18 + 12)}{(12 \times 18)} = \frac {30}{216} = \frac {1}{7.2}[/latex]
Together, A & B will finish the work in 7.2 days.
 Q2
Q2
If 'A' completes a piece of work in 3 days, which 'B' completes it in 5 days and 'C' takes 10 days to complete the same work. How long will they take to complete the work, if they work together?
- A. 1.5 days
B. 4.5 days
C. 7 days
D. 9.8 days
A's one day work = [latex]\frac {1}{3}[/latex]
B's one day work = [latex]\frac {1}{5}[/latex]
C's one day work = [latex]\frac {1}{10}[/latex]
(A+ B+ C)'s one day work = [latex]\frac {1}{3} + \frac {1}{5} = \frac {1}{10} = \frac {1}{1.5} [/latex]
Hence, A ,B & C together will take 1.5 days to complete the work.
 Q3
Q3
Two painters 'P1' & 'P2' paint the bungalow in 3 days. If P1 alone can paint the bungalow in 12 days, in how many days can 'P2' alone complete the same paint work?
- A. 4 days
B. 6 days
C. 9 days
D. 12 days
If a person can do a part of work in 'n' days, then person's work in 1 day = [latex]\frac {1}{n}[/latex]
As painters P1 & P2 paint the bungalows in 3 days, then work done by both painters = [latex]\frac {1}{3}[/latex]
As P1 paint it alone in 12 days, then work done by painter P1 = [latex]\frac {1}{12}[/latex]
Work done by painter P2 = [latex]\frac {1}{3} - \frac {1}{12} = \frac {4 - 1}{12} = \frac {3}{12} = \frac {1}{4} [/latex]
Therefore, same work will be completed by painter P2 in 4 days.
 Q4
Q4
A & B can make paintings in 6 days, B & C can can make those paintings in 10 days. If A, B & C together can finish the work in 4 days, then A & C together will do it in ________ days.
- A. 4 (2/7) days
B. 4 days
C. 8 days
D. 10 days
We are given that, A,B, & C together complete the work in 4 days.
We can write, (A + B + C)'s 1 day work = [latex]\frac {1}{4}[/latex]
Similarly, (A+B) 's 1 day work = 1/6 days & (B+C)'s 1 day work = [latex]\frac {1}{10}[/latex]
Since the work is divided in combination and we are asked to find out the combined work of (A + C), so we can find out,
(A + C)'s 1 day work = [2 (A+B+C)'s 1 day work] – [(A+ B) 's 1 day work + (B+C)'s 1 day work]
[latex][2 \times \frac {1}{4}] - [(\frac {1}{6}) + (\frac {1}{10})][/latex]
[latex][ \frac {1}{2}] - \frac {16}{60} = \frac {1}{2} - \frac {4}{15} = \frac {7}{30}[/latex]
Hence, A & C together can complete the work in [latex]\frac {30}{7} days = 4 \frac {2}{7} days[/latex]
 Q5
Q5
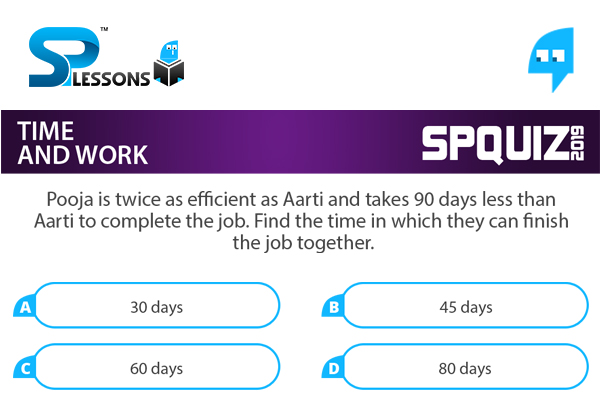
Pooja is twice as efficient as Aarti and takes 90 days less than Aarti to complete the job. Find the time in which they can finish the job together.
- A. 30 days
B. 45 days
C. 60 days
D. 80 days
Hint:
Assume that Pooja completes the job in 'x' days.
So, Aarti will take '2x' days to complete the same job.
As Pooja takes 90 days less than Aarti, we get
x = 2x – 90
By solving this equation, we get x = 90 .
Thus, 2x = 2 x 90 = 180
Part of job done by Pooja in 1 day = [latex]\frac {1}{90}[/latex]
Part of job done by Aarti in 1 day = [latex]\frac {1}{180}[/latex]
(Part of job done together in 1 day) = (Part of job done by Pooja in 1 day) + (Part of job done by Aarti in 1 day)
= [latex]\frac {1}{90} + \frac {1}{180}[/latex]
= [latex]\frac {3}{180}[/latex]
=[latex]\frac {1}{60}[/latex]
([latex]{(\frac {1}{60})}^{th}[/latex]) part of whole job will be completed by Pooja and Aarti together in one day.
Therefore, the whole job will be completed in 60 days together.