Introduction
Introduction
Time and Work is one of important topic in Quantitative Aptitude Section. In Time and Work Quiz 13 article candidates can find questions with an answer. By solving this questions candidates can improve and maintain, speed, and accuracy in the exams. Time and Work Quiz 13 questions are very useful for different exams such as IBPS PO, Clerk, SSC CGL, SBI PO, NIACL Assistant, NICL AO, IBPS SO, RRB, Railways, Civil Services etc.
 Q1
Q1
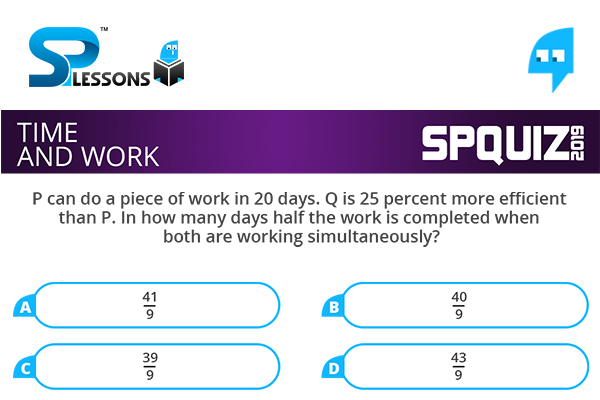
P can do a piece of work in 20 days. Q is 25 percent more efficient than P. In how many days half the work is completed when both are working simultaneously?
- A. [latex]\frac {41}{9}[/latex]
B. [latex]\frac {40}{9}[/latex]
C. [latex]\frac {39}{9}[/latex]
D. [latex]\frac {43}{9}[/latex]
Q is 25 percent more efficient so he will complete the work in 16 days
[latex](\frac {1}{20} + \frac {1}{16}) \times t = \frac {1}{2}[/latex]
[latex](\frac {4 + 5}{80}) \times t = \frac {1}{2}[/latex]
[latex]t = \frac {1}{2} \times \frac {80}{9}[/latex]
[latex]t = \frac {40}{9}[/latex]
 Q2
Q2
A does half as much work as B does in one sixth of the time. If together they take 20 days to complete the work, then what is the time taken by B to complete the work independently.
- A. 80 days
B. 100 days
C. 120 days
D. 140 days
Let B take X days to complete the work then in one – sixth of the time i.e. [latex]\frac {x}{6}[/latex] days. Now A do half work as done by B so A will take twice the time i.e. [latex]2 \times \frac {x}{6} = \frac {x}{3}[/latex] to complete the job alone
So [latex] \frac {1}{x} + \frac {3}{x} = \frac {1}{20}, x = 80[/latex] days
 Q3
Q3
A contractor undertakes to make a mall in 60 days and he employs 30 men. After 30 days it is found that only [latex]\frac{1}{3}[/latex] of the work is completed. How many extra men should he employ so that the work is completed on time?
- A. 20men
B. 25men
C. 30men
D. 40men
Let total work is w and it is given that one-third of the work is completed after 30 days. Means
[latex]M \times D = 30 \times 30 = \frac {w}{3}[/latex], so total work = [latex]30 \times 30 \times 3[/latex]
[latex]2700 = 30 \times 30 + (30 + p) \times 30[/latex], so we get P = 30 (p = additional men)
 Q4
Q4
P and Q were assigned to do a work for an amount of 1200. P alone can do it in 15 days while Q can do it in 12 days. With the help of R they finish the work in 6 days. Find the share if C.
- A. 100
B. 120
C. 140
D. 160
[latex]\frac {1}{15} + \frac {1}{12} + \frac {1}{C} = \frac {1}{6}[/latex], we got C = 60 (it means C will take 60 days to complete the work alone)
So ratio of work done by P : Q : R = 4 : 5 : 1
So c share = [latex](\frac {1}{10}) \times 1200 = 120[/latex]
 Q5
Q5
P does half as much work as Q in three-fourth of the time. If together they take 24 days to complete the work, how much time shall P take to complete the work?
- A. 50 days
B. 60 days
C. 70 days
D. 80 days
Let Q take x days to complete the work, so P will take [latex]2 \times \frac {3}{4}[/latex] of X day to complete the work i.e. [latex]\frac {3x}{2}[/latex] days
[latex]\frac {1}{x} + \frac {2}{3x} = \frac {1}{24}[/latex], we get x = 40 days, so P will take = [latex]\frac {3}{2}[/latex] of 40 = 60 days