Introduction
Introduction
Speed and Time is one of important topic in Quantitative Aptitude Section. In Speed and Time – Quiz 3 article candidates can find a question with an answer. By solving this question candidates can improve and maintain, speed, and accuracy in the exams. Speed and Time – Quiz 3 questions are very useful for different exams such as IBPS PO, Clerk, SSC CGL, SBI PO, NIACL Assistant, NICL AO, IBPS SO, RRB, Railways, Civil Services etc.
 Q1
Q1
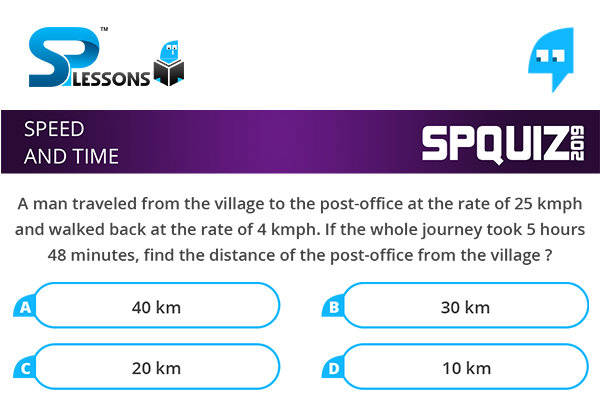
A man traveled from the village to the post-office at the rate of 25 kmph and walked back at the rate of 4 kmph. If the whole journey took 5 hours 48 minutes, find the distance of the post-office from the village ?
- A. 40 km
B. 30 km
C. 20 km
D. 10 km
Average speed = [latex]\frac {2ab}{a + b}[/latex], here a = 25 b = 4
= 2 x 25 x [latex]\frac {4}{(25 + 4)}[/latex] = [latex]\frac {200}{29}[/latex] km/hr.
Distance covered in 5 hours 48 minutes
= Speed x time = ([latex]\frac {200}{29}[/latex])x ([latex]\frac {29}{5}[/latex]) Distance covered in 5 hours 48 minutes = 40 kms.
Distance of the post office from the village = ([latex]\frac {40}{2}[/latex]) = 20 km.
 Q2
Q2
Two boys starting from the same place walk at a rate of 5kmph and 5.5kmph respectively. What time will they take to be 8.5km apart, if they walk in the same direction?
- A. 15 hours
B. 16 hours
C. 17 hours
D. 18 hours
In this type of questions we need to get the relative speed between them,
The relative speed of the boys = 5.5 kmph – 5 kmph
= 0.5 kmph
Distance between them is 8.5 km
Time = [latex]\frac {dist}{speed}[/latex]
Time= [latex]\frac {8.5 km}{ 0.5 kmph }[/latex] = 17 hrs
 Q3
Q3
A man reaches his office 20 min late, if he walks from his home at 3 km per hour and reaches 30 min early if he walks 4 km per hour. How far is his office from his house ?
- A. 20 km
B. 16 km
C. 14 km
D. 10 km
Let distance = x km.
Time taken at 3 kmph : [latex]\frac {dist}{speed}[/latex] = [latex]\frac {x}{3}[/latex] = 20 min late.
time taken at 4 kmph : [latex]\frac {x}{4}[/latex] = 30 min earlier
difference between time taken : 30 -(-20) = 50 mins = [latex]\frac {50}{60}[/latex] hours.
[latex]\frac {x}{3}[/latex] - [latex]\frac {x}{4}[/latex] = [latex]\frac {50}{60}[/latex]
[latex]\frac {x}{12}[/latex] = [latex]\frac {5}{6}[/latex]
x = 10 km.
 Q4
Q4
A train covers a distance in 50 minutes, if it runs at a speed of 48 kmph on an average. Find the speed at which the train must run to reduce the time of journey to 40 minutes.
- A. 50 km/hr
B. 60 km/hr
C. 65 km/hr
D. 70 km/hr
We are having time and speed given, so first we will calculate the distance. Then we can get new speed for given time and distance.
Time = [latex]\frac {50}{60}[/latex] hr = [latex]\frac {5}{6}[/latex] hr
Speed = 48 mph
Distance = S [latex]\times[/latex] T = 48 [latex]\times \frac {5}{6}[/latex] = 40 km
New time will be 40 minutes so,
Time = [latex]\frac {40}{60}[/latex] hr = [latex]\frac {2}{3}[/latex] hr
Now we know,
Speed = [latex]\frac {distance}{time}[/latex]
New speed = [latex]\frac {40 \times 3}{2}[/latex] kmph = 60 kmph
 Q5
Q5
The distance from town A to town B is five miles. C is six miles from B. Which of the following could be the maximum distance from A to C?
- A. 11
B. 8
C. 2
D. 4
Do not assume that AB and C are on a straight line. Make a diagram with A and B marked 5 miles apart. Draw a circle centered on B, with radius 6. C could be anywhere on this circle. The minimum distance will be 1, and maximum 11