Introduction
Introduction
Ratios and Proportions is one of important topic in Quantitative Aptitude Section. In Ratios and Proportions – Quiz 3 article candidates can find a question with an answer. By solving this question candidates can improve and maintain, speed, and accuracy in the exams. Ratios and Proportions – Quiz 3 questions are very useful for different exams such as IBPS PO, Clerk, SSC CGL, SBI PO, NIACL Assistant, NICL AO, IBPS SO, RRB, Railways, Civil Services etc.
 Q1
Q1
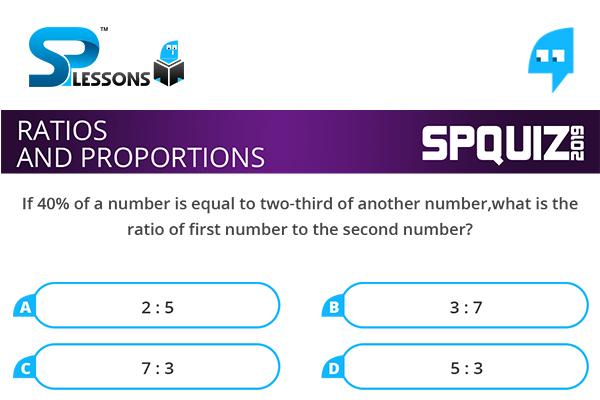
If 40% of a number is equal to two-third of another number, what is the ratio of first number to the second number?
- A. 2 : 5
B. 3 : 7
C. 7 : 3
D. 5 : 3
let 40% of A = [latex]\frac{2}{3}[/latex]
Then [latex]\frac{40 A}{100}[/latex] = [latex]\frac{2 B}{3}[/latex]
[latex]\Rightarrow \frac{2A}{5}[/latex] = [latex]\frac{2 B}{3}[/latex]
[latex]\Rightarrow[/latex] A : B = 5 : 3
 Q2
Q2
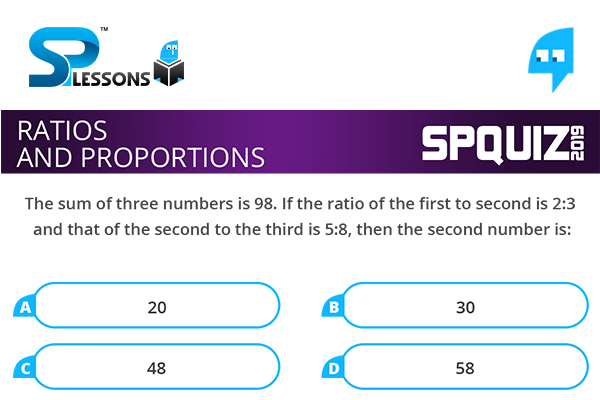
The sum of three numbers is 98. If the ratio of the first to second is 2:3 and that of the second to the third is 5:8, then the second number is:
- A. 20
B. 30
C. 48
D. 58
Let the three parts be A, B, C. Then,
A : B = 2 : 3 and B : C = 5 : 8 = 5 × [latex]\frac{3}{5}[/latex] : 8 [latex]\times \frac{3}{5}[/latex] = 3 : [latex]\frac{24}{5}[/latex]
A : B : C = 2 : 3 : [latex]\frac{24}{5}[/latex] = 10 : 15 : 24
[latex]\Rightarrow[/latex] B = 98 [latex]\times \frac{15}{49}[/latex] = 30
 Q3
Q3
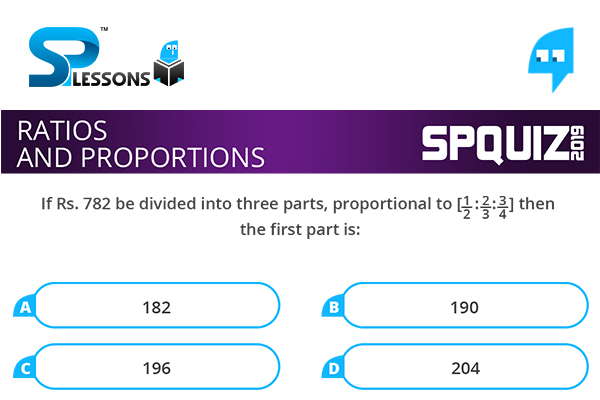
If Rs. 782 be divided into three parts, proportional to [latex][\frac{1}{2} : \frac{2}{3} : \frac{3}{4}][/latex] then the first part is:
- A. 182
B. 190
C. 196
D. 204
Given ratio = [latex][\frac{1}{2} : \frac{2}{3} : \frac{3}{4}][/latex] = 6 ; 8 : 9
The first part is Rs 782 × [latex]\frac{6}{23}[/latex] = Rs 204
 Q4
Q4
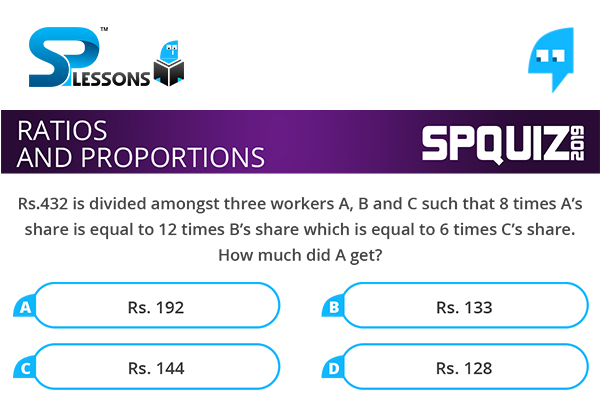
Rs.432 is divided amongst three workers A, B and C such that 8 times A’s share is equal to 12 times B’s share which is equal to 6 times C’s share. How much did A get?
- A. Rs. 192
B. Rs. 133
C. Rs. 144
D. Rs. 128
8 times A’s share = 12 times B’s share = 6 times C’s share
Note that this is not the same as the ratio of their wages being 8 : 12 : 6
In this case, find out the L.C.M of 8, 12 and 6 and divide the L.C.M by each of the above numbers to get the ratio of their respective shares.
The L.C.M of 8, 12 and 6 is 24.
Therefore, the ratio A : B : C is
[latex]\frac{24}{8}[/latex] : [latex]\frac{24}{12}[/latex] : [latex]\frac{24}{6}[/latex] [latex]\Rightarrow[/latex] A : B : C :: 3 : 2 : 4
The sum of the total wages
= 3x + 2x + 4x = 432
9x = 432 or x = 48.
Hence A gets 3 × 48= Rs 144
 Q5
Q5
If 20 men or 24 women or 40 boys can do a job in 12 days working for 8 hours a day, how many men working with 6 women and 2 boys take to do a job four times as big working for 5 hours a day for 12 days?
- A. 120 men
B. 122 men
C. 128 men
D. 134 men
Let's try solving this Problem using ratio approach.
Amount of work done by 20 men = 24 women = 40 boys or 1 man = 1.2 woman = 2 boys.
Let us, therefore, find out the amount of men required, if only men were working on the job, to complete the new job under the new conditions and then make adjustments for the women and children working with the men.
The man hours required to complete the new job = 4 times the man hours required to complete the old job. (As the new job is 4 times as big as the old job)
Let n be the number of men required.
n × 5 × 12 = 20 × 8 × 12 × 4 = or n = 128
i.e. 128 men working on the job will be able to complete the given job.
However, the problem states that 6 women and 2 boys are working on the job.
6 women $ = [latex]\frac{6}{1.2} [/latex] = 5 men and 2 boys = 1 man.
i.e, The equivalent of 5 + 1 = 6 men are already working.
Thus, final number of men working,
= 128 − 6 = 122 men