Introduction
Introduction
Ratios and Proportions is one of important topic in Quantitative Aptitude Section. In Ratios and Proportions – Quiz 1 article candidates can find a question with an answer. By solving this question candidates can improve and maintain, speed, and accuracy in the exams. Ratios and Proportions - Quiz 1 questions are very useful for different exams such as IBPS PO, Clerk, SSC CGL, SBI PO, NIACL Assistant, NICL AO, IBPS SO, RRB, Railways, Civil Services etc.
 Q1
Q1
The salaries A, B, C are in the ratio 2:3:5. If the increments of 15%, 10% and 20% are allowed respectively in their salaries, then what will be new ratio of their salaries?
- A. 3 : 3 : 10
B. 10 : 11 : 20
C. 23 : 33 : 60
D. Cannot be determined
Let A = 2k, B = 3k and C = 5k
A's new salary = [latex](\frac {115}{100} \times 2k) = \frac {23 k}{10}[/latex]
B's new salary = [latex](\frac {120}{100} \times 5k) = \frac {33 k}{10}[/latex]
C's new salary = [latex](\frac {115}{100} \times 2k) = 6k[/latex]
New ratio = ([latex]\frac {23 k}{10} [/latex] : [latex] \frac {33 k}{10}[/latex] : 6k) = 23 : 33 : 60
 Q2
Q2
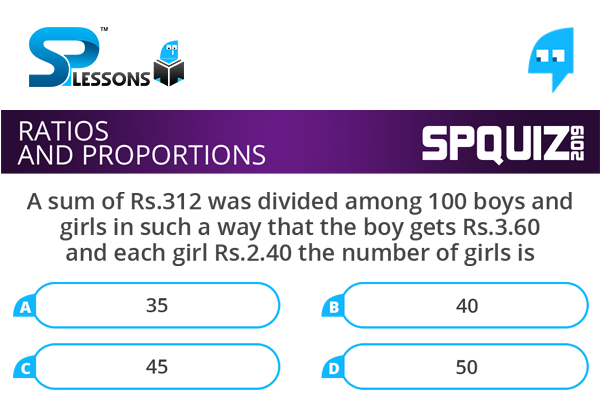
A sum of Rs.312 was divided among 100 boys and girls in such a way that the boy gets Rs.3.60 and each girl Rs.2.40 the number of girls is
- A. 35
B. 40
C. 45
D. 50
Step (i) Let x be the number of boys and y be the number of girls.
Given total number of boys and girls = 100
x + y = 100
Step (ii) A boy gets Rs. 3.60 and a girl gets Rs. 2.40
The amount given to 100 boys and girls = Rs. 312
3.6x + 2.4y =312
Step (iii)
Solving (i) and (ii)
3.6x +3.6y = 360 multiplying by 3.6
3.6x+2.4y1.20yy=312=48=40
The number of girls is 40.
 Q3
Q3
In a mixture 60 litres, the ratio of milk and water 2:1. If the this ratio is to be 1:2, then the quantity of water to be further added is:
- A. 20 litres
B. 30 litres
C. 40 litres
D. 60 litres
Quantity of milk = 60 [latex]\times \frac{2}{3} [/latex] = 40 litres
Quantity of water in it =(60−40) litres =20 litres.
New ratio =1:2
Let, the quantity of water to be added further be x litres.
Then milk : water = [latex] \frac{40}{20 + x} [/latex]
Now, [latex]\frac{40}{20 + x} = \frac{1}{2} [/latex]
20 + x = 80
[latex]\Rightarrow[/latex]x = 60
 Q4
Q4
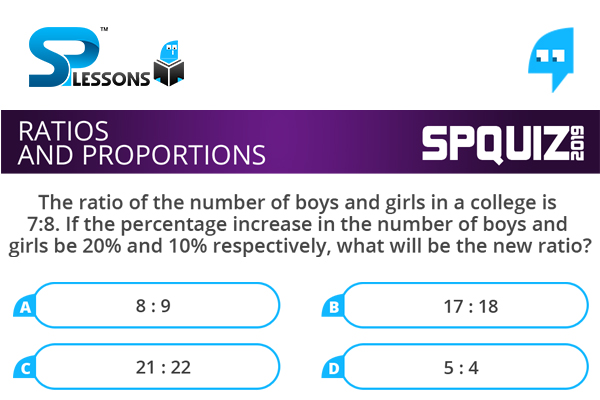
The ratio of the number of boys and girls in a college is 7:8. If the percentage increase in the number of boys and girls be 20% and 10% respectively, what will be the new ratio?
- A. 8 : 9
B. 17 : 18
C. 21 : 22
D. 5 : 4
Originally, let the number of boys and girls in the college be 7x and 8x respectively.
Their increased number is (120 % of 7x) and (110 % of 8x).
[latex]\Rightarrow (\frac{120 }{100} \times 7x)[/latex] and [latex](\frac{110}{100}\times 8x) [/latex]
[latex]\Rightarrow \frac{42 x}{5}[/latex] and [latex]\frac{44x}{5} [/latex]
So, the required ratio = ([latex] \frac{42 x}{5}[/latex] : [latex]\frac{44x}{5} [/latex]) = 21 : 22
 Q5
Q5
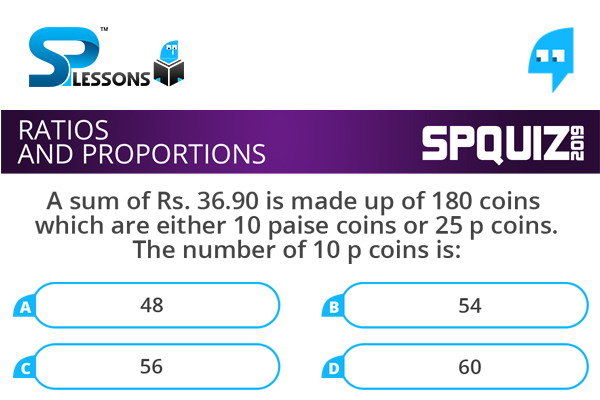
A sum of Rs. 36.90 is made up of 180 coins which are either 10 paise coins or 25 p coins. The number of 10 p coins is:
- A. 48
B. 54
C. 56
D. 60
Step (i) Total number of coins = 180
Let x be number of 10p coins and y be number of 25p coins
x + y = 180 --------(i)
Step (ii) Given 10p coins and 25p coins make the sum = Rs. 36.90
[latex]\frac{10 x}{100} + \frac{25 y}{100} = 36.9 [/latex]
[latex]\Rightarrow[/latex]10x + 25y = 3690-----(ii)
Step (iii)
Solving (i) and (ii),
10x + 10y = 1800
10x + 25y = 3600
-15y = 1890
y = 126
Substitute y value in equation (i)
x = 180 – 126 = 54
Number of 10p coins = 54