Introduction
Introduction
Ratios and Proportions is one of important topic in Quantitative Aptitude Section. In Ratios and Proportions Quiz 12 article candidates can find questions with an answer. By solving this questions candidates can improve and maintain, speed, and accuracy in the exams. Ratios and Proportions Quiz 12 questions are very useful for different exams such as IBPS PO, Clerk, SSC CGL, SBI PO, NIACL Assistant, NICL AO, IBPS SO, RRB, Railways, Civil Services etc.
 Q1
Q1
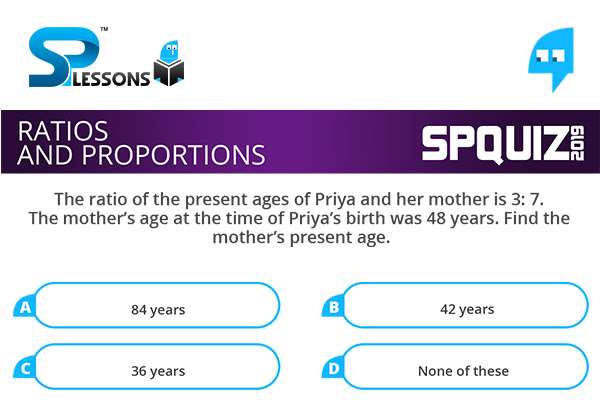
The ratio of the present ages of Priya and her mother is 3: 7. The mother’s age at the time of Priya’s birth was 48 years. Find the mother’s present age.
- A. 84 years
B. 42 years
C. 36 years
D. None of these
Present ratio is 7 : 3.
∴ actual ages are 7x and 3x.
∴ 7x – 3x = 48 → x = 12.
Hence their present ages are 84 and 36 yrs.
 Q2
Q2
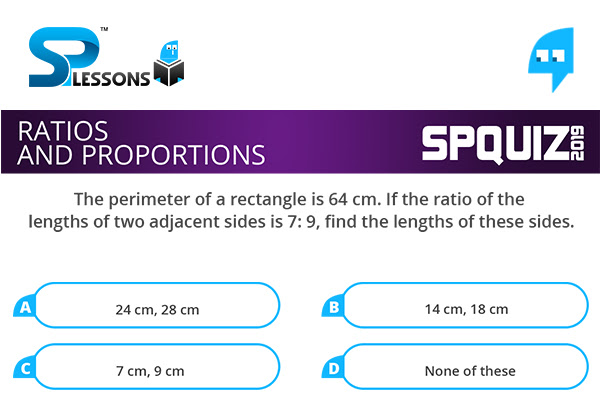
The perimeter of a rectangle is 64 cm. If the ratio of the lengths of two adjacent sides is 7: 9, find the lengths of these sides.
- A. 24 cm, 28 cm
B. 14 cm, 18 cm
C. 7 cm, 9 cm
D. None of these
Perimeter of a rectangle = 2 (L + B)
Also L: B = 7 : 9
∴ Actual values are 5x and 8x.
Hence 2(7x + 9x) = 64. → x = 2
∴ sides will be of 14 cm and 18 cm.
 Q3
Q3
The ratio of the length and the breadth of a rectangle is 3 : 5 and its area is 1.35 [latex]{cm}^{2}[/latex]. Find the length of the rectangle.
- A. 9 cm
B. 0.9 cm
C. 0.09 cm
D. 90 cm
Area of a rectangle = LB.
Ratio of sides = 3 : 5.
∴(3x)(5x) = 1.35 → x = 0.3.
→ Length of the rectangle
= 3 (0.3) = 0.9 cm
And breadth = 5(0.3) = 1.5 cm.
 Q4
Q4
The ratio of the present ages of John and Jim is 5 : 3. Four years hence it will be 3 : 2. Find the present age of John.
- A. 5 years
B. 12 years
C. 10 years
D. 20 years
Present ratio = 5 : 3.
∴ actual values are 5x and 3x.
So [latex] \frac {(5x + 4)}{(3x + 4)} = \frac {3}{2} → x = 4[/latex]
So present ages are 20 yrs and 12 yrs.
 Q5
Q5
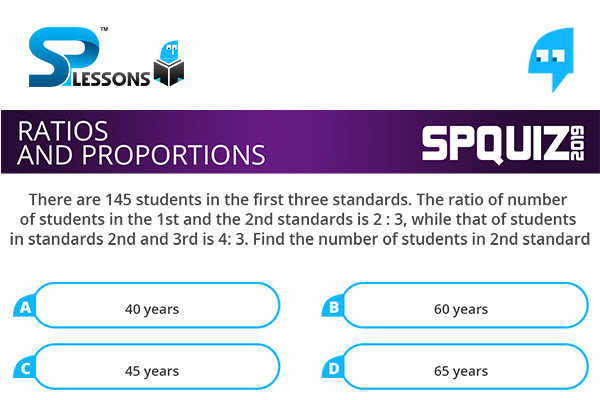
There are 145 students in the first three standards. The ratio of number of students in the 1st and the 2nd standards is 2 : 3, while that of students in standards 2nd and 3rd is 4: 3. Find the number of students in 2nd standard
- A. 40 years
B. 60 years
C. 45 years
D. 65 years
Total students = 145. Ratio of students in 1st and 2nd standards = 2 : 3
Ratio of students in 2nd and 3rd standards = 4 : 3
Hence combined ratio i.e. 1st: 2nd: 3rd is 8 : 12 : 9.
∴ Number of students in each standard
= [latex] \frac {(145 × 8)}{29} = 40[/latex]
[latex]\frac {(145 × 12)}{29} = 60 and \frac {(145 × 9)}{29} = 45.[/latex]