Introduction
Introduction
Quadratic Equation is one of important topic in Quantitative Aptitude Section. In Quadratic Equation – Quiz 6 article candidates can find questions with answer. By solving this questions candidates can improve and maintain, speed, and accuracy in the exams. Quadratic Equation - Quiz 6 questions are very useful for different exams such as IBPS PO, Clerk, SSC CGL, SBI PO, NIACL Assistant, NICL AO, IBPS SO, RRB, Railways, Civil Services etc.
 Q1
Q1
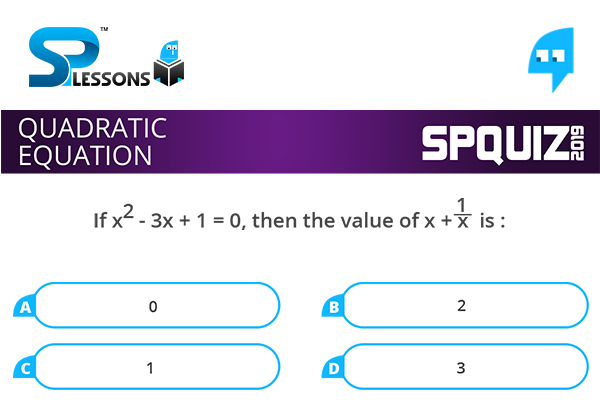
If [latex]{x}^{2} -3x + 1 = 0 [/latex], then the value of x + [latex]\frac {1}{x}[/latex] is :
- A. 0
B. 2
C. 1
D. 3
[latex]{x}^{2} -3x + 1 = 0 \Rightarrow {x}^{2} + 1 = 3x[/latex]
[latex]\Rightarrow \frac {{x}^{2} + 1}{x} = 3[/latex]
[latex]\Rightarrow x + \frac {1}{x}[/latex] = 3
 Q2
Q2
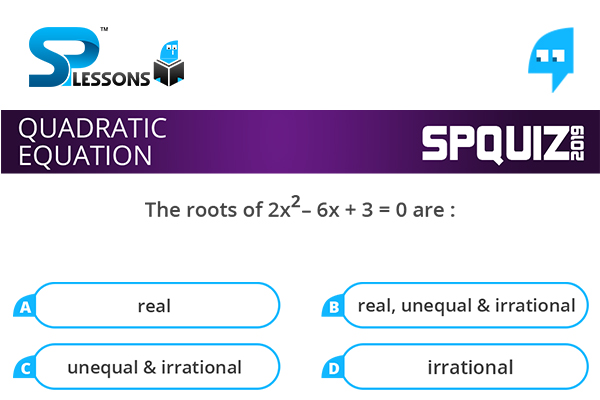
The roots of [latex]2 {x}^{2} - 6x + 3 = 0[/latex] are :
- A. real
B. real, unequal & irrational
C. unequal & irrational
D. irrational
D = [latex][{(6)}^{2} - 4 \times 2 \times 3] = (36 - 24) = 12[/latex]
Thus, D>0 and not a perfect square
i.e, Roots are real, unequal and irrational
 Q3
Q3
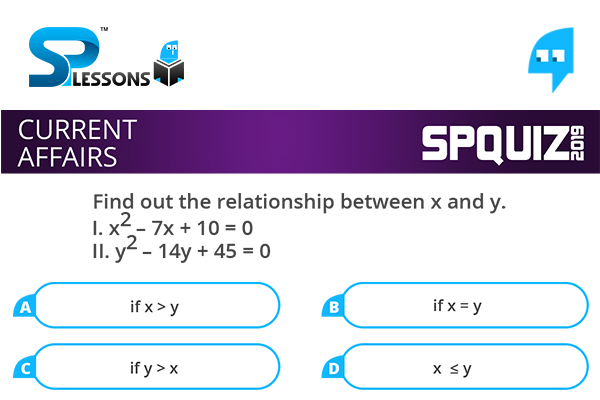
Find out the relationship between x and y.
I. [latex]{x}^{2} - 7x + 10 = 0[/latex]
II. [latex]{y}^{2} - 14y + 45 = 0[/latex]
- A. if x > y
B. if x = y
C. if y > x
D. x [latex]\leq[/latex] y
I. [latex]{x}^{2} - 7x + 10 = 0[/latex]
[latex]\Rightarrow {x}^{2} - 5x -2x + 10 = 0[/latex]
[latex]\Rightarrow x(x - 5) - 2(x - 5) = 0[/latex]
[latex]\Rightarrow x = 2, 5[/latex]
II. [latex]{y}^{2} - 14y + 45 = 0[/latex]
[latex]\Rightarrow {y}^{2} - 9y - 5y + 45 = 0[/latex]
[latex]\Rightarrow y(y -9) -5(y -9) = 0[/latex]
[latex]\Rightarrow y = 9, 5[/latex]
i.e, x [latex]\leq[/latex] y
 Q4
Q4
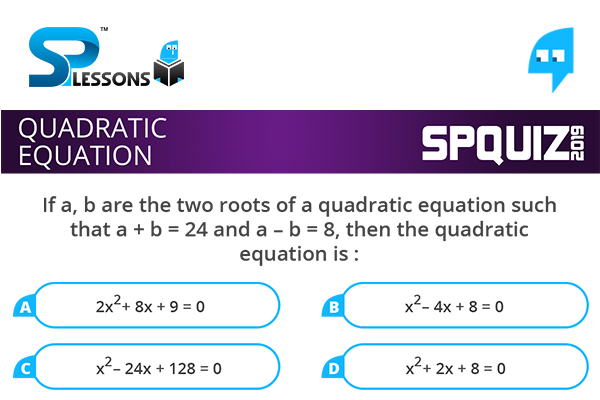
If a, b are the two roots of a quadratic equation such that a + b = 24 and a - b = 8, then the quadratic equation is :
- A. [latex] 2{x}^{2} + 8x + 9 = 0[/latex]
B. [latex] {x}^{2} - 4x + 8 = 0[/latex]
C. [latex] {x}^{2} - 24x + 128 = 0[/latex]
D. [latex] {x}^{2} + 2x + 8 = 0[/latex]
On solving a + b = 24 and a- b = 8, we get a = 16, b = 8
i.e, ab = 128
i.e, Required equation is [latex] {x}^{2} - (a + b)x + ab = 0 [/latex]
i.e, [latex] {x}^{2} - 24x + 128 = 0[/latex]
 Q5
Q5
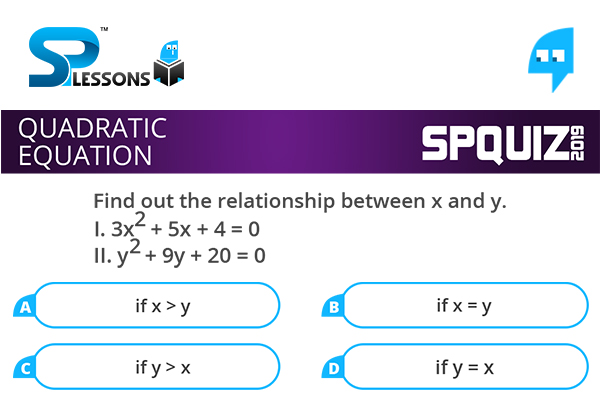
Find out the relationship between x and y.
I. [latex]3{x}^{2} + 5x + 4 = 0[/latex]
II. [latex]{y}^{2} + 9y + 20 = 0[/latex]
- A. if x > y
B. if x = y
C. if y > x
D. if y = x
I. [latex]3{x}^{2} + 5x + 4 = 0[/latex]
[latex]3{x}^{2} + 3x + 2x + 4 = 0[/latex]
[latex]\Rightarrow 3x(x + 1) + (x + 1) = 0[/latex]
[latex]\Rightarrow = -1. \frac{-2}{3}[/latex]
II. [latex]{y}^{2} + 9y + 20 = 0[/latex]
[latex]\Rightarrow y(y + 9) + 3(y + 9) = 0[/latex]
[latex]\Rightarrow = -3, -9[/latex]
i.e, x>y