Introduction
Introduction
Quadratic Equation is one of important topic in Quantitative Aptitude Section. In Quadratic Equation – Quiz 4 article candidates can find questions with an answer. By solving this questions candidates can improve and maintain, speed, and accuracy in the exams. Quadratic Equation – Quiz 4 questions are very useful for different exams such as IBPS PO, Clerk, SSC CGL, SBI PO, NIACL Assistant, NICL AO, IBPS SO, RRB, Railways, Civil Services etc.
 Q1
Q1
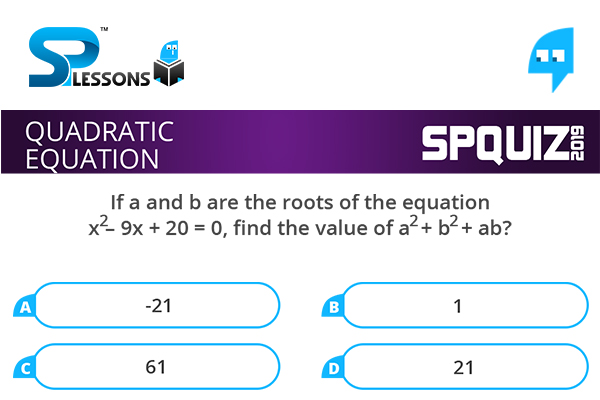
If a and b are the roots of the equation [latex]{x}^{2}[/latex] - 9x + 20 = 0, find the value of [latex]{a}^{2}[/latex] + [latex]{b}^{2}[/latex] + ab?
- A. -21
B. 1
C. 61
D. 21
[latex]{a}^{2}[/latex] + [latex]{b}^{2}[/latex] + ab = [latex]{a}^{2}[/latex] + [latex]{b}^{2}[/latex] + 2ab - ab
i.e, [latex]{(a + b)}^{2}[/latex] + ab
from [latex]{x}^{2}[/latex] - 9x + 20 = 0, we have
a + b = 9 and ab = 20. Hence the value of required expression [latex]{(9)}^{2}[/latex] - 20 = 61.
 Q2
Q2
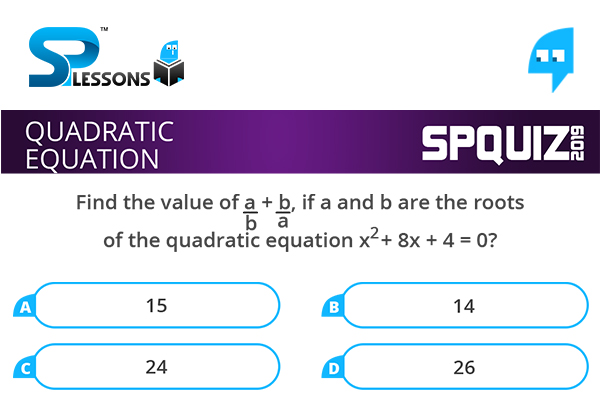
Find the value of [latex]\frac{a}{b}[/latex] + [latex]\frac{b}{a}[/latex], if a and b are the roots of the quadratic equation [latex]{x}^{2}[/latex] + 8x + 4 = 0?
- A. 15
B. 14
C. 24
D. 26
[latex]\frac{a}{b}[/latex] + [latex]\frac{b}{a}[/latex] = [latex]\frac{{a}^{2} + {b}^{2}}{ab}[/latex] + [latex]\frac{{a}^{2} + {b}^{2} + b + a}{ab}[/latex]
= [latex]\frac{({(a + b)}^{2} - 2ab)}{ab}[/latex]
a + b = [latex]\frac{-8}{1} = -8[/latex]
ab = [latex]\frac{4}{1}[/latex] = 4
Hence [latex]\frac{a}{b}[/latex] + [latex]\frac{b}{a}[/latex] = [latex]\frac{({(-8)}^{2} - 2(4))}{4}[/latex] = [latex]\frac{56}{4}[/latex] = 14
 Q3
Q3
A man could buy a certain number of notebooks for Rs.300. If each notebook cost is Rs.5 more, he could have bought 10 notebooks less for the same amount. Find the price of each notebook?
- A. 10
B. 8
C. 15
D. 7.5
Let the price of each note book be Rs.x.
Let the number of note books which can be brought for Rs.300 each at a price of Rs.x be y.
Hence xy = 300
[latex]\Rightarrow[/latex] y = [latex]\frac{300}{x}[/latex]
(x + 5)(y - 10) = 300 [latex]\Rightarrow[/latex] xy + 5y - 10x - 50 = xy
[latex]\Rightarrow[/latex] 5([latex]\frac{300}{x}[/latex]) - 10x - 50 = 0 [latex]\Rightarrow[/latex] - 150 + [latex]{x}^{2}[/latex] + 5x = 0
multiplying both sides by [latex]\frac{- 1}{10 x}[/latex]
[latex]\Rightarrow[/latex] [latex]{x}^{2}[/latex] + 15x - 10x - 150 = 0
[latex]\Rightarrow[/latex] x(x + 15) - 10(x + 15) = 0
[latex]\Rightarrow[/latex] x = 10 or -15
As x > 0, x = 10.
 Q4
Q4
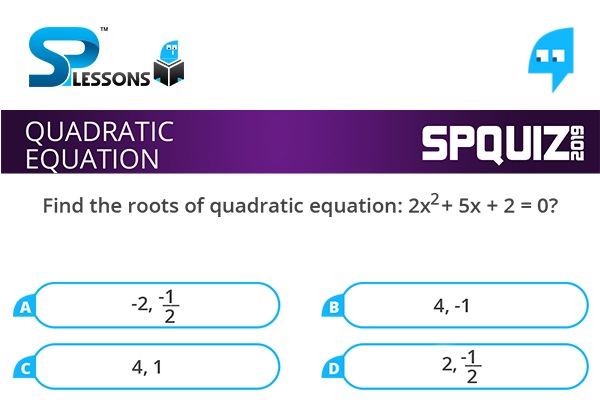
Find the roots of quadratic equation: 2[latex]{x}^{2}[/latex] + 5x + 2 = 0?
- A. -2, [latex]\frac{-1}{2}[/latex]
B. 4, -1
C. 4, 1
D. 2, [latex]\frac{-1}{2}[/latex]
2[latex]{x}^{2}[/latex] + 5x + 2 = 0
2x(x + 2) + 1(x + 2) = 0
(x + 2)(2x + 1) = 0 [latex]\Rightarrow[/latex] x = -2, [latex]\frac{-1}{2}[/latex]
 Q5
Q5
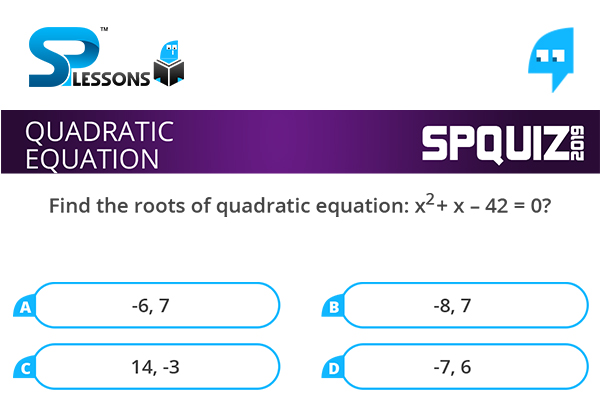
Find the roots of quadratic equation: [latex]{x}^{2}[/latex] + x - 42 = 0?
- A. -6, 7
B. -8, 7
C. 14, -3
D. -7, 6
[latex]{x}^{2}[/latex] + 7x - 6x + 42 = 0
x(x + 7) - 6(x + 7) = 0
(x + 7)(x - 6) = 0 [latex]\Rightarrow[/latex] x = -7, 6