Introduction
Introduction
Quadratic Equation is one of important topic in Quantitative Aptitude Section. In Quadratic Equation – Quiz 3 article candidates can find a question with an answer. By solving this question candidates can improve and maintain, speed, and accuracy in the exams. Quadratic Equation – Quiz 3 questions are very useful for different exams such as IBPS PO, Clerk, SSC CGL, SBI PO, NIACL Assistant, NICL AO, IBPS SO, RRB, Railways, Civil Services etc.
 Q1
Q1
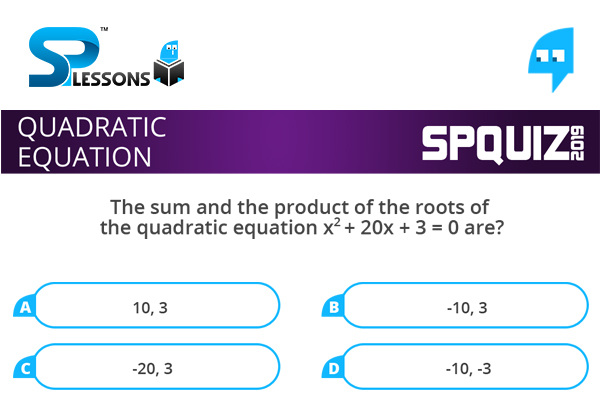
The sum and the product of the roots of the quadratic equation [latex]{x}^{2}[/latex] + 20x + 3 = 0 are?
- A. 10, 3
B. -10, 3
C. -20, 3
D. -10, -3
Sum of the roots and the product of the roots are -20 and 3 respectively.
 Q2
Q2
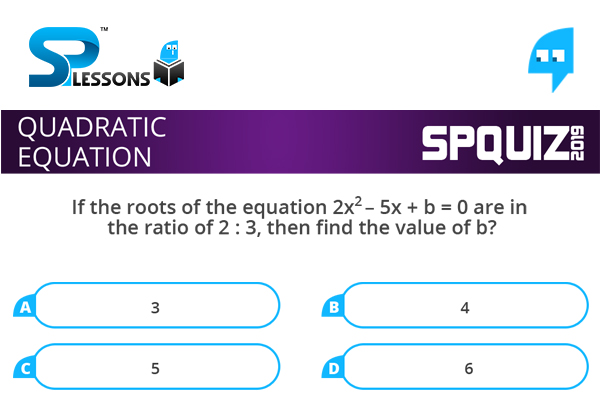
If the roots of the equation 2[latex]{x}^{2}[/latex] - 5x + b = 0 are in the ratio of 2 : 3, then find the value of b?
- A. 3
B. 4
C. 5
D. 6
Let the roots of the equation 2a and 3a respectively.
2a + 3a = 5a = -([latex]- \frac {5}{2}[/latex]) = [latex] \frac {5}{2} \Rightarrow [/latex] a = [latex] \frac {1}{2}[/latex]
Product of the roots: 6[latex]{a}^{2}[/latex] = [latex] \frac {b}{2} \Rightarrow [/latex] b = 12[latex]{a}^{2}[/latex]
a = [latex] \frac {1}{2}[/latex], b = 3.
 Q3
Q3
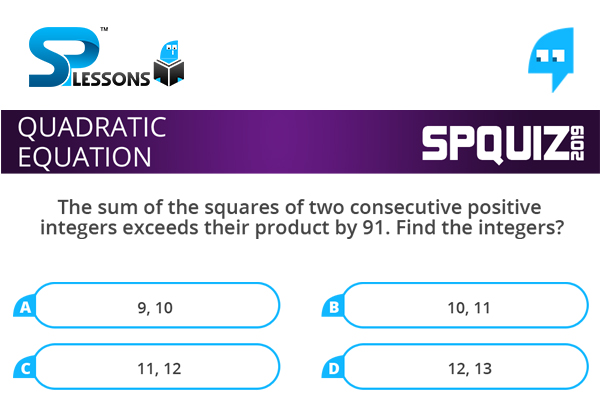
The sum of the squares of two consecutive positive integers exceeds their product by 91. Find the integers?
- A. 9, 10
B. 10, 11
C. 11, 12
D. 12, 13
Let the two consecutive positive integers be x and x + 1
[latex]{x}^{2}[/latex] + [latex]{(x + 1)}^{2}[/latex] - x(x + 1) = 91
[latex]{x}^{2}[/latex] + x - 90 = 0
(x + 10)(x - 9) = 0 [latex]\Rightarrow[/latex] x = -10 or 9.
As x is positive x = 9
Hence the two consecutive positive integers are 9 and 10.
 Q4
Q4
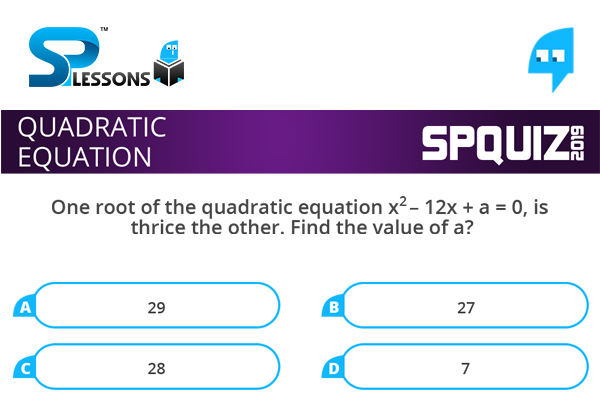
One root of the quadratic equation [latex]{x}^{2}[/latex] - 12x + a = 0, is thrice the other. Find the value of a?
- A. 29
B. 27
C. 28
D. 7
Let the roots of the quadratic equation be x and 3x.
Sum of roots = -(-12) = 12
a + 3a = 4a = 12 => a = 3
Product of the roots = 3[latex]{a}^{2}[/latex] = 3[latex]{(3)}^{2}[/latex] = 27.
 Q5
Q5
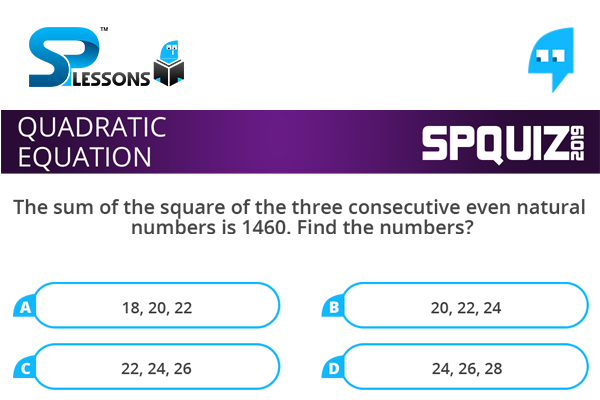
The sum of the square of the three consecutive even natural numbers is 1460. Find the numbers?
- A. 18, 20, 22
B. 20, 22, 24
C. 22, 24, 26
D. 24, 26, 28
Three consecutive even natural numbers be 2x - 2, 2x and 2x + 2.
[latex]{(2x - 2)}^{2}[/latex] + [latex]{(2x)}^{2}[/latex] + [latex]{(2x + 2)}^{2}[/latex] = 1460
[latex]4{x}^{2}[/latex] - 8x + 4 + [latex]4{x}^{2}[/latex] + 8x + 4 = 1460
[latex]12{x}^{2}[/latex] = 1452 [latex]\Rightarrow {x}^{2}[/latex] = 121 [latex]\Rightarrow[/latex] x = ± 11
As the numbers are positive, 2x > 0. Hence x > 0. Hence x = 11.
Required numbers are 20, 22, 24.