Introduction
Introduction
Probability is one of important topic in Quantitative Aptitude Section. In Probability – Quiz 7 article candidates can find questions with answer. By solving this questions candidates can improve and maintain, speed, and accuracy in the exams. Probability - Quiz 7 questions are very useful for different exams such as IBPS PO, Clerk, SSC CGL, SBI PO, NIACL Assistant, NICL AO, IBPS SO, RRB, Railways, Civil Services etc.
 Q1
Q1
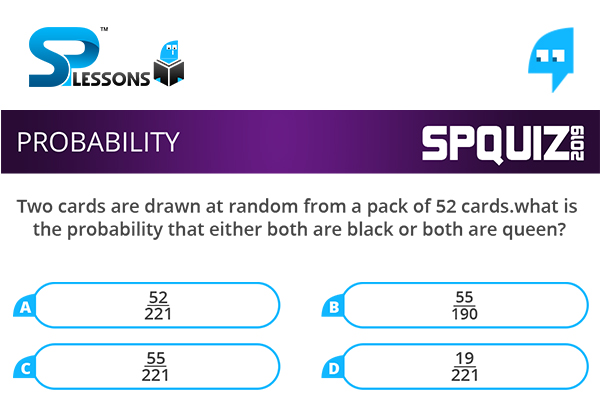
Two cards are drawn at random from a pack of 52 cards.what is the probability that either both are black or both are queen?
- A. [latex]\frac {52}{221}[/latex]
B. [latex]\frac {55}{190}[/latex]
C. [latex]\frac {55}{221}[/latex]
D. [latex]\frac {19}{221}[/latex]
We have n(s) = [latex]^{52}{C}_{2} 52 = 52 \times \frac {51}{2 \times 1} = 1326[/latex]
Let A = event of getting both black cards
B = event of getting both queens
A∩B = event of getting queen of black cards
n(A) = [latex]\frac {52 \times 51}{2 \times 1} = ^{26}{C}_{2} = 325, n(B)= \frac{26 \times 25 }{2 \times 1}= \frac {4 \times 3}{2 \times 1} = 6 and n(A∩B) = ^{4}{C}_{2} = 1 [/latex]
P(A) = [latex]\frac {n(A)}{n(S)} = \frac {325}{1326}[/latex]
P(B) = [latex]\frac {n(B)}{n(S)} = \frac {6}{1326}[/latex]
P(A∩B) = [latex]\frac {n(A∩B)}{n(S)} = \frac {1}{1326}[/latex]
P(A∪B) = P(A) + P(B) - P(A∩B) = [latex]\frac {(325 + 6 - 1)}{1326} = \frac {330}{1326} = \frac {55}{221}[/latex]
 Q2
Q2
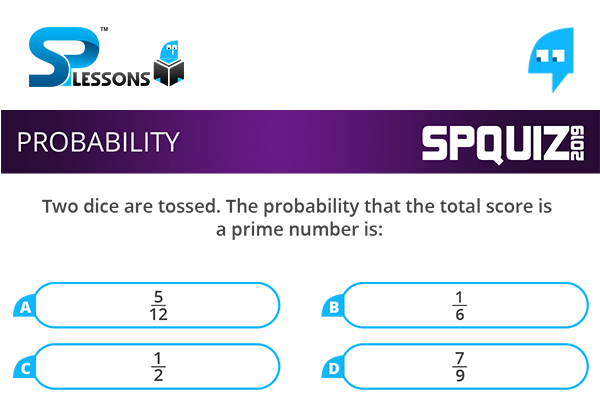
Two dice are tossed. The probability that the total score is a prime number is:
- A. [latex]\frac {5}{12}[/latex]
B. [latex]\frac {1}{6}[/latex]
C. [latex]\frac {1}{2}[/latex]
D. [latex]\frac {7}{9}[/latex]
Clearly, n(S) = (6 x 6) = 36.
Let E = Event that the sum is a prime number.
Then E= { (1, 1), (1, 2), (1, 4), (1, 6), (2, 1), (2, 3), (2, 5), (3, 2), (3, 4), (4, 1), (4,3),(5, 2), (5, 6), (6, 1), (6, 5) }
n(E) = 15.
P(E) = [latex]\frac {n(E)}{n(S)} = \frac {15}{36} = \frac {5}{12}[/latex]
 Q3
Q3
A bag contains 4 white, 5 red and 6 blue balls. Three balls are drawn at random from the bag. The probability that all of them are red, is:
- A. [latex]\frac {2}{91}[/latex]
B. [latex]\frac {1}{22}[/latex]
C. [latex]\frac {3}{22}[/latex]
D. [latex]\frac {2}{77}[/latex]
Let S be the sample space.
Then, n(S) = number of ways of drawing 3 balls out of 15 = [latex] ^{15}{C}_{3} = \frac {15 \times 14 \times 13}{3 \times 2 \times 1} = 455[/latex]
Let E = event of getting all the 3 red balls.
n(E) = [latex] ^{5}{C}_{3} = \frac {5 \times 4 }{3 \times 2 \times 1} = 10 [/latex]
=> P(E) = [latex]\frac {n(E)}{n(S)} \frac {10}{455} = \frac {2}{91}[/latex]
 Q4
Q4
In a lottery, there are 10 prizes and 25 blanks. A lottery is drawn at random. What is the probability of getting a prize?
- A. [latex]\frac {2}{7}[/latex]
B. [latex]\frac {5}{7}[/latex]
C. [latex]\frac {1}{5}[/latex]
D. [latex]\frac {1}{2}[/latex]
Total number of outcomes possible, n(S) = 10 + 25 = 35
Total number of prizes, n(E) = 10
P(E) = [latex]\frac {n(E)}{n(s)} = \frac {10}{35} = \frac {2}{7}[/latex]
 Q5
Q5
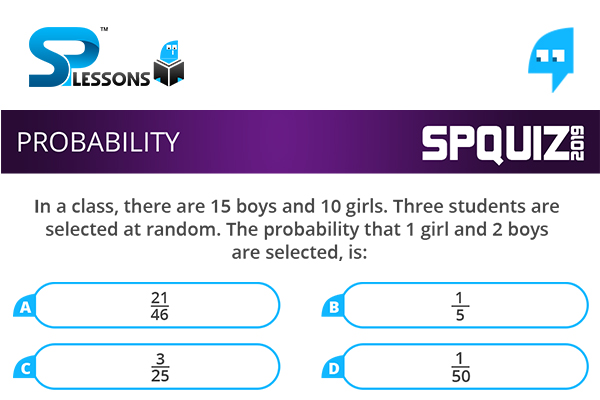
In a class, there are 15 boys and 10 girls. Three students are selected at random. The probability that 1 girl and 2 boys are selected, is:
- A. [latex]\frac {21}{46}[/latex]
B. [latex]\frac {1}{5}[/latex]
C. [latex]\frac {3}{25}[/latex]
D. [latex]\frac {1}{50}[/latex]
Let, S - sample space E - event of selecting 1 girl and 2 boys.
Then, n(S) = Number ways of selecting 3 students out of 25
= [latex]^{25}{C}_{3}[/latex]
= 2300.
n(E) = [latex]^{10}{C}_{1}[/latex] × [latex]^{15}{C}_{2}[/latex] = 1050.
P(E) = [latex]\frac {n(E)}{n(s)} = \frac {1050}{2300} = \frac {21}{46}[/latex]
 Study Guide
Study Guide
| Section Name | Name of the Article |
|---|---|
| Study-Guide | Quantitative Aptitude |
Reasoning Ability |
|
General Awareness |
|
Computer Awareness |
|
English Knowledge |
|
World of Words (WOW) |
|
Descriptive Test |
 Exams
Exams
| Category | Notification |
|---|---|
| Degree & PG | NPAT 2019 |
| Engineering | AIEEA PG 2019 |
| AIEEA UG 2019 | |
| AUEET 2019 | |
| VITMEE | |
| Professor | TS SET 2019 |
| Medical | FMGE 2019 |
 CA
CA
 SP Quiz
SP Quiz
| Category | Quiz |
|---|---|
| Quant Aptitude | |
| Permutation and Combination | |
| Speed and Time | |
| Mensuration | |
| Profit and Loss | |
| Reasoning Ability | Puzzles |
| Seating Arrangement | |
| Direction Sense | |
| General Awareness | General Awareness Quiz |
| Banking Awareness | Banking Awareness Quiz |
| Computer Awareness | Computer Awareness Quiz |