Introduction
Introduction
Probability is one of important topic in Quantitative Aptitude Section. In Probability – Quiz 4 article candidates can find questions with an answer. By solving this questions candidates can improve and maintain, speed, and accuracy in the exams. Probability - Quiz 4 questions are very useful for different exams such as IBPS PO, Clerk, SSC CGL, SBI PO, NIACL Assistant, NICL AO, IBPS SO, RRB, Railways, Civil Services etc.
 Q1
Q1
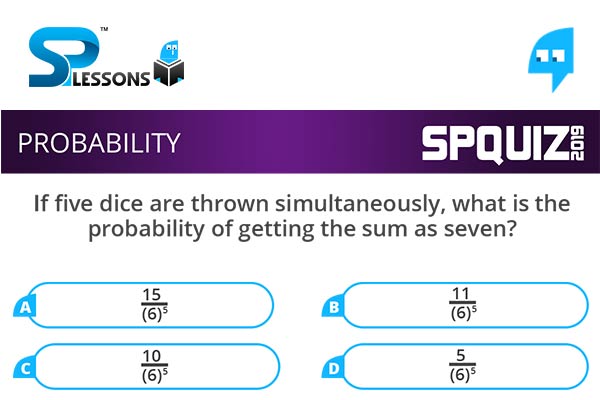
If five dice are thrown simultaneously, what is the probability of getting the sum as seven?
- A. [latex]\frac{15}{{(6)}^{5}}[/latex]
B. [latex]\frac{11}{{(6)}^{5}}[/latex]
C. [latex]\frac{10}{{(6)}^{5}}[/latex]
D. [latex]\frac{5}{{(6)}^{5}}[/latex]
The following are two cases when the sum will be 7:
7 = 1 + 1 + 1 + 1 + 3 ⇒ [latex]^{5}{C}_{1}[/latex] ways = 5
7 = 1 + 1 + 1 + 2 + 2 ⇒ [latex]^{5}{C}_{2}[/latex] ways = 10
Total number of possible ways of throwing five dice = [latex]{(6)}^{5}[/latex]
The required probability = [latex]\frac{15}{{(6)}^{5}}[/latex]
 Q2
Q2
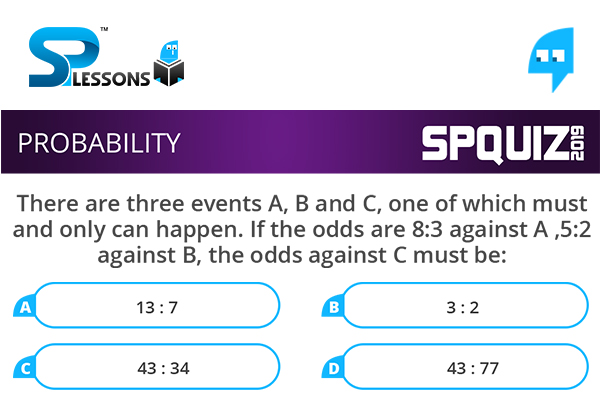
There are three events A, B and C, one of which must and only can happen. If the odds are 8:3 against A ,5:2 against B, the odds against C must be:
- A. 13 : 7
B. 3 : 2
C. 43 : 34
D. 43 : 77
According to the question,
[latex]\frac{P(A')}{P(A)}[/latex] = [latex]\frac{8}{3}[/latex], P(A) = [latex]\frac{3}{11}[/latex] and P(A') = [latex]\frac{8}{11}[/latex]
Also [latex]\frac{P(B')}{P(B)}[/latex] = [latex]\frac{8}{3}[/latex]
P(B) = [latex]\frac{2}{7}[/latex] and P(B') = [latex]\frac{8}{3}[/latex]
Now, out of A,B and C, one and only one can happen.
P(A) + P(B) + P(C) = 1
P(C) = [latex]\frac{34}{77}[/latex]
P(C') = 1 − P(C)
= [latex]\frac{43}{77}[/latex]
So odd against C
[latex]\frac{P(C)}{P(C')}[/latex] = [latex]\frac{43}{34}[/latex]
 Q3
Q3
If the probability that X will live 15 year is 7/8 and that Y will live 15 years is 9/10, then what is the probability that both will live after 15 years?
- A. [latex]\frac{1}{20}[/latex]
B. [latex]\frac{63}{80}[/latex]
C. [latex]\frac{1}{5}[/latex]
D. None of these
Required probability
[latex]\frac{7}{8} \times \frac{9}{10}[/latex]
[latex]\frac{63}{80}[/latex]
 Q4
Q4
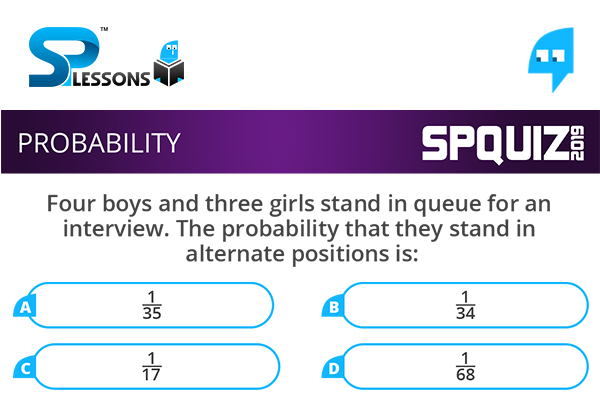
Four boys and three girls stand in queue for an interview. The probability that they stand in alternate positions is:
- A. [latex]\frac{1}{35}[/latex]
B. [latex]\frac{1}{34}[/latex]
C. [latex]\frac{1}{17}[/latex]
D. [latex]\frac{1}{68}[/latex]
Total number of possible arrangements for 4 boys and 3 girls in a queue = 7!
When they occupy alternate position the arrangement would be like:
B G B G B G B
Thus, total number of possible arrangements for boys = (4× 3 × 2)
Total number of possible arrangements for girls = (3 × 2)
Required probability
= [latex]\frac{4 \times 3 \times 2 \times 3 \times \times 2}{7!}[/latex]
= [latex]\frac{1}{35}[/latex]
 Q5
Q5
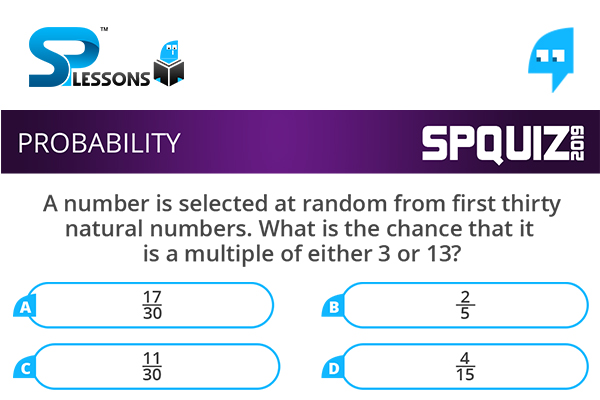
A number is selected at random from first thirty natural numbers. What is the chance that it is a multiple of either 3 or 13?
- A. [latex]\frac{17}{30}[/latex]
B. [latex]\frac{2}{5}[/latex]
C. [latex]\frac{11}{30}[/latex]
D. [latex]\frac{4}{15}[/latex]
We know that,
Probability = [latex]\frac{Favorable Cases}{Total Cases}[/latex]
The probability that the number is a multiple of 3 is [latex]\frac{10}{30}[/latex].
Since favorable cases here {3, 6, 9, 12, 15, 18, 21, 24, 27, 30}
= 10 cases
Total cases = 30 cases
Similarly the probability that the number is a multiple of 13 is [latex]\frac{2}{30}[/latex].
Since favorable cases here {13, 26}
= 2 cases
Total cases = 30 cases
Neither 3 nor 13 has common multiple from 1 to 30.
Hence, these events are mutually exclusive events.
Therefore chance that the selected number is a multiple of 3 or 13 is:
= [latex]\frac{10 + 2}{30}[/latex]
= [latex]\frac{2}{5}[/latex]