Introduction
Introduction
Probability is one of important topic in Quantitative Aptitude Section. In Probability – Quiz 3 article candidates can find questions with an answer. By solving this questions candidates can improve and maintain, speed, and accuracy in the exams. Probability - Quiz 3 questions are very useful for different exams such as IBPS PO, Clerk, SSC CGL, SBI PO, NIACL Assistant, NICL AO, IBPS SO, RRB, Railways, Civil Services etc.
 Q1
Q1
There are 2 positive integers x and y. What is the probability that x + y is odd?
- A. 4
B. 10
C. [latex]\frac{1}{2}[/latex]
D. 36
Here we can have four cases:
a). x is even, y is even
b). x is odd, y is even
c). x is even, y is odd
d). x is odd, y is odd
Out of these four cases, in case 'b' and 'c' the sum will be odd.
So required probability = [latex]\frac{2}{4}[/latex] = [latex]\frac{1}{2}[/latex]
 Q2
Q2
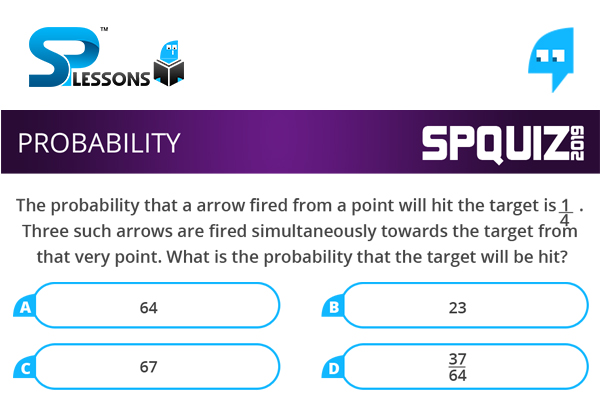
The probability that a arrow fired from a point will hit the target is [latex]\frac{1}{4}[/latex]. Three such arrows are fired simultaneously towards the target from that very point. What is the probability that the target will be hit?
- A. 64
B. 23
C. 67
D. [latex]\frac{37}{64}[/latex]
Probability of a arrow not hitting the target = [latex]\frac{3}{4}[/latex]
Probability that none of the 3 arrow will hit the target: = [latex]{(\frac{3}{4})}^{3}[/latex]
= [latex]\frac{27}{64}[/latex]
Probability that the target will hit at least once:
= 1 - [latex]\frac{27}{64}[/latex]
= [latex]\frac{37}{64}[/latex]
 Q3
Q3
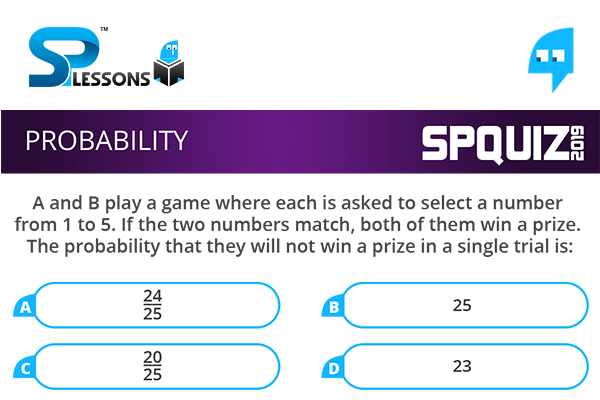
A and B play a game where each is asked to select a number from 1 to 5. If the two numbers match, both of them win a prize. The probability that they will not win a prize in a single trial is:
- A. [latex]\frac{24}{25}[/latex]
B. 25
C. [latex]\frac{20}{25}[/latex]
D. 23
Total number of ways in which both of them can select a number each:
=5 × 5
=25
Total number of ways in which both of them can select a same number so that they both can win:
= 5 ways [They both can select {(1,1), (2,2), (3,3), (4,4), (5,5)}]
Probability that they win the prize:
= [latex]\frac {Favorable Cases}{Total Cases}[/latex]
= [latex]\frac{5}{25}[/latex]
Probability that they do not win a prize:
= 1 − [latex]\frac{5}{25}[/latex]
= [latex]\frac{20}{25}[/latex]
 Q4
Q4
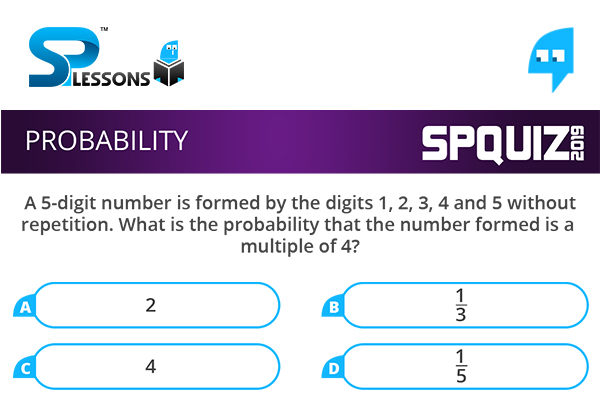
A 5-digit number is formed by the digits 1, 2, 3, 4 and 5 without repetition. What is the probability that the number formed is a multiple of 4?
- A. 2
B. [latex]\frac{1}{3}[/latex]
C. 4
D. [latex]\frac{1}{5}[/latex]
Total number of 5 digits number = 5! = 120.
Now to be multiply of 4, the last 2 digits of the number have to be divisible by 4. i.e. they must be 12, 24, 32 or 52.
Corresponding to each of these ways there are 3! = 6, i.e. 6 ways of filling the remaining 3 places.
The required probability
= [latex]\frac{4 \times 6}{120}[/latex]
= [latex]\frac{1}{5}[/latex]
 Q5
Q5
A box contains 9 red toys, 7 green toys and 9 blue toys. Each ball is of a different size. The probability that the red ball being selected is the smallest red toy, is:
- A. [latex]\frac{1}{9}[/latex]
B. [latex]\frac{2}{21}[/latex]
C. [latex]\frac{1}{25}[/latex]
D. [latex]\frac{6}{25}[/latex]
Since, there are 9 red toys and all of them are of different sizes, the probability of choosing the smallest among them is [latex]\frac{1}{9}[/latex]