Introduction
Introduction
Probability is one of important topic in Quantitative Aptitude Section. In Probability – Quiz 2 article candidates can find a question with an answer. By solving this question candidates can improve and maintain, speed, and accuracy in the exams. Probability - Quiz 2 questions are very useful for different exams such as IBPS PO, Clerk, SSC CGL, SBI PO, NIACL Assistant, NICL AO, IBPS SO, RRB, Railways, Civil Services etc.
 Q1
Q1
The letters B, G, I, N and R are rearranged to form the word 'Bring'. Find its probability:
- A. [latex]\frac{1}{120}[/latex]
B. [latex]\frac{1}{40}[/latex]
C. [latex]\frac{1}{24}[/latex]
D. [latex]\frac{1}{76}[/latex]
There are total 5 letters. The probability that B gets the first position is [latex]\frac{1}{5}[/latex]
The probability that G is in the second position is [latex]\frac{1}{4}[/latex]
Likewise, probability for I, N and G
Hence required probability:
= [latex]\frac{1}{5}\times \frac{1}{4} \times \frac{1}{3} \times \frac{1}{2} \times 1[/latex]
= [latex]\frac{1}{120}[/latex]
 Q2
Q2
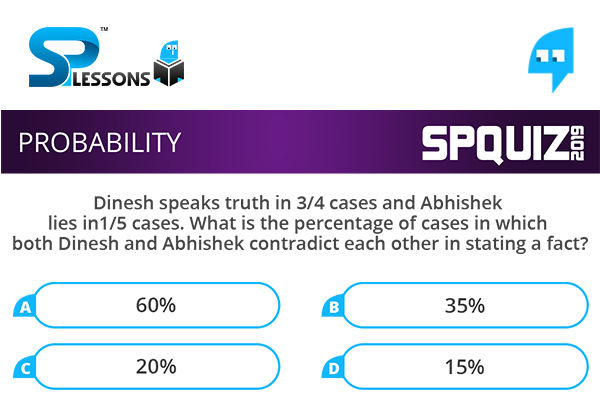
Dinesh speaks truth in 3/4 cases and Abhishek lies in 1/5 cases. What is the percentage of cases in which both Dinesh and Abhishek contradict each other in stating a fact?
- A. 60%
B. 35%
C. 20%
D. 15%
D and A will contradict each other when one speaks truth and other speaks lies.
Probability of D speak truth and A lies
= [latex]\frac{3}{4} \times \frac{1}{5}[/latex]
[latex]\frac{3}{20}[/latex]
Probability of A speak truth and D lies
= [latex]\frac{4}{5} \times \frac{1}{4}[/latex]
= [latex] \frac{1}{5}[/latex]
The two probabilities are mutually exclusive.
Hence, probabilities that D and A contradict each other:
= [latex]\frac{3}{20} + \frac{1}{5}[/latex]
= [latex]\frac{7}{20}[/latex]
= 0.35 [latex]\times[/latex] 100 %
= 35 %
 Q3
Q3
A set of cards bearing the number 200-299 is used in a game. if a card is drawn at random, what is the probability that it is divisible by 3:
- A. 0.66
B. 0.33
C. 0.44
D. 0.55
Total numbers of cards = 100 {and not 99}
Multiples of three = 33
The required probability,
= [latex]\frac{33}{100}[/latex]
= 0.33
 Q4
Q4
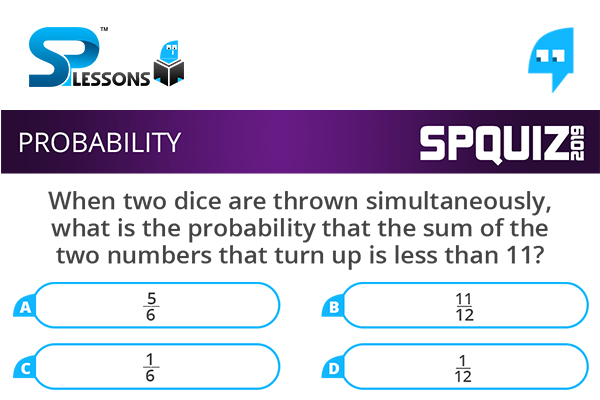
When two dice are thrown simultaneously, what is the probability that the sum of the two numbers that turn up is less than 11?
- A. [latex]\frac{5}{6}[/latex]
B. [latex]\frac{11}{12}[/latex]
C. [latex]\frac{1}{6}[/latex]
D. [latex]\frac{1}{12}[/latex]
Instead of finding the probability of this event directly, we will find the probability of the non-occurrence of this event and subtract it from 1 to get the required probability.
Combination whose sum of 12 is (6,6)
Combinations whose sum of 11 is (5,6), (6,5).
Therefore, there are totally 3 occurrences out of 36 occurrences that satisfy the given condition.
Probability whose sum of two numbers is greater than or equal to 11:
= [latex]\frac{3}{36} = \frac{1}{12}[/latex]
Hence probability whose sum of two numbers is lesser than 11:
= 1 - [latex] \frac{1}{12}[/latex]
= [latex] \frac{11}{12}[/latex]
 Q5
Q5
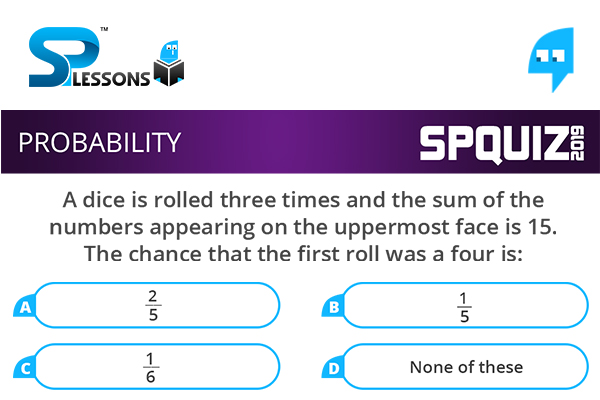
A dice is rolled three times and the sum of the numbers appearing on the uppermost face is 15. The chance that the first roll was a four is:
- A. [latex] \frac{2}{5}[/latex]
B. [latex] \frac{1}{5}[/latex]
C. [latex] \frac{1}{6}[/latex]
D. None of these
The sum of numbers can be 15 in the following three ways :
Case I: 15 = 3 + 6 + 6
The first, second and third throws can be (3, 6, 6), (6, 3, 6) and (6, 6, 3) respectively.
Total number of ways in which 3,6 and 6 can be obtained = 3
Case II: 15 = 4 + 5 + 6
The first, second and third throws can be 4, 5 and 6.
Total number of ways in which 4,5 and 6 can be obtained = 6
Case III: 15 = 5 + 5 + 5
The first, second and third throws can be 5, 5 and 5.
Total number of ways in which 5,5, and 5 can be obtained = 1.
Hence, The total number of ways = 3 + 6 + 1 = 10
The total number of ways in which the first roll will be 4 is 2.
Required chance =[latex] \frac{2}{10}[/latex] = [latex] \frac{1}{5}[/latex]