SP Quiz - SPLessons
boy2

Probability Quiz 12

Home > > Tutorial
SPLessons 5 Steps, 3 Clicks
5 Steps - 3 Clicks

Probability Quiz 12

shape Introduction

Probability is one of important topic in Quantitative Aptitude Section. In Probability Quiz 12 article candidates can find questions with answer. By solving this questions candidates can improve and maintain, speed, and accuracy in the exams. Probability Quiz 12 questions are very useful for different exams such as IBPS PO, Clerk, SSC CGL, SBI PO, NIACL Assistant, NICL AO, IBPS SO, RRB, Railways, Civil Services etc.

shape Q1

Three unbiased coins are tossed. What is the probability of getting at least 2 tails?
    A. 0.75 B. 0.5 C. 0.25 D. 0.2

SPLessons

S = {HHH, HHT, HTH, HTT, THH, THT, TTH, TTT}
E = {HTT, THT, TTH, TTT}
n(S) = 8
n(E) = 4
P(E) = [latex]\frac {n(E)}{n(S)} = \frac {4}{8} = 0.5[/latex]

shape Q2

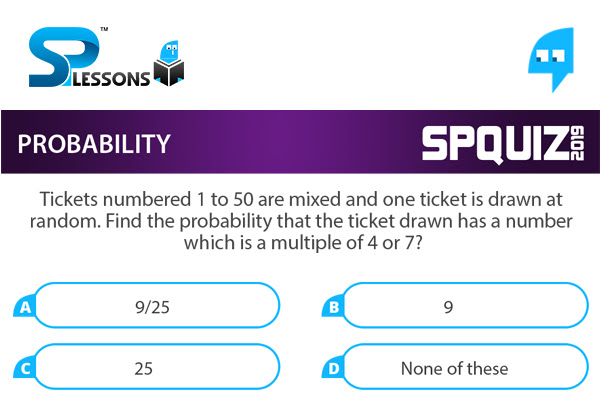
Tickets numbered 1 to 50 are mixed and one ticket is drawn at random. Find the probability that the ticket drawn has a number which is a multiple of 4 or 7?
    A. 9/25 B. 9 C. 25 D. None of these

SPLessons

S = {1, 2, 3, … , 49, 50}
E = {4, 8, 12, 16, 20, 24, 28, 32, 36, 40, 44, 48, 7, 14, 21, 35, 42, 49}
n(S) = 50
n(E) = 18
P(E) = [latex] \frac {n(E)}{n(S)} = \frac {18}{50}[/latex]
= [latex] \frac {9}{25}[/latex]

shape Q3

From a pack of 52 cards, one card is drawn at random. Find the probability that the drawn card is a club or a jack?
    A. 17 B. 16 C. 4/13 D. 15

SPLessons

n(S) = 52
n(E) = 16
P(E) = [latex] \frac {n(E)}{n(S)} = \frac {16}{52}[/latex]
= [latex]\frac {4}{13}[/latex]

shape Q4

Two friends A and B apply for a job in the same company. The chances of A getting selected is 2/5 and that of B is 4/7. What is the probability that both of them get selected?
    A. 8/35 B. 34 C. 27 D. 35

SPLessons

P(A) = [latex] \frac {2}{5}[/latex]
P(B) = [latex] \frac {4}{7}[/latex]
E = {A and B both get selected}
[latex]P(E) = P(A) \times P(B)[/latex]
= [latex] \frac {2}{5} \times \frac {4}{7} [/latex]
= [latex] \frac {8}{35}[/latex]

shape Q5

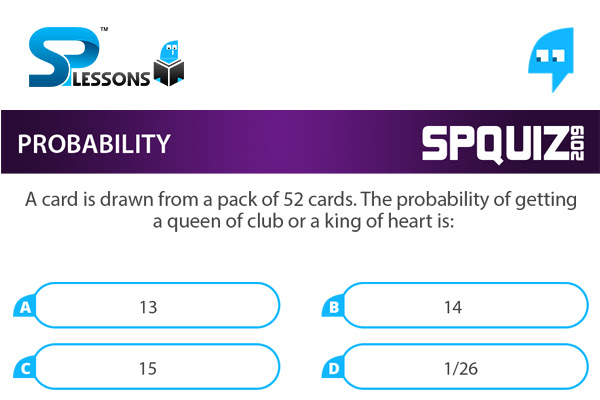
A card is drawn from a pack of 52 cards. The probability of getting a queen of club or a king of heart is:
    A. 13 B. 14 C. 15 D. 1/26

SPLessons

Here, n(S) = 52.
Let E = event of getting a queen of club or a king of heart.
Then, n(E) = 2.
i.e, P(E) = [latex]\frac {n(E)}{n(s)} = \frac {2}{52} = \frac {1}{26}[/latex]
Hide Index Show Index