Introduction
Introduction
Probability is one of important topic in Quantitative Aptitude Section. In Probability – Quiz 1 article candidates can find questions with answers. By solving this question candidates can improve and maintain, speed, and accuracy in the exams. Probability - Quiz 1 questions are very useful for different exams such as IBPS PO, Clerk, SSC CGL, SBI PO, NIACL Assistant, NICL AO, IBPS SO, RRB, Railways, Civil Services etc.
 Q1
Q1
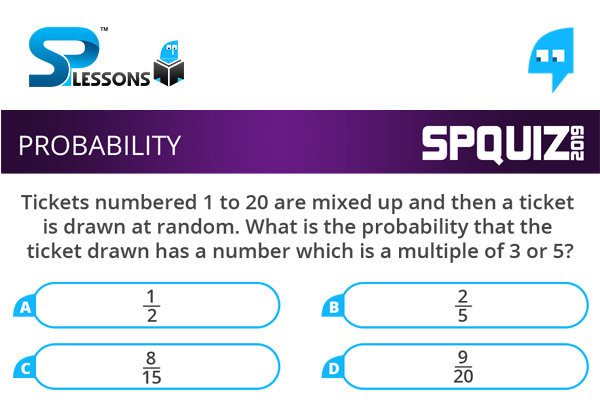
Tickets numbered 1 to 20 are mixed up and then a ticket is drawn at random. What is the probability that the ticket drawn has a number which is a multiple of 3 or 5?
- A. [latex]\frac {1}{2}[/latex]
B. [latex]\frac {2}{5}[/latex]
C. [latex]\frac {8}{15}[/latex]
D. [latex]\frac {9}{20}[/latex]
Here, S = {1, 2, 3, 4, ...., 19, 20}.
Let E = event of getting a multiple of 3 or 5 = {3, 6 , 9, 12, 15, 18, 5, 10, 20}.
i.e, P(E) = [latex]\frac {n(E)}{n(s)}[/latex] = [latex]\frac {9}{20}[/latex]
 Q2
Q2
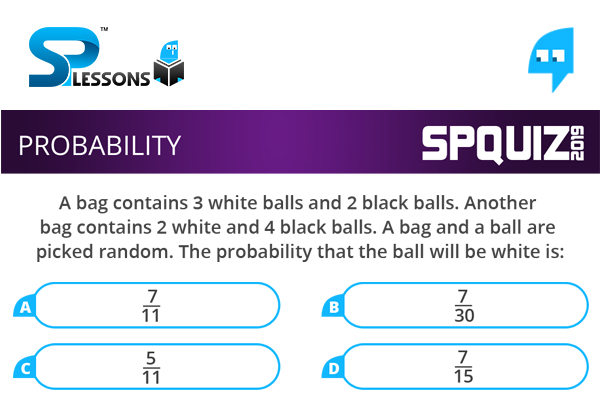
A bag contains 3 white balls and 2 black balls. Another bag contains 2 white and 4 black balls. A bag and a ball are picked random. The probability that the ball will be white is:
- A. [latex]\frac {7}{11}[/latex]
B. [latex]\frac {7}{30}[/latex]
C. [latex]\frac {5}{11}[/latex]
D. [latex]\frac {7}{15}[/latex]
The probability of selecting one bag = [latex]\frac {1}{2}[/latex]
Now, probability of getting a white ball from bag A
= [latex]\frac {1}{2}\times[/latex] [latex]\frac {3}{5}[/latex] = [latex]\frac {3}{10}[/latex]
And probability of getting a white ball from bag B
= [latex]\frac {1}{2}\times[/latex] [latex]\frac {2}{6}[/latex] = [latex]\frac {1}{6}[/latex]
Hence, Probability that white ball is drawn either first or second bag
= [latex]\frac {3}{10}[/latex] + [latex]\frac {1}{6}[/latex] = [latex]\frac {7}{15}[/latex]
 Q3
Q3
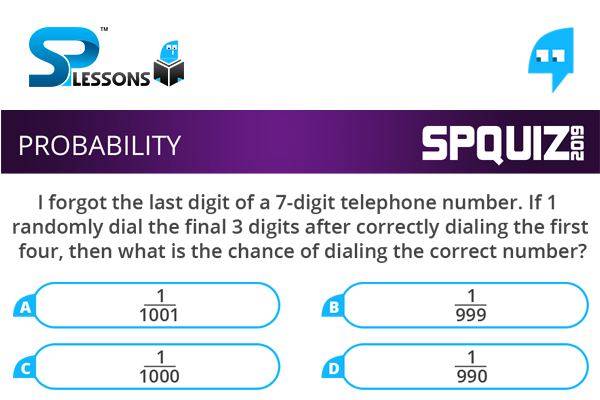
I forgot the last digit of a 7-digit telephone number. If 1 randomly dial the final 3 digits after correctly dialing the first four, then what is the chance of dialing the correct number?
- A. [latex]\frac {1}{1001}[/latex]
B. [latex]\frac {1}{999}[/latex]
C. [latex]\frac {1}{1000}[/latex]
D. [latex]\frac {1}{990}[/latex]
It is given that last three digits are randomly dialed. then each of the digit can be selected out of 10 digits in 10 ways.
Hence required probability
= [latex]{(\frac {1}{10})}^{3}[/latex]
= [latex]\frac {1}{1000}[/latex]
 Q4
Q4
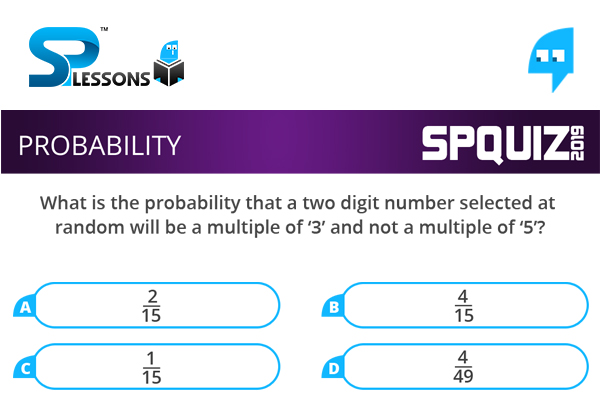
What is the probability that a two digit number selected at random will be a multiple of '3' and not a multiple of '5'?
- A. [latex]\frac {2}{15}[/latex]
B. [latex]\frac {4}{15}[/latex]
C. [latex]\frac {1}{15}[/latex]
D. [latex]\frac {4}{49}[/latex]
There are a total of 90 two digit numbers. Every third number will be divisible by '3'. Therefore, there are 30 of those numbers that are divisible by '3'.
Of these 30 numbers, the numbers that are divisible by '5' are those that are multiples of '15'. i.e. numbers that are divisible by both '3' and '5'.
There are 6 such numbers -- 15, 30, 45, 60, 75 and 90.
We need to find out numbers that are divisible by '3' and not by '5', which will be:
30 − 6 = 24.
24 out of the 90 numbers are divisible by '3' and not by '5'.
The required probability is therefore,
= [latex]\frac {24}{90}[/latex]
= [latex]\frac {4}{15}[/latex]
 Q5
Q5
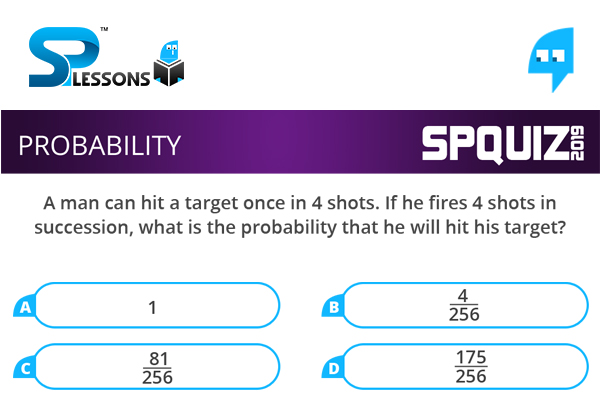
A man can hit a target once in 4 shots. If he fires 4 shots in succession, what is the probability that he will hit his target?
- A. 1
B. [latex]\frac {1}{256}[/latex]
C. [latex]\frac {81}{256}[/latex]
D. [latex]\frac {175}{256}[/latex]
The man will hit the target even if he hits it once or twice or thrice or all four times in the four shots that he takes.
So, the only case where the man will not hit the target is when he fails to hit the target even in one of the four shots that he takes.
The probability that he will not hit the target in one shot =1 - Probability that he will hit target in exact one shot = 1 - [latex]\frac {1}{4}[/latex]
= [latex]\frac {3}{4}[/latex]
Therefore, the probability that he will not hit the target in all the four shots
= [latex]\frac {3}{4} \times[/latex] [latex]\frac {3}{4} \times[/latex] [latex]\frac {3}{4} \times[/latex] [latex]\frac {3}{4} [/latex]
= [latex]\frac {81}{256}[/latex]
Hence, the probability that he will hit the target at least in one of the four shots:
= (1 - [latex]\frac {81}{256}[/latex])
= [latex]\frac {175}{256}[/latex]