Introduction
Permutation and Combination is one of important topic in Quantitative Aptitude Section. In Permutation and Combination Quiz 9 article candidates can find questions with answer. By solving this questions candidates can improve and maintain, speed, and accuracy in the exams. Permutation and Combination Quiz 9 questions are very useful for different exams such as IBPS PO, Clerk, SSC CGL, SBI PO, NIACL Assistant, NICL AO, IBPS SO, RRB, Railways, Civil Services etc.
Q1
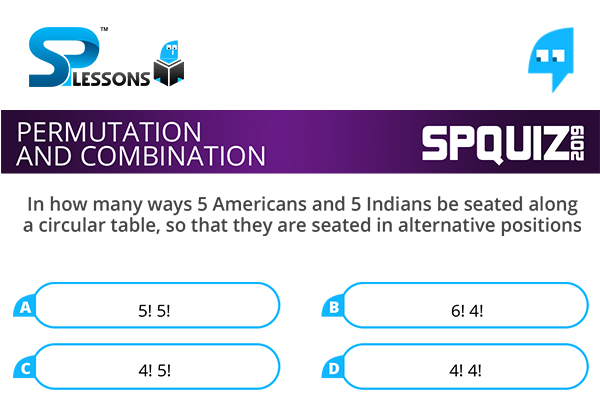
In how many ways 5 Americans and 5 Indians be seated along a circular table, so that they are seated in alternative positions
- A. 5! 5!
B. 6! 4!
C. 4! 5!
D. 4! 4!
First Indians can be seated along the circular table in 4! Ways and now Americans can be seated in 5! Ways. So 4! 5! Ways
Q2
4 matches are to be played in a chess tournament. In how many ways can result be decided?
- A. 27
B. 9
C. 81
D. 243
Every chess match can have three result i.e. win, loss and draw
so now of ways = 3 × 3 × 3 × 3 = 81 ways
Q3
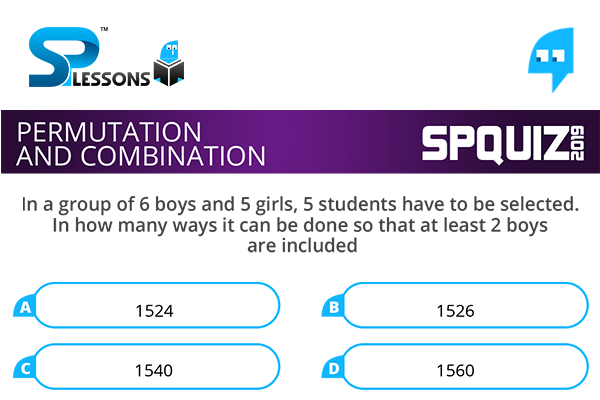
In a group of 6 boys and 5 girls, 5 students have to be selected. In how many ways it can be done so that at least 2 boys are included
- A. 1524
B. 1526
C. 1540
D. 1560
[latex]^{6}{C}_{2} \times ^{5}{C}_{3} + ^{6}{C}_{3} \times ^{5}{C}_{2} + ^{6}{C}_{4} \times ^{5}{C}_{1} + ^{6}{C}_{5}[/latex] = 1526
Q4
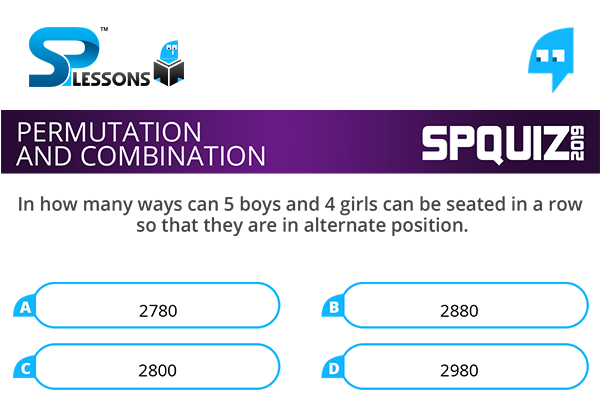
In how many ways can 5 boys and 4 girls can be seated in a row so that they are in alternate position.
- A. 2780
B. 2880
C. 2800
D. 2980
First boys are seated in 5 position in 5! Ways, now remaining 4 places can be filled by 4 girls in 4! Ways, so number of ways = 5! 4! = 2880
Q5
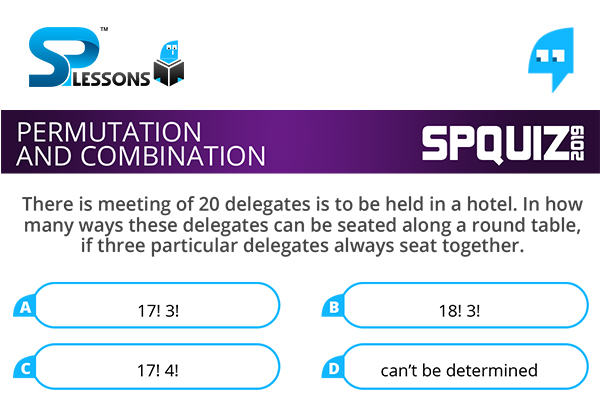
There is meeting of 20 delegates is to be held in a hotel. In how many ways these delegates can be seated along a round table, if three particular delegates always seat together.
- A. 17! 3!
B. 18! 3!
C. 17! 4!
D. can’t be determined
Total 20 persons, 3 always seat together, 17 + 1 =18 delegates can be seated in (18 - 1)! Ways = 17!
And now that three can be arranged in 3! Ways. So, 17! 3!
Other Articles
Study Guide
Exams
| Competitive Exams - College Entrance Exams | |||
|---|---|---|---|
| Category | Notification | ||
| Diploma | NITC New Delhi | Goa Diploma Admissions 2019 | |
| Click Here For – All India Entrance Exam Notifications | |||
Daily CA
Job-Alerts
SP Quiz
| Competitive Exams - Practice Sets | |
|---|---|
| Category | Quiz |
| Quant Aptitude | Probability |
| Speed and Time | |
| Reasoning Ability | Puzzles |
| English Language | Spotting Errors |
GK
| General Knowledge for Competitive Examinations | |
|---|---|
| Topic | Name of the Article |
| GK - World | Earth Mountain Ranges |
| National Emblems | |
| GK - India | Indian Airports |
| Indian National Parks | |
| GK - Abbreviations | International Organizations Abbreviations |
| Science Information Technology Abbreviations | |
| GK - Banking & Insurance | Stock Broking – SEBI Entities |
| Basel Norms – Banking System | |
| GK - Science & Technology | Vitamins and Food Sources |
| Zoology Terminology | |
| IBM IIT Bombay AI Research | |