Introduction
Introduction
Permutation and Combination is one of important topic in Quantitative Aptitude Section. In Permutation and Combination – Quiz 7 article candidates can find questions with answer. By solving this questions candidates can improve and maintain, speed, and accuracy in the exams. Permutation and Combination - Quiz 7 questions are very useful for different exams such as IBPS PO, Clerk, SSC CGL, SBI PO, NIACL Assistant, NICL AO, IBPS SO, RRB, Railways, Civil Services etc.
 Q1
Q1
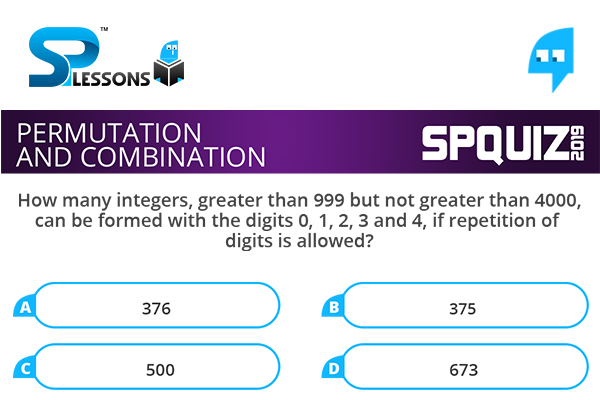
How many integers, greater than 999 but not greater than 4000, can be formed with the digits 0, 1, 2, 3 and 4, if repetition of digits is allowed?
- A. 376
B. 375
C. 500
D. 673
The smallest number in the series is 1000, a 4-digit number.
The largest number in the series is 4000, the only 4-digit number to start with 4.
The left most digit (thousands place) of each of the 4 digit numbers other than 4000 can take one of the 3 values 1 or 2 or 3.
The next 3 digits (hundreds, tens and units place) can take any of the 5 values 0 or 1 or 2 or 3 or 4.
Hence, there are 3 x 5 x 5 x 5 or 375 numbers from 1000 to 3999.
Including 4000, there will be 376 such numbers.
 Q2
Q2
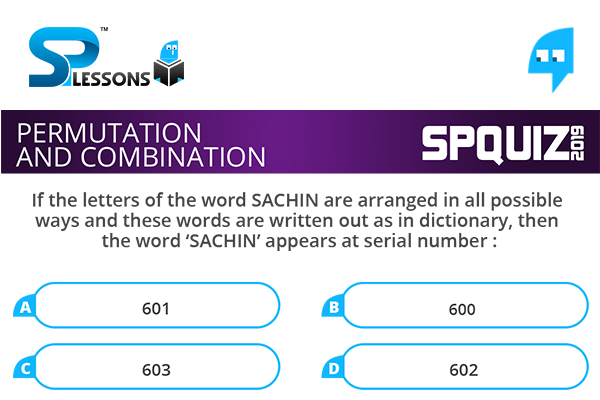
If the letters of the word SACHIN are arranged in all possible ways and these words are written out as in dictionary, then the word ‘SACHIN’ appears at serial number :
- A. 601
B. 600
C. 603
D. 602
If the word started with the letter A then the remaining 5 positions can be filled in 5! Ways.
If it started with c then the remaining 5 positions can be filled in 5! Ways.Similarly if it started with H,I,N the remaining 5 positions can be filled in 5! Ways.
If it started with S then the remaining position can be filled with A,C,H,I,N in alphabetical order as on dictionary.
The required word SACHIN can be obtained after the 5 X 5! = 600 Ways i.e. SACHIN is the [latex]{601}^{th}[/latex] letter.
 Q3
Q3
12 people at a party shake hands once with everyone else in the room. How many handshakes took place?
- A. 72
B. 66
C. 76
D. 64
There are 12 people, so this is our n value.
So, [latex]^{12}{C}_{2}[/latex]= 66
 Q4
Q4
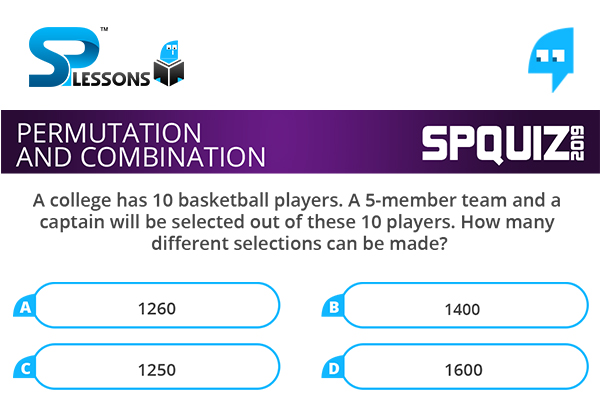
A college has 10 basketball players. A 5-member team and a captain will be selected out of these 10 players. How many different selections can be made?
- A. 1260
B. 1400
C. 1250
D. 1600
A team of 6 members has to be selected from the 10 players. This can be done in [latex]^{10}{C}_{6}[/latex] or 210 ways.
Now, the captain can be selected from these 6 players in 6 ways.
Therefore, total ways the selection can be made is 210 × 6 = 1260
 Q5
Q5
How many arrangements can be made out of the letters of the word COMMITTEE, taken all at a time, such that the four vowels do not come together?
- A. 216
B. 45360
C. 1260
D. 43200
There are total 9 letters in the word COMMITTEE in which there are 2M's, 2T's, 2E's.
The number of ways in which 9 letters can be arranged = [latex]\frac {9!}{2! × 2! × 2!}[/latex] = 45360
There are 4 vowels O,I,E,E in the given word. If the four vowels always come together, taking them as one letter we have to arrange 5 + 1 = 6 letters which include 2Ms and 2Ts and this be done in [latex]\frac {6!}{2!× 2!}[/latex] = 180 ways.
In which of 180 ways, the 4 vowels O,I,E,E remaining together can be arranged in [latex]\frac {4!}{2!}[/latex] = 12 ways.
The number of ways in which the four vowels always come together = 180 x 12 = 2160.
Hence, the required number of ways in which the four vowels do not come together = 45360 - 2160 = 43200
 Study Guide
Study Guide
| Section Name | Name of the Article |
|---|---|
| Study-Guide | Quantitative Aptitude |
Reasoning Ability |
|
General Awareness |
|
Computer Awareness |
|
English Knowledge |
|
World of Words (WOW) |
|
Descriptive Test |
 Exams
Exams
| Category | Notification |
|---|---|
| Degree & PG | NPAT 2019 |
| Engineering | HPCET 2019 |
| UPJEE 2019 | |
| AUEET 2019 | |
| VITMEE | |
| Teaching | TS SET 2019 |
| Medical | FMGE 2019 |
 CA
CA
 SP Quiz
SP Quiz
| Category | Quiz |
|---|---|
| Quant Aptitude | |
| Permutation and Combination | |
| Speed and Time | |
| Mensuration | |
| Profit and Loss | |
| Reasoning Ability | Puzzles |
| Seating Arrangement | |
| Direction Sense | |
| General Awareness | General Awareness Quiz |
| Banking Awareness | Banking Awareness Quiz |
| Computer Awareness | Computer Awareness Quiz |