Introduction
Introduction
Permutation and Combination is one of important topic in Quantitative Aptitude Section. In Permutation and Combination – Quiz 5 article candidates can find questions with an answer. By solving this questions candidates can improve and maintain, speed, and accuracy in the exams. Permutation and Combination – Quiz 5 questions are very useful for different exams such as IBPS PO, Clerk, SSC CGL, SBI PO, NIACL Assistant, NICL AO, IBPS SO, RRB, Railways, Civil Services etc.
 Q1
Q1
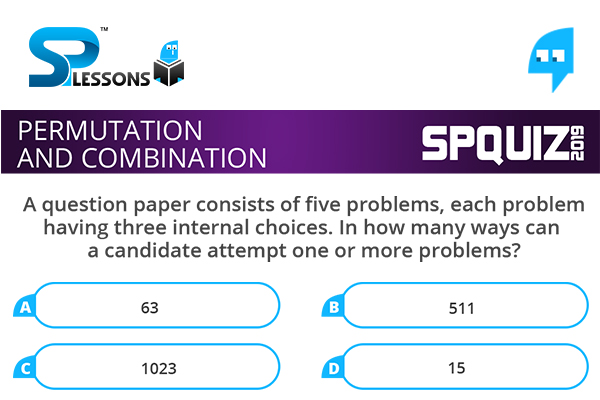
A question paper consists of five problems, each problem having three internal choices. In how many ways can a candidate attempt one or more problems?
- A. 63
B. 511
C. 1023
D. 15
Given that, the question paper consists of five problems. For each problem, one or two or three or none of the choices can be attempted.
Hence, the required number of ways = [latex]{(4)}^{5}[/latex] - 1.
= [latex]{(2)}^{10}[/latex] - 1 = 1024 - 1 = 1023
 Q2
Q2
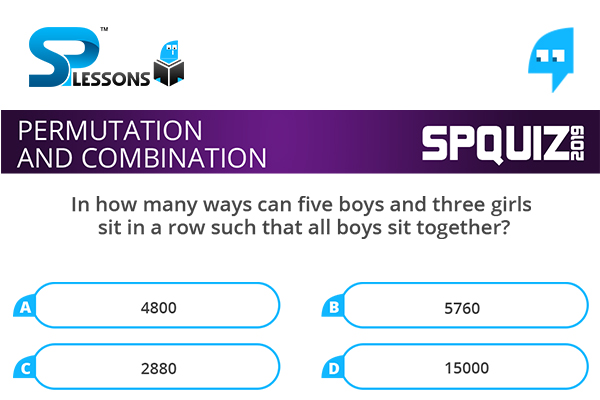
In how many ways can five boys and three girls sit in a row such that all boys sit together?
- A. 4800
B. 5760
C. 2880
D. 15000
Treat all boys as one unit. Now there are four students and they can be arranged in 4! ways. Again five boys can be arranged among themselves in 5! ways.
Required number of arrangements = 4! [latex]\times[/latex] 5! = 24 [latex]\times[/latex] 120 = 2880.
 Q3
Q3
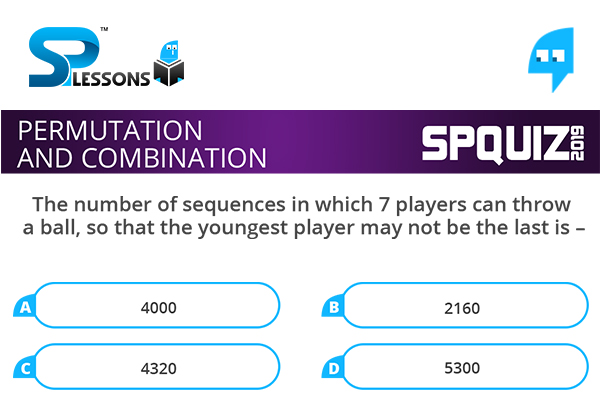
The number of sequences in which 7 players can throw a ball, so that the youngest player may not be the last is -
- A. 4000
B. 2160
C. 4320
D. 5300
The last ball can be thrown by any of the remaining 6 players. The first 6 players can throw the ball in [latex]^{6}{P}_{6}[/latex] ways.
The required number of ways = 6(6!) = 4320
 Q4
Q4
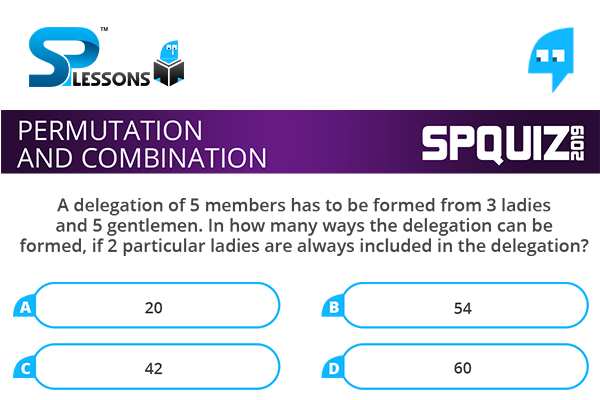
A delegation of 5 members has to be formed from 3 ladies and 5 gentlemen. In how many ways the delegation can be formed, if 2 particular ladies are always included in the delegation?
- A. 20
B. 54
C. 42
D. 60
There are three ladies and five gentlemen and a committee of 5 members to be formed.
Number of ways such that two ladies are always included in the committee = [latex]^{6}{C}_{3} = \frac {(6 \times 5 \times 4)} {6}[/latex] = 20.
 Q5
Q5
The number of new words that can be formed by rearranging the letters of the word 'ALIVE' is -.
- A. 24
B. 23
C. 119
D. 120
Number of words which can be formed = 5! - 1 = 120 - 1 = 119.