Introduction
Introduction
Permutation and Combination is one of important topic in Quantitative Aptitude Section. In Permutation and Combination – Quiz 4 article candidates can find questions with an answer. By solving this questions candidates can improve and maintain, speed, and accuracy in the exams. Permutation and Combination – Quiz 4 questions are very useful for different exams such as IBPS PO, Clerk, SSC CGL, SBI PO, NIACL Assistant, NICL AO, IBPS SO, RRB, Railways, Civil Services etc.
 Q1
Q1
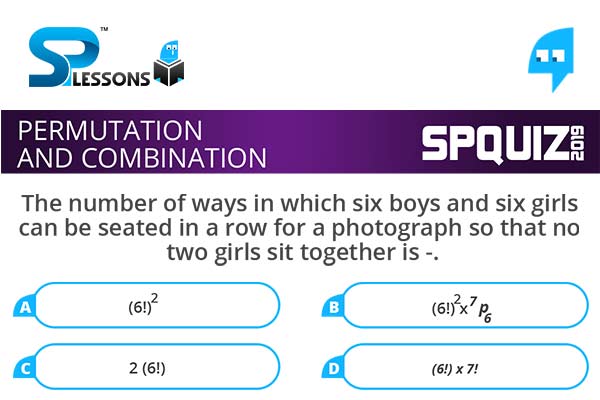
The number of ways in which six boys and six girls can be seated in a row for a photograph so that no two girls sit together is -.
- A. [latex]{(6!)}^{2}[/latex]
B. [latex]{(6!)}^{2} \times ^{7}{p}_{6}[/latex]
C. 2 (6!)
D. [latex](6!) \times 7![/latex]
We can initially arrange the six boys in 6! ways.
Having done this, now three are seven places and six girls to be arranged. This can be done in [latex]^{7}{p}_{6}[/latex] ways.
Hence required number of ways = [latex]{(6!)}^{2} \times ^{7}{p}_{6}[/latex]
 Q2
Q2
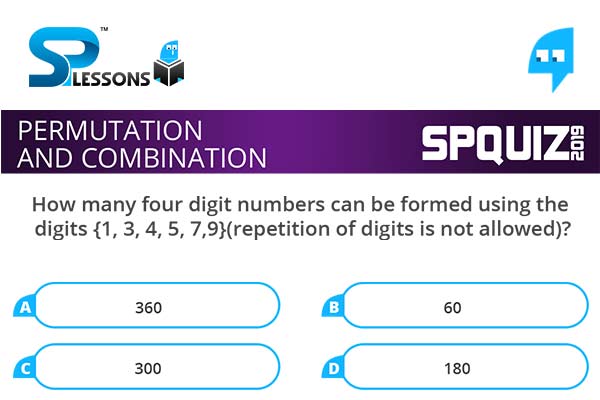
How many four digit numbers can be formed using the digits {1, 3, 4, 5, 7,9}(repetition of digits is not allowed)?
- A. 360
B. 60
C. 300
D. 180
The given digits are six.
The number of four digit numbers that can be formed using six digits is [latex]^{6}{p}_{4}[/latex] = 6 [latex]\times [/latex] 5 [latex]\times [/latex] 4 [latex]\times [/latex] 3 = 360.
 Q3
Q3
In how many ways can six members be selected from a group of ten members?
- A. 4
B. [latex]^{10}{c}_{4}[/latex]
C. 10
D. [latex]^{7}{p}_{6}[/latex]
Six members can be selected from ten members in
[latex]^{10}{c}_{6}[/latex] = [latex]^{10}{c}_{4}[/latex] ways.
 Q4
Q4
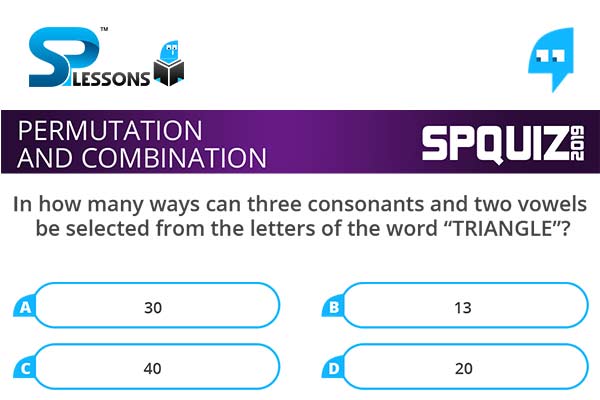
In how many ways can three consonants and two vowels be selected from the letters of the word "TRIANGLE"?
- A. 30
B. 13
C. 40
D. 20
The word contains five consonants. Three vowels, three consonants can be selected from five consonants in [latex]^{5}{c}_{3}[/latex] ways, two vowels can be selected from three vowels in [latex]^{3}{c}_{2}[/latex] ways.
3 consonants and 2 vowels can be selected in [latex]^{5}{c}_{3}[/latex] . [latex]^{3}{c}_{2}[/latex] ways i.e., 10 [latex]\times[/latex] 3 = 30 ways.
 Q5
Q5
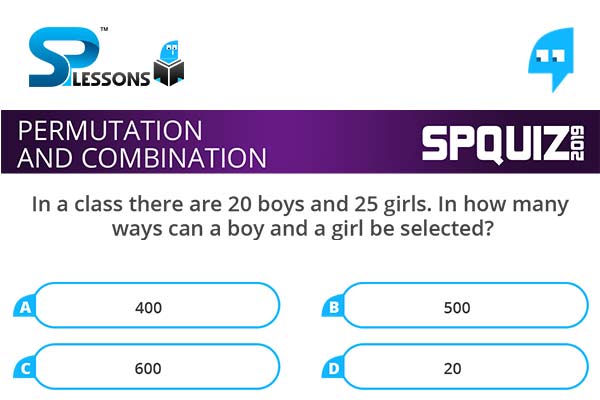
In a class there are 20 boys and 25 girls. In how many ways can a boy and a girl be selected?
- A. 400
B. 500
C. 600
D. 20
We can select one boy from 20 boys in 20 ways.
We select one girl from 25 girls in 25 ways
We select a boy and girl in 20 [latex]\times[/latex] 25 ways i.e., = 500 ways.