Introduction
Introduction
Permutation and Combination is one of important topic in Quantitative Aptitude Section. In Permutation and Combination – Quiz 3 article candidates can find questions with an answer. By solving this questions candidates can improve and maintain, speed, and accuracy in the exams. Permutation and Combination – Quiz 3 questions are very useful for different exams such as IBPS PO, Clerk, SSC CGL, SBI PO, NIACL Assistant, NICL AO, IBPS SO, RRB, Railways, Civil Services etc.
 Q1
Q1
A committee has 5 men and 6 women. What are the number of ways of selecting 2 men and 3 women from the given committee?
- A. 150
B. 200
C. 250
D. 300
The number of ways to select two men and three women = [latex]^{5}{C}_{2} \times ^{6}{C}_{3}[/latex]
= [latex]\frac {(5 \times 4)}{(2 \times 1)}[/latex] [latex]\times[/latex] [latex]\frac {(6 \times 5 \times 4)}{(3 \times 2)}[/latex]
= 200
 Q2
Q2
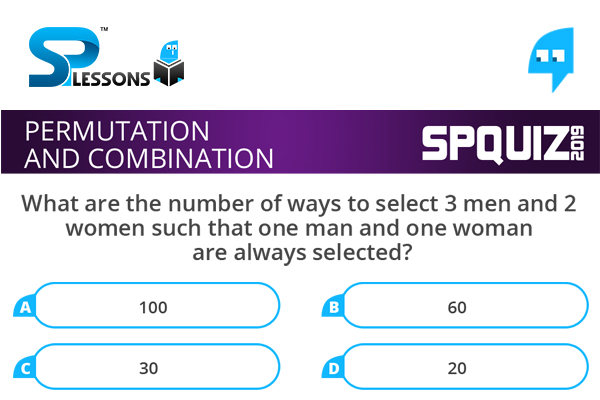
What are the number of ways to select 3 men and 2 women such that one man and one woman are always selected?
- A. 100
B. 60
C. 30
D. 20
The number of ways to select three men and two women such that one man and one woman are always selected = Number of ways selecting two men and one woman from men and five women
= [latex]^{4}{C}_{2} \times ^{5}{C}_{1}[/latex]
= [latex]\frac {(4 \times 3)}{(2 \times 1)}\times 5[/latex]
= 30 ways
 Q3
Q3
A committee has 5 men and 6 women. What are the number of ways of selecting a group of eight persons?
- A. 165
B. 185
C. 205
D. 225
Total number of persons in the committee = 5 + 6 = 11
Number of ways of selecting group of eight persons = [latex]^{11}{C}_{8} \times ^{11}{C}_{3}[/latex] = [latex]\frac {(11 \times 10 \times 9)}{(3 \times 2)}[/latex] = 165 ways
 Q4
Q4
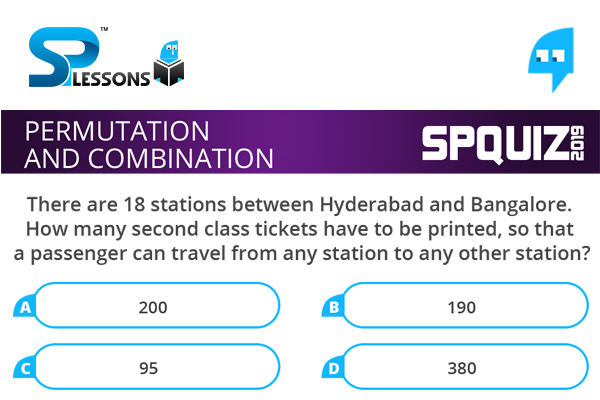
There are 18 stations between Hyderabad and Bangalore. How many second class tickets have to be printed, so that a passenger can travel from any station to any other station?
- A. 200
B. 190
C. 95
D. 380
The total number of stations = 20
From 20 stations we have to choose any two stations and the direction of travel (i.e., Hyderabad to Bangalore is different from Bangalore to Hyderabad) in [latex]^{20}{P}_{2}[/latex] ways.
[latex]^{20}{P}_{2}[/latex] = 20 * 19 = 380.
 Q5
Q5
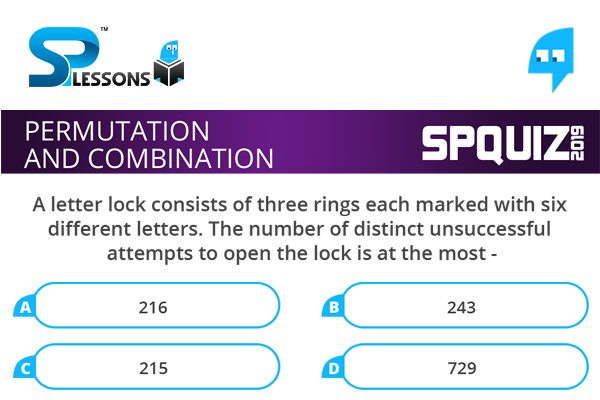
A letter lock consists of three rings each marked with six different letters. The number of distinct unsuccessful attempts to open the lock is at the most -
- A. 216
B. 243
C. 215
D. 729
Since each ring consists of six different letters, the total number of attempts possible with the three rings is = 6 [latex]\times[/latex] 6 [latex]\times[/latex] 6 = 216. Of these attempts, one of them is a successful attempt.
Maximum number of unsuccessful attempts = 216 - 1 = 215.