Introduction
Introduction
Permutation and Combination is one of important topic in Quantitative Aptitude Section. In Permutation and Combination Quiz 10 article candidates can find questions with an answer. By solving this questions candidates can improve and maintain, speed, and accuracy in the exams. Permutation and Combination Quiz 10 questions are very useful for different exams such as IBPS PO, Clerk, SSC CGL, SBI PO, NIACL Assistant, NICL AO, IBPS SO, RRB, Railways, Civil Services etc.
 Q1
Q1
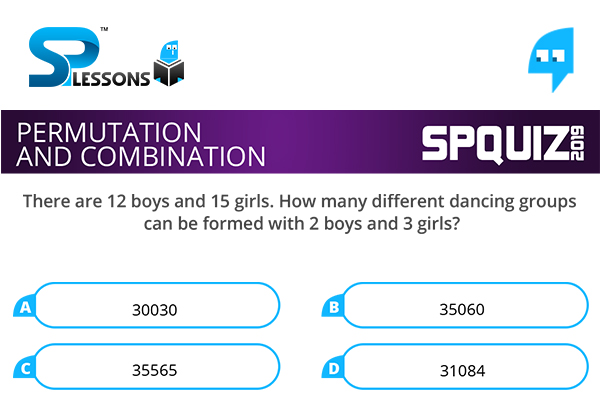
There are 12 boys and 15 girls. How many different dancing groups can be formed with 2 boys and 3 girls?
- A. 30030
B. 35060
C. 35565
D. 31084
Selecting 2 boys from a group of 12 and correspondingly selecting 3 girls from group of 15 girls
[latex]^{12}{C}_{2} \times ^{15}{C}_{3} = 30030[/latex]
i.e, [latex]^{n}{C}_{r} = \frac{n!}{r! \times (n-r)!}[/latex]
 Q2
Q2
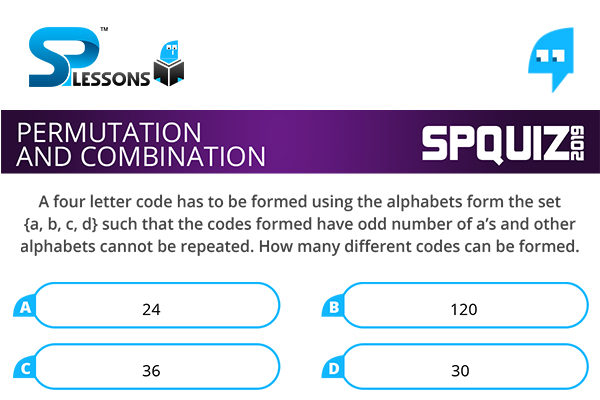
A four letter code has to be formed using the alphabets form the set {a, b, c, d} such that the codes formed have odd number of a's and other alphabets cannot be repeated. How many different codes can be formed
- A. 24
B. 120
C. 36
D. 30
Since the code has to be four lettered and need to have odd number of a’s, the code can have either 1 a or 3 a’s.
If the code has one a, then the remaining letters of the code are b, c and d.
∴ Number of different possible codes = 4! = 24
If the code has three a’s then remaining letter can be chosen in 3c1 = 3 ways
∴ Number of ways of forming the code = 3 × (4! ÷ 3!) = 12 (∵ 3 letters are repeated)
∴ Total number of ways of forming the code = 24 + 12 = 36 ways.
 Q3
Q3
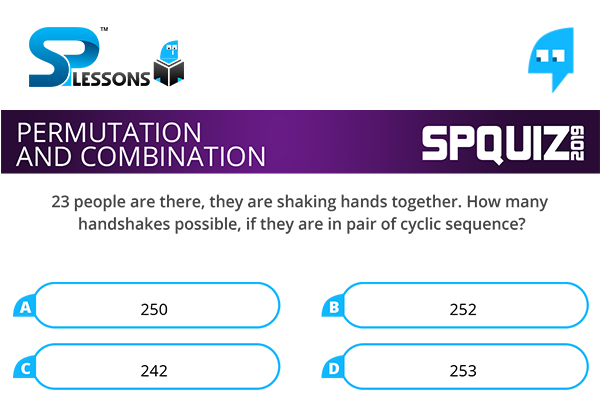
23 people are there, they are shaking hands together. How many handshakes possible, if they are in pair of cyclic sequence?
- A. 250
B. 252
C. 242
D. 253
Every person shakes hand with every other person = 22 hand shakes
⇒ Total handshakes = 22 × 23
But A handshaking with B is same as B handshaking A
⇒ Total handshakes = [latex]\frac{(22 × 23)}{2}[/latex] = 253
 Q4
Q4
How many alphabets need to be there in a language if one were to make 1 million 3 letter initials using the alphabets of the language?
- A. 140
B. 1000
C. 10
D. 100
Suppose we have A number of alphabets in a language.
⇒ Number of ways to make a 3 letter initial = A × A × A = [latex]{A}^{3}[/latex]
Given, number of ways to make 3 letter initial = 1 million = [latex]{10}^{6}[/latex]
⇒ [latex]{A}^{3}[/latex] = [latex]{10}^{6}[/latex]
⇒ A = [latex]{10}^{2}[/latex] = 100
∴ The language needs 100 alphabets
 Q5
Q5
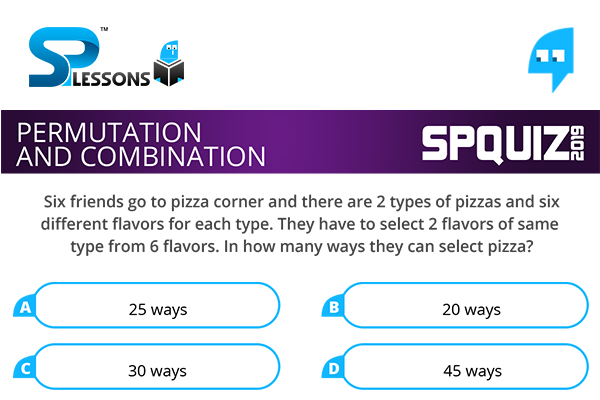
Six friends go to pizza corner and there are 2 types of pizzas and six different flavors for each type. They have to select 2 flavors of same type from 6 flavors. In how many ways they can select pizza?
- A. 25 ways
B. 20 ways
C. 30 ways
D. 45 ways
Number of ways 2 flavors can be selected out of 6 flavors = [latex]^{6}{C}_{2}[/latex] = 15
Number of ways pizza can be selected = 2 [there are 2 types of pizzas]
∴ Number of ways to select a pizza = 2 × 15 = 30 ways
Other Articles
 Study Guide
Study Guide
 Exams
Exams
| Competitive Exams - College Entrance Exams | ||
|---|---|---|
| Category | Notification | |
| Diploma | NITC New Delhi | Goa Diploma Admissions 2019 |
PG |
GATE 2020 |
ATMA 2019 |
| Click Here For – All India Entrance Exam Notifications | ||
 Daily CA
Daily CA
 Job-Alerts
Job-Alerts
 SP Quiz
SP Quiz
| Competitive Exams - Practice Sets | |
|---|---|
| Category | Quiz |
| Quant Aptitude | Direction Sense Quiz |
| English Knowledge | Spotting Errors |
| Insurance Awareness | Insurance Awareness |
 GK
GK
| General Knowledge for Competitive Examinations | |
|---|---|
| Topic | Name of the Article |
| GK - World | June 2019 Awards and Honors |
| Countries Independence Day | |
| International Organizations Headquarters – IGO & INGO | |
| GK - India | Padma Awards 2019 |
| Niti Aayog Objectives for India 2022- 2023 | |
| Indian Rivers | |
| GK - Abbreviations | Indian Government Policies Abbreviations |
| Computer Aptitude Abbreviations | |
| GK - Banking & Insurance | Stock Broking – SEBI Entities |
| Australia Countries Capitals Currencies | |
| GK - Science & Technology | Zoology Terminology |
| IBM IIT Bombay AI Research | |
| Indian Research Institutes | |