Introduction
Introduction
Mensuration is one of important topic in Quantitative Aptitude Section. In Mensuration – Quiz 3 article candidates can find a question with an answer. By solving this question candidates can improve and maintain, speed, and accuracy in the exams. Mensuration – Quiz 3 questions a very useful for different exams such as IBPS PO, Clerk, SSC CGL, SBI PO, NIACL Assistant, NICL AO, IBPS SO, RRB, Railways, Civil Services, etc.
 Q1
Q1
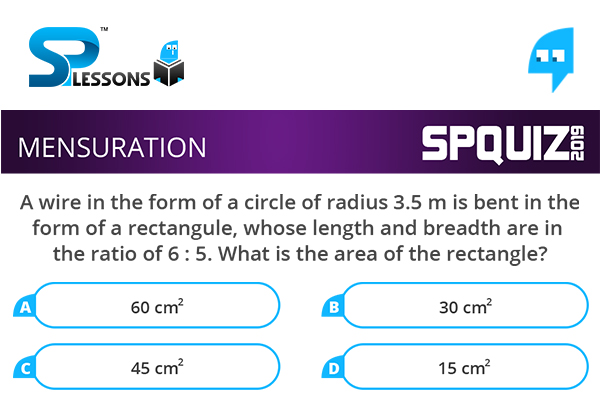
A wire in the form of a circle of radius 3.5 m is bent in the form of a rectangule, whose length and breadth are in the ratio of 6 : 5. What is the area of the rectangle?
- A. 60 [latex]{cm}^{2}[/latex]
B. 30 [latex]{cm}^{2}[/latex]
C. 45 [latex]{cm}^{2}[/latex]
D. 15 [latex]{cm}^{2}[/latex]
The circumference of the circle is equal to the permeter of the rectangle.
Let l = 6x and b = 5x 2(6x + 5x) = 2 [latex]\times \frac{22}{7} \times[/latex] 3.5
[latex]\Rightarrow[/latex] x = 1
Therefore l = 6 cm and b = 5 cm Area of the rectangle = 6 [latex]\times[/latex] 5 = 30 [latex]{cm}^{2}[/latex]
 Q2
Q2
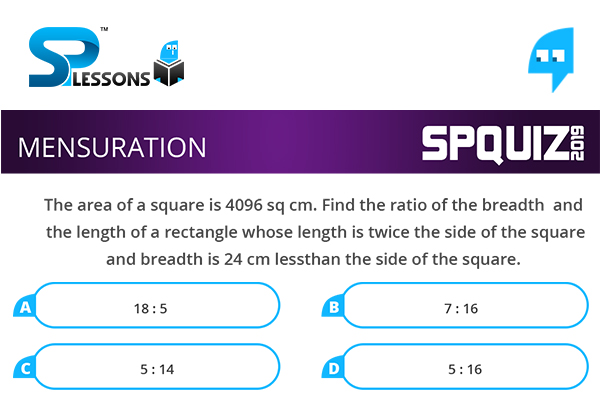
The area of a square is 4096 sq cm. Find the ratio of the breadth and the length of a rectangle whose length is twice the side of the square and breadth is 24 cm less than the side of the square.
- A. 18 : 5
B. 7 : 16
C. 5 : 14
D. 5 : 16
Let the length and the breadth of the rectangle be l cm and b cm respectively. Let the side of the square be a cm.
[latex]{a}^{2}[/latex] = 4096 = [latex]{2}^{12}[/latex]
a = [latex]({{(2)}^{12}})^{\frac{1}{2}}[/latex] = [latex]{2}^{6}[/latex] = 64
L = 2a and b = a - 24
b : l = a - 24 : 2a = 40 : 128 = 5 : 16
 Q3
Q3
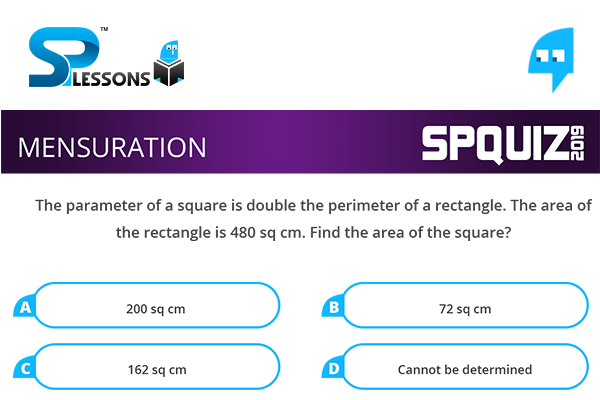
The parameter of a square is double the perimeter of a rectangle. The area of the rectangle is 480 sq cm. Find the area of the square?
- A. 200 sq cm
B. 72 sq cm
C. 162 sq cm
D. Cannot be determined
Let the side of the square be a cm. Let the length and the breadth of the rectangle be l cm and b cm respectively.
4a = 2(l + b)
2a = l + b
l . b = 480
We cannot find ( l + b) only with the help of l . b. Therefore a cannot be found.
Area of the square cannot be found.
 Q4
Q4
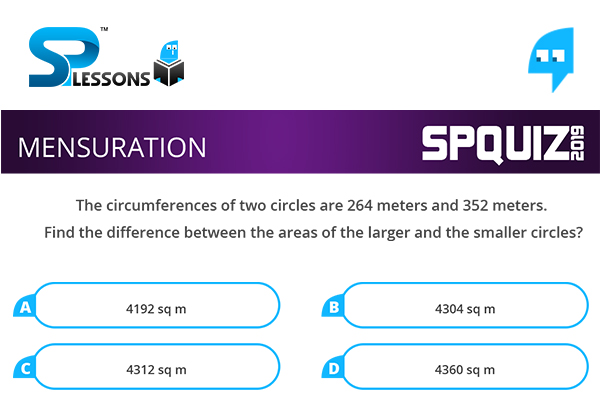
The circumferences of two circles are 264 meters and 352 meters. Find the difference between the areas of the larger and the smaller circles?
- A. 4192 sq m
B. 4304 sq m
C. 4312 sq m
D. 4360 sq m
Let the radii of the smaller and the larger circles be s m and l m respectively.
∏ (mathematics) A symbol representing a product over a set of terms.
2∏s = 264 and 2∏l = 352
s = [latex]\frac{264}{2∏}[/latex] and l = [latex]\frac{352}{2∏}[/latex]
Difference between the areas = ∏[latex]{l}^{2}[/latex] - ∏[latex]{s}^{2}[/latex]
= ∏( [latex]\frac{{176}^{2}}{{∏}^{2}}[/latex] - [latex]\frac{{132}^{2}}{{∏}^{2}}[/latex])
= [latex]\frac{{176}^{2}}{∏}[/latex] - [latex]\frac{{132}^{2}}{∏}[/latex]
= [latex]\frac{(176 - 132)(176 + 132)}{∏}[/latex]
= [latex]\frac{\frac{44}{308}}{\frac{22}{7}}[/latex] = (2)(308)(7) = 4312 sq m
 Q5
Q5
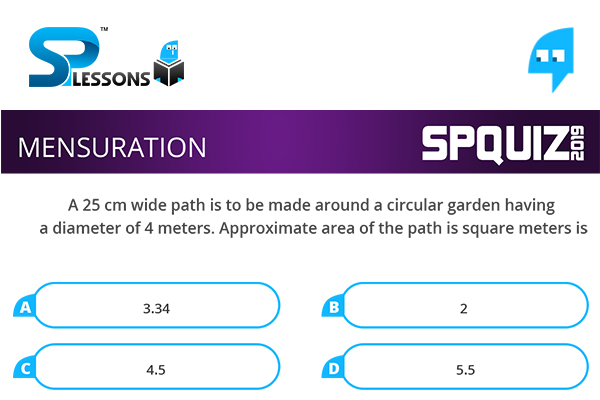
A 25 cm wide path is to be made around a circular garden having a diameter of 4 meters. Approximate area of the path is square meters is
- A. 3.34
B. 2
C. 4.5
D. 5.5
∏ (mathematics) A symbol representing a product over a set of terms.
Area of the path = Area of the outer circle - Area of the inner circle = ∏{4/2 + 25/100}2 - ∏[4/2]2
= ∏[[latex]{(2.25)}^{2}[/latex] - [latex]{2}^{2}[/latex]] = ∏(0.25)(4.25)
=([latex]{a}^{2}[/latex] - [latex]{b}^{2}[/latex]) = (a - b)(a + b)
= (3.14)([latex]\frac{1}{4}[/latex])([latex]\frac{17}{4}[/latex]) = [latex]\frac{53.38}{16}[/latex] = 3.34 sq m