SP Quiz - SPLessons
boy2

Compound Interest Quiz 10

Home > > Tutorial
SPLessons 5 Steps, 3 Clicks
5 Steps - 3 Clicks

Compound Interest Quiz 10

shape Introduction

What is compound interest? Compound interest is the addition of interest to the principal sum of a loan or deposit, or in other words, interest on interest. It is the result of reinvesting interest, rather than paying it out, so that interest in the next period is then earned on the principal sum plus previously accumulated interest.
Compound Interest is one of important topic in Quantitative Aptitude Section. In Compound Interest Quiz 10 article candidates can find questions with answer. By solving this questions candidates can improve and maintain, speed, and accuracy in the exams. Compound Interest Quiz 10 questions are very useful for different exams such as IBPS PO, Clerk, SSC CGL, SBI PO, NIACL Assistant, NICL AO, IBPS SO, RRB, Railways, Civil Services etc.

shape Q1

Rs 3903 is to be divided in a way that A’s share at the end of 7 years is equal to the B’s share at the end of 9 years. If the rate of interest is 4% compounded annually, find A’s share.
    A. Rs 2475 B. Rs 1875 C. Rs 2175 D. Rs 1935

SPLessons

A's share = [latex]{ (1 + \frac {1}{100})}^{7}[/latex]
B's share = [latex]{ (1 + \frac {1}{100})}^{9}[/latex]
Divide both, [latex]\frac {B}{A} {( 1 + \frac {1}{100})}^{2} = \frac {676}{625}[/latex]
So A's share = [latex]\frac {625}{676 + 625} \times 3903[/latex] = Rs 1875

shape Q2

Aswin invested an amount of Rs.9000 in a fixed deposit scheme for 2 years at CI rate 6% pa. How much amount will Aswin get on maturity of the fixed amount ?
    A. Rs.11,230 B. Rs.10,250 C. Rs.10,112 D. Data inadequate

SPLessons

Amout = [latex]\frac {9000 \times 106} {\frac {100 \times 106}{100}}[/latex]
= [latex]\frac {9000 \times 53 }{\frac {50 \times 53}{50}}[/latex]
= 10112

shape Q3

Rs.5200 was partly invested in Scheme A at 10% pa CI for 2 years and Partly invested in Scheme B at 10% pa SI for 4 years. Both the schemes earn equal interests. How much was invested in Scheme A ?
    A. Rs.1790 B. Rs.2200 C. Rs.3410 D. Rs.2670

SPLessons

Amount invested in Scheme B = X
Amount invested in Scheme A = 5200 - X
[latex]\frac {x \times 10 \times 4}{100} = \frac {(5200 - x) \times 21}{100}[/latex]
[latex]{(1 - \frac {10}{100})}^{2} - 1 = \frac {21}{100}[/latex]
[latex]\frac {40x}{100} = \frac {(5200 - x) \times 21}{100}[/latex]
[latex]\frac {2x}{5} = \frac {(5200 - x) \times 21}{100}[/latex]
[latex]200x = 5200 \times 21 \times 5 - x \times 5 \times 21[/latex]
200x = 546000 - 105x
305x = 546000
x = 1790
Scheme A = 5200 - 1790 = 3410

shape Q4

The CI on Rs.7000 for 3 years at 5% for first year, 7% for second year, 10% for the third year will be
    A. Rs.1800 B. Rs.1530 C. Rs.1651 D. Rs.2050

SPLessons

A = [latex]\frac {7000 \times 105}{\frac {100 \times 107}{\frac {107 \times 100}{110 \times 100}}}[/latex]
= [latex]7000 \times 1.05 \times 1.07 \times 1.1[/latex] = 8650.95 = 8651
CI = 8651 - 7000 = 1651

shape Q5

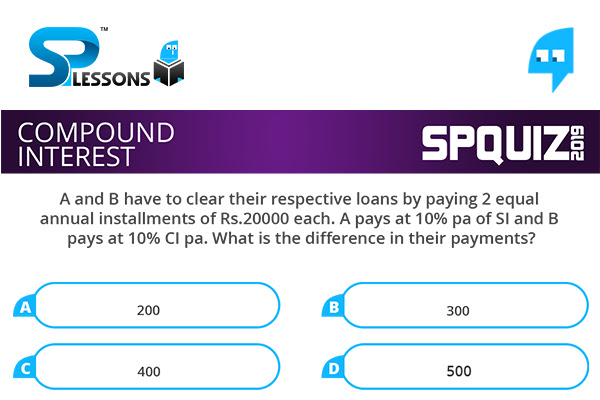
A and B have to clear their respective loans by paying 2 equal annual installments of Rs.20000 each. A pays at 10% pa of SI and B pays at 10% CI pa. What is the difference in their payments?
    A. 200 B. 300 C. 400 D. 500

SPLessons

D = [latex][\frac {20000 \times 110}{\frac {110 \times 100}{100}} - 20000] - \frac {20000 \times 10 \times 2}{100}[/latex]
= [24200 - 20000]-4000
= 4200 - 4000
D = 200
Hide Index Show Index