Introduction
Introduction
Calendar deals with odd days, leap year, ordinary year, counting of odd days, and day of the week related to odd days. To find the day of the week on a given date concept of odd days is used. The article Calendar Quiz 4 lists important Calendar practice questions for competitive exams like RRB ALP/Technical Exams/Junior Engineer Recruitment Exams, SSC, IBPS PO Exams and etc.
 Concepts
Concepts
Odd days:
Extra days, apart from the complete weeks in given periods are called odd days.
Note: An ordinary year has one day while a leap year has two odd days.
Leap year:
A leap year is divisible by 4 except for a century. For a century to be a leap year, it must be divisible by 400.
Examples:
- Years like 1988, 2008 are leap year (divisible by 4).
Centuries like 2000, 2400 are leap year ( divisible by 400).
Years like 1999, 2003 are not leap year (not divisible by 4).
Centuries like 1700, 1800 are not leap year ( not divisible by 400).
In a century, there is 76 ordinary year and 24 leap year.
Note: A leap year has 366 days(52 complete weeks + 2 extra days = 366 days).
Ordinary year:
Ordinary year is other than leap years. A ordinary year has 365 days.
Counting of odd days:
(i) 1 ordinary year = 365 days = (52 weeks + 1 day).
An ordinary year has one odd day.
(ii) 1 leap year = 366 days = (52 weeks + 2 days).
A leap year has 2 odd days.
Day of the week related to odd days:
Let the number of days be 0, 1, 2, 3, 4, 5 , 6 and their days are Sunday, Monday, Tuesday, Wednesday, Thursday, Friday and Saturday respectively.
 Q1
Q1
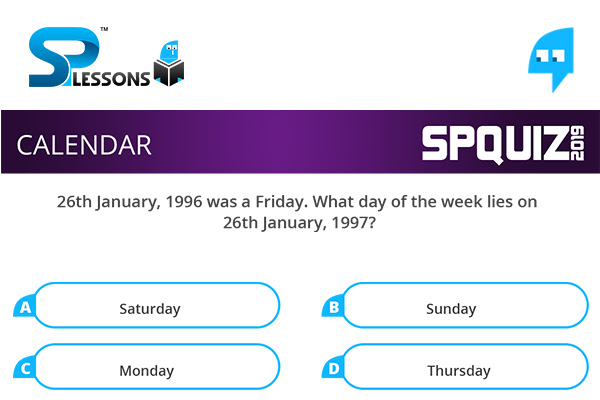
26th January, 1996 was a Friday. What day of the week lies on 26th January, 1997?
- A. Saturday
B. Sunday
C. Monday
D. Thursday
The year 1996 was a leap year. Hence, it adds 2 odd days. As 26th January, 1996 was a Friday, 26th January, 1997 was a Sunday.
 Q2
Q2
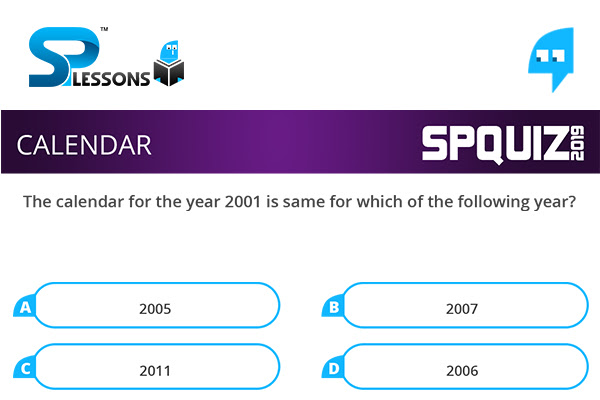
The calendar for the year 2001 is same for which of the following year?
- A. 2005
B. 2007
C. 2011
D. 2006
The total number of odd days from 2001 onwards should be zero.
Now, as an ordinary year adds 1 odd day and a leap year adds 2 odd days
we have:
2001, 2002, 2003, 2005, 2006 – 1 odd day each
2004 – 2 odd days
Hence, at the end of 2006 total number of odd days = 7 or 0
Therefore, the calendar for the year 2001 is repeated in the year 2007.
 Q3
Q3
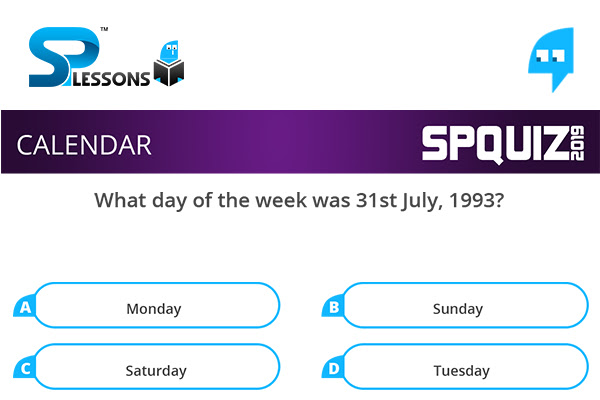
What day of the week was 31st July, 1993?
- A. Monday
B. Sunday
C. Saturday
D. Tuesday
31st July, 1993 = (1992 years + period from 1st January, 1993 to 31st July, 1993)
1600 years have 0 odd days and 300 years have 1 odd day.
Now, the period from 1900 to 1992 have 69 ordinary years and 23 leap years
= [latex](69 \times 1 + 23 \times 2)[/latex] = 115 odd days = (16 weeks + 3 days) = 3 odd days.
= 212 days = (30 weeks + 2 days) = 2 odd days
Therefore, total number of odd days = 1 + 3 + 2 = 6 odd days.
Therefore, the required day was Saturday.
| Januart | February | March | April | May | June | July |
|---|---|---|---|---|---|---|
| 31 | 28 | 31 | 30 | 31 | 30 | 31 |
 Q4
Q4
The last day of the century cannot be:
- A. Sunday
B. Wednesday
C. Friday
D. Saturday
100 years have 5 odd days. Hence the last day of 1st century is a Friday.
200 years have 10 odd days or 1 week + 3 odd days. Hence, the last day of the 2nd century is a Wednesday.
300 years have 15 odd days or 2 week + 1 odd day. Hence, the last day of the 3rd century is a Monday.
400 years have 0 odd days. Hence, the last day of the 4th century is a Sunday.
 Q5
Q5
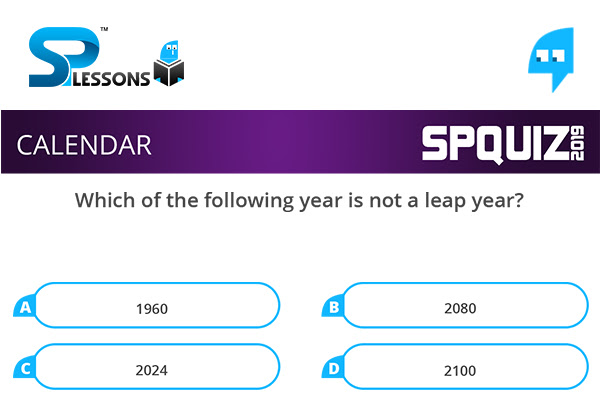
Which of the following year is not a leap year?
- A. 1960
B. 2080
C. 2024
D. 2100
The two conditions that decide that a year is a leap year or not is:
• For a year to be a leap year, it should be divisible by 4.
• No century is a leap year unless it is divisible by 400.
Hence, the year 2100 is not a leap year as it is not divisible by 400.