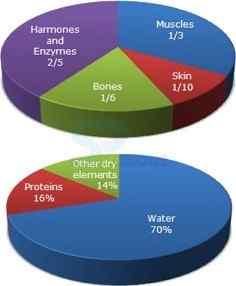
Directions (1-5): Study the following pie-diagrams carefully and answer the questions given below it:

Percentage Composition of Human Body

1. What percent of the total weight of human body is equivalent to the weight of the proteins in skin in human body?

A. 0.016 B. 1.6 C. 0.16 D. Data inadequate

2. What will be the quantity of water in the body of a person weighing 50 kg?

A. 20 kg B. 35 kg C. 41 kg D. 42.5 kg

3. What is the ratio of the distribution of proteins in the muscles to that of the distribution of proteins in the bones?

A. 1 : 18 B. 1 : 2 C. 2 : 1 D. 18 : 1

4. To show the distribution of proteins and other dry elements in the human body, the arc of the circle should subtend at the centre an angle of:

A. 54° B. 126° C. 108° D. 252°

5. In the human body, what part is made of neither bones nor skin?

A. [latex]\frac {1}{40}[/latex] B. [latex]\frac {3}{80}[/latex] C. [latex]\frac {2}{5}[/latex] D. [latex]\frac {11}{15}[/latex]

Answers and Explanations
1. Answer - Option B
Explanation -
Let the body weight be x kg.
Then, weight of skin protein in the body
= 16% of [latex](\frac {1}{10} of x)[/latex] kg
= [latex](\frac {16x}{1000})[/latex]kg
i.e, Required percentage =[latex][\frac {(\frac {16x}{1000})}{x} \times 100][/latex]% = 1.6%
2. Answer - Option B
Explanation -
Quantity of water in the body of a person weighing 50 kg = (70% of 50) kg
= 35 kg.
3. Answer - Option C
Explanation -
Required ratio = [latex]\frac {16 of \frac {1}{3}}{16 of \frac {1}{6}} = \frac {6}{3} = \frac {2}{1} [/latex]
4. Answer - Option C
Explanation -
Percentage of proteins and other dry elements in the body = (16% + 14%)
= 30%.
Therefore Central angle corresponding to proteins and other dry elements together
= 30% of 360°
= 108°.
5. Answer - Option D
Explanation -
Part of the body made of neither bones nor skin = 1 - [latex](\frac {1}{6} + \frac {1}{10}) = \frac {11}{15}[/latex]

Directions (1 to 5): The following pie chart shows the amount of subscriptions generated for India Bonds from different categories of investors.

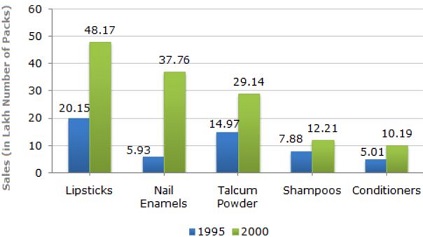
Sales (in lakh number of packs) of five different products of Cosmetic Company during 1995 and 2000

1. The sales of lipsticks in 2000 was by what percent more than the sales of nail enamels in 2000? (rounded off to nearest integer)

A. 33% B. 31% C. 28% D. 22%

2. During the period 1995-2000, the minimum rate of increase in sales is in the case of?

A. Shampoos B. Nail enamels C. Talcum powders D. Lipsticks

3. What is the approximate ratio of the sales of nail enamels in 2000 to the sales of Talcum powders in 1995?

A. 7 : 2 B. 5 : 2 C. 4 : 3 D. 2 :1

4. The sales have increase by nearly 55% from 1995 to 2000 in the case of?

A. Lipsticks B. Nail enamels C. Talcum powders D. Shampoos

5. The sales of conditioners in 1995 was by what percent less than the sales of shampoos in 1995? (rounded off to nearest integer)

A. 57% B. 36% C. 29% D. 25%

Answers and Explanations
1. Answer - Option C
Explanation -
Required percentage = [latex][\frac {(48.17 - 37.76)}{37.76} \times 100][/latex] %
= 27.57%
≈ 28%.
2. Answer - Option A
Explanation -
The percentage increase from 1995 to 2000 for various products are:
Lipsticks = [latex][\frac{(48.17 - 20.15)}{20.15} \times 100][/latex]% = 139.06%.
Nail enamels = [latex][\frac {(37.76 - 5.93)}{5.92} \times 100][/latex]% = 536.76%.
Talcum powders = [latex][\frac {(29.14 - 14.97)}{14.97} \times 100][/latex]% = 94.66%.
Shampoos = [latex][\frac{(12.21 - 7.88)}{7.88}\times 100][/latex]% = 54.95% ≈ 55%.
Conditioners = [latex][ \frac{(10.19 - 5.01)}{5.01} \times 100 ][/latex]% = 103.39%.
Therefore The minimum rate of increase in sales from 1995 to 2000 is in the case of Shampoos.
3. Answer - Option B
Explanation -
Required ratio =[latex]\frac {37.76}{14.97} ≈ 2.5 = \frac {5}{2} [/latex]
4. Answer - Option D
Explanation -
The percentage increase from 1995 to 2000 for various products are:
Lipsticks = [latex][\frac {(48.17 - 20.15)}{20.15} \times 100][/latex] % = 139.06%.
Nail enamels = [latex][\frac {(37.76 - 5.93)}{5.93} \times 100][/latex] % = 536.76%.
Talcum powders =[latex][\frac{(29.14 - 14.97)}{14.97} \times 100][/latex]% = 94.66%.
Shampoos =[latex][\frac {(12.21 - 7.88)}{7.88} \times 100][/latex]% = 54.95% ≈ 55%.
Conditioners = [latex][\frac {(10.19 - 5.01)}{5.01} \times 100][/latex]% = 103.39%.
5. Answer - Option B
Explanation -
Required percentage = [latex][\frac {(7.88 - 5.01)}{7.88} \times 100][/latex] %
= 36.42%
≈ 36%.

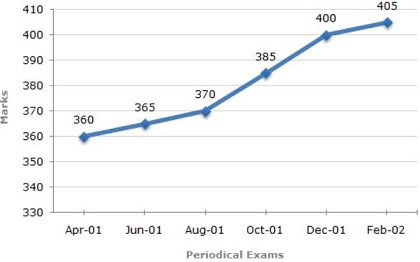
Directions (1 to 5): In a school the periodical examination are held every second month. In a session during April 2001 - March 2002, a student of Class IX appeared for each of the periodical exams. The aggregate marks obtained by him in each perodical exam are represented in the line-graph given below.

Marks Obtained by student in Six Periodical Held in Every Two Months During the Year in the Session 2001 - 2002. Maximum Total Marks in each Periodical Exam = 500

1. In which periodical exams did the student obtain the highest percentage increase in marks over the previous periodical exams ?

A. June, 01 B. August, 01 C. October, 01 D. December, 01

2. The total number of marks obtained in Feb. 02 is what percent of the total marks obtained in April 01 ?

A. 110% B. 112.5% C. 115% D. 116.5%

3. What is the percentage of marks obtained by the student in the periodical exams of August, 01 and Oct, 01 taken together ?

A. 73.25% B. 75.5% C. 77% D. 78.75%

4. What are the average marks obtained by the student in all the periodical exams during the last session ?

A. 373 B. 379 C. 381 D. 385

5. In which periodical exams there is a fall in percentage of marks as compared to the previous periodical exams ?

A. None B. June, 01 C. Oct, 01 D. Feb, 02

Answers and Explanations
1. Answer - Option C
Explanation -
Percentage increase in marks in various periodical exams compared to the previous exams are:
For Jun 01 = [latex][\frac {(365 - 360)}{360} \times 100][/latex] % = 1.39%.
For Aug 01 = [latex][\frac {(370 - 365)}{365} \times 100][/latex] % = 1.37%.
For Oct 01 = [latex][\frac {(385 - 370)}{370} \times 100][/latex] % = 4.05%.
For Dec 01 = [latex][\frac {(400 - 385)}{385} \times 100][/latex] % = 3.90%.
For Feb 02 = [latex][\frac {(404 - 400)}{400} \times 100][/latex] % = 1.25%.
Clearly, highest percentage increase in marks is in Oct 01.
2. Answer - Option B
Explanation -
Here it is clear from the graph that the student obtained 360, 365, 370, 385, 400 and 405 marks in periodical exams held in Apr 01, Jun 01, Aug 01, Oct 01, Dec 01 and Feb 02 respectively.
Required percentage =[latex](\frac {405}{360} \times 100)[/latex]% = 112.5%.
3. Answer - Option B
Explanation -
Required percentage =[latex][\frac {(370 + 385)}{(500 + 500)} \times 100][/latex]% = [latex]\frac {(755 x 100)}{1000}[/latex]% = 75.5%.
4. Answer - Option C
Explanation -
Average marks obtained in all the periodical exams
=[latex]\frac {1}{6} \times [360 + 365 + 370 + 385 + 400 + 405][/latex] = 380.83 ≈ 381.
5. Answer - Option A
Explanation -
As is clear from the graph, the total marks obtained in periodical exams, go on increasing. Since, the maximum marks for all the periodical exams are the same; it implies that the percentage of marks also goes on increasing.
Thus, in none of the periodical exams, there is a fall in percentage of marks compared to the previous exam.