Directions(1–5): Study the following information carefully and answer the questions given below.

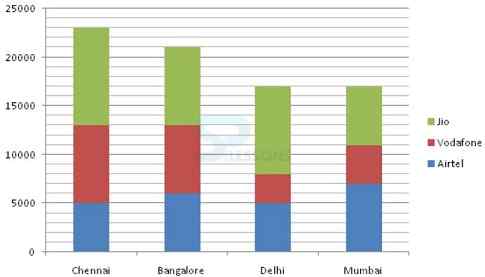
The given bar graph shows the number of person who uses the different networks in different cities.

1. What is the average of the difference between the number of Airtel users in Chennai and Mumbai and the difference between the number of Vodafone users in Delhi and Bangalore?

A. 2000 B. 3000 C. 4000 D. 5000 E. None of these

2. The number of Jio users in Bangalore is approximately what percent of the total number of users in Mumbai all the networks together?

A. 43% B. 45% C. 47% D. 49% E. 41%

3. What is the average number of Vodafone users in all the cities together?

A. 5200 B. 5300 C. 5400 D. 5500 E. None of these

4. What is the ratio of the average number of Airtel and Jio users in Chennai to the average number of Vodafone and Jio users in Delhi?

A. 3 : 2 B. 4 : 3 C. 5 : 4 D. 6 : 5 E. None of these

5. In Bangalore, 60% of the Jio users are male and 80% of Airtel users are female. What is the difference between the male users in Jio and Airtel together and female users in Jio and Airtel together?

A. 1500 B. 2000 C. 3500 D. 3000 E. None of these

Answers and Explanations
1. Answer - Option B
Explanation -
Difference of Airtel user in Chennai and Mumbai = 7000 – 5000 = 2000
Difference of Vodafone user in Delhi and Bangalore = 7000 – 3000 = 4000
Average = [latex]\frac {(2000 + 4000)}{2} = 3000[/latex]
2. Answer - Option C
Explanation -
Required percentage = [latex]\frac {8000}{(7000 + 4000 + 6000)} \times 100 = 47%[/latex]
3. Answer - Option D
Explanation -
Average = [latex]\frac {(8000 + 7000 + 3000 + 4000)}{4} = 5500[/latex]
4. Answer - Option C
Explanation -
Average Airtel and Jio users in Chennai = [latex]\frac {(5000 + 10000)}{2} = 7500[/latex]
Average of Vodafone and Jio users in Delhi = [latex]\frac {(3000 + 9000)}{2} = 6000[/latex]
Required ratio = 7500: 6000 = 5 : 4
5. Answer - Option B
Explanation -
Male user in Jio = [latex]\frac {8000 \times 60}{100} = 4800[/latex]
Female users in Jio = [latex]\frac {8000 \times 40}{100} = 3200[/latex]
Female users in Airtel = [latex]\frac {80}{100} \times 6000 = 4800[/latex]
Number of male users in Airtel = [latex]\frac {20}{100} \times 6000 = 1200[/latex]
Difference = (4800 + 3200) – (4800 + 1200) = 2000

Directions(1–3): What value should come in the place of (?) in the following number series?
1. 4080, 3360, 2730, 2184, 1716, ?

A. 1320 B. 1850 C. 1560 D. 1210 E. 1080

2. 3, 21, 33, 39, 39, ?

A. 52 B. 48 C. 33 D. 28 E. 22

3. 19, 17, 30, 82, 312, ?

A. 1528 B. 1236 C. 1328 D. 1124 E. 1456

4. Three unbiased coins are tossed. What is the probability of getting at least 2 tails?

A. 0.75 B. 0.5 C. 0.25 D. 0.2 E. None of these

5. Tickets numbered 1 to 50 are mixed and one ticket is drawn at random. Find the probability that the ticket drawn has a number which is a multiple of 4 or 7?

A. [latex]\frac {9}{25}[/latex] B. [latex]\frac {9}{50}[/latex] C. [latex]\frac {18}{25}[/latex] D. [latex]\frac {1}{25}[/latex] E. None of these

Answers and Explanations
1. Answer - Option A
Explanation -
[latex]{16}^{3}[/latex] – 16 = 4080
[latex]{15}^{3}[/latex] – 15 = 3360
[latex]{14}^{3}[/latex] – 14 = 2730
[latex]{13}^{3}[/latex] – 13 = 2184
[latex]{12}^{3}[/latex] – 12 = 1716
[latex]{11}^{3}[/latex] – 11 = 1320
2. Answer - Option C
Explanation -
3 + (3 * 6) = 21
21 + (2 * 6) = 33
33 + (1 * 6) = 39
39 + (0 * 6) = 39
39 + (-1 * 6) = 33
3. Answer - Option A
Explanation -
19 * 1 – 2 = 17
17 * 2 – 4 = 30
30 * 3 – 8 = 82
82 * 4 – 16 = 312
312 * 5 – 32 = 1528
4. Answer - Option B
Explanation -
S = {HHH, HHT, HTH, HTT, THH, THT, TTH, TTT}
E = {HTT, THT, TTH, TTT}
n(S) = 8
n(E) = 4
P(E) =[latex]\frac {n(E)}{ n(S)} = \frac {4}{8}[/latex] = 0.5
5. Answer - Option A
Explanation -
S = {1, 2, 3, … , 49, 50}
E = {4, 8, 12, 16, 20, 24, 28, 32, 36, 40, 44, 48, 7, 14, 21, 35, 42, 49}
n(S) = 50
n(E) = 18
P(E) = [latex]\frac {n(E)}{ n(S)} = \frac {18}{50} = \frac {9}{25}[/latex]

1. A dealer marks price of all the goods at 30 % above the cost price and assumes that he will make a profit of 15 % if he offers a discount of 15%. Find what will be his actual profit on sales?

A. 15% B. 30% C. 12.50% D. 10.50% E. None of these

2. A manufacturer sells a pair shoes to a wholesale dealer at a profit of 20 %. Wholesaler sells them to retailer at a profit of 25 %. The shoes are again sold to the customer for Rs. 50.50, thereby earning a profit of 30 %. Find the cost price of manufacturer.

A. Rs. 20.36 B. Rs. 22.90 C. Rs. 25.89 D. Rs. 30.50 E. None of these

3. 6 men can pack 12 boxes in 7 days by working for 7 hours a day. In how many days can 14 men pack 18 boxes if they work for 9 hours a day?

A. 3.5 days B. 5 days C. 7.5 days D. 12 days E. None of these

4. Monika is twice as good as Sonika and together they complete a piece of work in 20 days. In how many days will Monika alone will finish the work?

A. 22 B. 30 C. 37 D. 52 E. 80

5. In a mixture of 13 litres, the ratio of milk and water is 3 : 2. If 3 liters of this mixture is replaced by 3 liters of milk, then what will be the ratio of milk and water in the newly formed mixture?

A. 10 : 3 B. 8 : 5 C. 9 : 4 D. 1 : 1 E. 4 : 4

Answers and Explanations
1. Answer - Option D
Explanation -
Let cost price goods be Rs. 100
Marked price (Selling Price) marked by the shopkeeper on goods = Rs. 130
He sells the goods at a discount of 15 %
Therefore,
Selling price = 85 % of Rs. 130 = Rs. 110.50
Gain = S.P. – C.P. = 110.5 – 100 = 10.50 %
2. Answer - Option C
Explanation -
Profit earned by manufacturer = 20 %
Profit earned by wholesaler = 25 %
Profit earned by retailer = 30%
S.P. of shoes = Rs. 50
Therefore, 130 % of 125 % of 120 % of x = 50.50
=[latex]\frac {120}{100} \times \frac {125}{100} \times \frac {130}{100} \times x = \frac {5050}{100} [/latex]
[latex]\frac {195}{100} x = \frac {5050}{100} [/latex]
x = [latex]\frac {5050 \times 100}{195 \times 100} [/latex]
x = 25.89
Cost price of shoes = Rs. 25.89
3. Answer - Option A
4. Answer - Option B
Explanation -
Hint: If 'A' is 'x' times as good a workman as 'B', then ratio of work done by A & B = x: 1
Monika's 1 day work : Sonika's 1 day work = 2:1
(Monika's + Sonika's ) 1 day work = [latex]\frac {1}{20}[/latex]
Divide [latex]\frac {1}{20}[/latex] in the ratio 2:1 ---------( To divide the number 'a' into ratio x & y , we have first part = [latex]\frac {ax}{x + y}[/latex])
Therefore, Monika's 1 day work = [latex](\frac {1}{20}) \times (\frac {2}{2} + 1) = \frac {1}{30}[/latex]
Hence, Monika will alone finish the work in 30 days.
5. Answer - Option C
Explanation -
Given: Total quantity of mixture = 13 liters
3 litres of mixture is removed from the container – So, let's forget this altogether!
Now, you are left with only 10 litres of mixture in 3:2 ratio.
Milk in 10 litres mix = 10 x [latex]\frac {3}{(2 + 3)}[/latex] = 6 liters
Water in 10 litres mix = 10 x [latex]\frac {2}{(2 + 3)}[/latex] = 4 liters
We add 3 litres milk to this.
So, milk in new mix is = 6 liters + 3 litres = 9 litres
Water= 4 litres
Ratio of milk : water = 9 : 4