Computation of whole numbers:
1. Which one of the following numbers is exactly divisible by 11?

A. 235641 B. 245642 C. 315624 D. 415624

Answer: Option D Explanation: 4 + 5 + 2) - (1 + 6 + 3) = 1, not divisible by 11. (2 + 6 + 4) - (4 + 5 + 2) = 1, not divisible by 11. (4 + 6 + 1) - (2 + 5 + 3) = 1, not divisible by 11. (4 + 6 + 1) - (2 + 5 + 4) = 0, So, 415624 is divisible by 11.
Decimals:
1. What decimal of an hour is a second ?

A. .0025 B. .0256 C. .00027 D. .000126

Answer: Option C Explanation: Required decimal =[latex]\frac{1}{60*60}[/latex] = [latex]\frac{1}{3600}[/latex] =.00027
Fractions and relationships between numbers:
1. If one-third of one-fourth of a number is 15, then three-tenth of that number is ?

A. 35 B. 36 C. 45 D. 54

Answer: Option D Explanation: Let the number be x. Then,[latex]\frac{1}{3}[/latex] of [latex]\frac{1}{4}[/latex] of x = 15 x = 15 x 3 x 4 = 180. So, required number = ([latex]\frac{3}{10}[/latex] * 180) = 54.
Percentage:
1. What percentage of numbers from 1 to 70 have 1 or 9 in the unit's digit?

A. 1 B. 14 C. 20 D. 21

Answer: Option C Explanation: Clearly, the numbers which have 1 or 9 in the unit's digit, have squares that end in the digit 1. Such numbers from 1 to 70 are 1, 9, 11, 19, 21, 29, 31, 39, 41, 49, 51, 59, 61, 69. Number of such number =14 Required percentage =([latex]\frac{14}{70}[/latex] * 100)% = 20%
Ratio & Proportion
1. A sum of money is to be distributed among A, B, C, D in the proportion of 5 : 2 : 4 : 3. If C gets Rs. 1000 more than D, what is B's share?

A. Rs. 500 B. Rs. 1500 C. Rs. 2000 D. None of these

Answer: Option C Explanation: Let the shares of A, B, C and D be Rs. 5x, Rs. 2x, Rs. 4x and Rs. 3x respectively. Then, 4x - 3x = 1000 x = 1000. B's share = Rs. 2x = Rs. (2 x 1000) = Rs. 2000.
Square roots:
1. The least perfect square, which is divisible by each of 21, 36 and 66 is:

A. 213444 B. 214344 C. 214434 D. 231444

Answer: Option A Explanation: L.C.M. of 21, 36, 66 = 2772. Now, 2772 = 2 x 2 x 3 x 3 x 7 x 11 To make it a perfect square, it must be multiplied by 7 x 11. So, required number = [latex]2^{2}[/latex]*[latex]3^{2}[/latex]*[latex]7^{2}[/latex]*[latex]11^{2}[/latex]= 213444
Averages:
1. A grocer has a sale of Rs. 6435, Rs. 6927, Rs. 6855, Rs. 7230 and Rs. 6562 for 5 consecutive months. How much sale must he have in the sixth month so that he gets an average sale of Rs. 6500?

A. Rs. 4991 B. Rs. 5991 C. Rs. 6001 D. Rs. 6991

Answer: Option A Explanation: Total sale for 5 months = Rs. (6435 + 6927 + 6855 + 7230 + 6562) = Rs. 34009. Required sale = Rs. [ (6500 x 6) - 34009 ] = Rs. (39000 - 34009) = Rs. 4991.
Simple Interest:
1. A sum of money at simple interest amounts to Rs. 815 in 3 years and to Rs. 854 in 4 years. The sum is:

A. Rs. 650 B. Rs. 690 C. Rs. 698 D. Rs. 700

Answer: Option C Explanation: S.I. for 1 year = Rs. (854 - 815) = Rs. 39. S.I. for 3 years = Rs.(39 x 3) = Rs. 117. Principal = Rs. (815 - 117) = Rs. 698.
Compound Interest:
1. The difference between simple interest and compound on Rs. 1200 for one year at 10% per annum reckoned half-yearly is:

A. Rs. 2.50 B. Rs. 3 C. Rs. 3.75 D. Rs. 4

Answer: Option B Explanation: S.I. = Rs[latex]\frac{1200*10*1}{100}[/latex] = Rs. 120. C.I. =[latex]1200 \times{(1+\frac{5}{10})}^{2}-1200[/latex] = = Rs. 123. Difference = Rs. (123 - 120) = Rs. 3.
Profit and Loss:
1. A vendor bought toffees at 6 for a rupee. How many for a rupee must he sell to gain 20%?

A. 3 B. 4 C. 5 D. 6

Answer: Option C Explanation: C.P. of 6 toffees = Re. 1 S.P. of 6 toffees = 120% of Re. 1 = Rs.[latex]\frac{6}{5}[/latex] For Rs.[latex]\frac{6}{5}[/latex] ,toffees sold = 6. For Re. 1, toffees sold =(6*[latex]\frac{5}{6}[/latex]) = 5.
Discount:
1. If Rs. 10 be allowed as true discount on a bill of Rs. 110 due at the end of a certain time, then the discount allowed on the same sum due at the end of double the time is:

A. Rs. 20 B. Rs. 21.81 C. Rs. 22 D. Rs. 18.33

Answer: Option D Explanation: S.I. on Rs. (110 - 10) for a certain time = Rs. 10. S.I. on Rs. 100 for double the time = Rs. 20. T.D. on Rs. 120 = Rs. (120 - 100) = Rs. 20. T.D. on Rs. 110 = Rs.([latex]\frac{20}{120}[/latex]*110)= Rs. 18.33
Partnership Business:
1. A, B and C jointly thought of engaging themselves in a business venture. It was agreed that A would invest Rs. 6500 for 6 months, B, Rs. 8400 for 5 months and C, Rs. 10,000 for 3 months. A wants to be the working member for which, he was to receive 5% of the profits. The profit earned was Rs. 7400. Calculate the share of B in the profit.

A. Rs. 1900 B. Rs. 2660 C. Rs. 2800 D. Rs. 2840

Answer: Option B Explanation: For managing, A received = 5% of Rs. 7400 = Rs. 370. Balance = Rs. (7400 - 370) = Rs. 7030. Ratio of their investments = (6500 x 6) : (8400 x 5) : (10000 x 3) = 39000 : 42000 : 30000 = 13 : 14 : 10 B's share = Rs.(7030*[latex]\frac{14}{37}[/latex]= Rs. 2660.
Mixture and Alligation:
1. A container contains 40 litres of milk. From this container 4 litres of milk was taken out and replaced by water. This process was repeated further two times. How much milk is now contained by the container?

A. 26.34 litres B. 27.36 litres C. 28 litres D. 29.16 litres

Answer: Option D Explanation: Amount of milk left after 3 operations = [40(1-[latex]\frac{4}{40})^{3}[/latex]]litres =(40*[latex]\frac{9}{10}[/latex]*[latex]\frac{9}{10}[/latex]*[latex]\frac{9}{10}[/latex])= 29.16 litres.
Time and distance:
1. Excluding stoppages, the speed of a bus is 54 kmph and including stoppages, it is 45 kmph. For how many minutes does the bus stop per hour?

A. 9 B. 10 C. 12 D. 20

Answer: Option B Explanation: Due to stoppages, it covers 9 km less. Time taken to cover 9 km =([latex]\frac{9}{54}[/latex]*60)min= 10min
Time & Work:
1. A and B can together finish a work 30 days. They worked together for 20 days and then B left. After another 20 days, A finished the remaining work. In how many days A alone can finish the work?

A. 40 B. 50 C. 54 D. 60

Answer: Option D Explanation: (A + B)'s 20 day's work =([latex]\frac{1}{30}[/latex]*20) = [latex]\frac{2}{3}[/latex] Remaining work =(1-[latex]\frac{2}{3}[/latex])= [latex]\frac{1}{3}[/latex] Now, [latex]\frac{1}{3}[/latex]work is done by A in 20 days. Therefore, the whole work will be done by A in (20 x 3) = 60 days.
Simplifications:
1. A man has Rs.480 in the denominations of one-rupee notes, five-rupee notes and ten-rupee notes. The number of notes of each denomination is equal. What is the total number of notes that he has ?

A. 45 B. 60 C. 75 D. 90

Answer: Option D Explanation: Let number of notes of each denomination be x. Then x + 5x + 10x = 480 16x = 480 x = 30. Hence, total number of notes = 3x = 90.
Triangle and its various kinds of centres:
1. A boat having a length 3 m and breadth 2 m is floating on a lake. The boat sinks by 1 cm when a man gets on it. The mass of the man is:

A. 12 kg B. 60 kg C. 72 kg D. 96 kg

Answer: Option B Explanation: Volume of water displaced= (3 x 2 x 0.01)[latex] m^{3}[/latex] = 0.06 [latex] m^{3}[/latex] Mass of man = Volume of water displaced x Density of water = (0.06 x 1000) kg = 60 kg.
Heights and Distances:
1. A man standing at a point P is watching the top of a tower, which makes an angle of elevation of 30° with the man's eye. The man walks some distance towards the tower to watch its top and the angle of the elevation becomes 60°. What is the distance between the base of the tower and the point P?

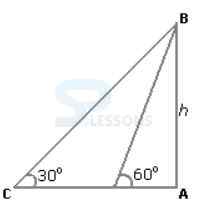
A. 43 units B. 8 units C. 12 units D. Data inadequate

Answer: Option D Explanation: One of AB, AD and CD must have given. So, the data is inadequate
Pie Charts:
The following pie-chart shows the percentage distribution of the expenditure incurred in publishing a book. Study the pie-chart and the answer the questions based on it.
1. If the difference between the two expenditures are represented by 18° in the pie-chart, then these expenditures possibly are

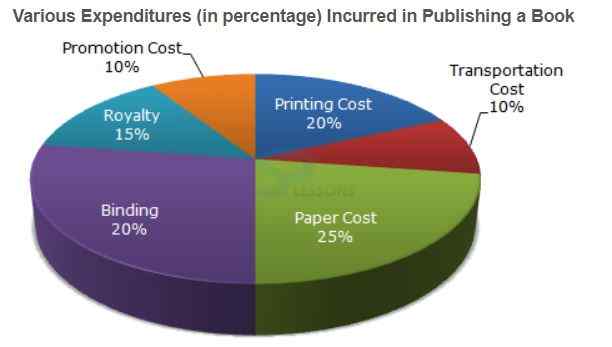
A. Binding Cost and Promotion Cost B. Paper Cost and Royalty C. Binding Cost and Printing Cost D. Paper Cost and Printing Cost

Answer: Option D Explanation: Central angle of 18° =([latex]\frac{18}{360}[/latex]*100)%of the total expenditure = 5% of the total expenditure. From the given chart it is clear that: Out of the given combinations, only in combination (d) the difference is 5% i.e. Paper Cost - Printing Cost = (25% - 20%) of the total expenditure = 5% of the total expenditure.
2.For an edition of 12,500 copies, the amount of Royalty paid by the publisher is Rs. 2,81,250. What should be the selling price of the book if the publisher desires a profit of 5%?

A. Rs. 152.50 B. Rs. 157.50 C. Rs. 162.50 D. Rs. 167.50

Answer: Option B Explanation: Clearly, S.P. of the book = 105% of C.P. Let the selling price of this edition (of 12500 books) be Rs. x. Then, 15 : 105 = 281250 : x x = Rs.([latex]\frac{105 x 281250}{15}[/latex])= Rs. 1968750 S.P. of one book = Rs.([latex]\frac{1968750}{12500}[/latex])= Rs. 157.50
3. If for an edition of the book, the cost of paper is Rs. 56250, then find the promotion cost for this edition.

A. Rs. 20,000 B. Rs. 22,500 C. Rs. 25,500 D. Rs. 28,125

Answer: Option B Explanation: Let the Promotion Cost for this edition be Rs. p. Then, 25 : 10 = 56250 : p p = Rs.([latex]\frac{56250 x 10}{25}[/latex])= Rs. 22,500
Bar Charts:
The bar graph given below shows the foreign exchange reserves of a country (in million US $) from 1991 - 1992 to 1998 - 1999.

1. The ratio of the number of years, in which the foreign exchange reserves are above the average reserves, to those in which the reserves are below the average reserves is?

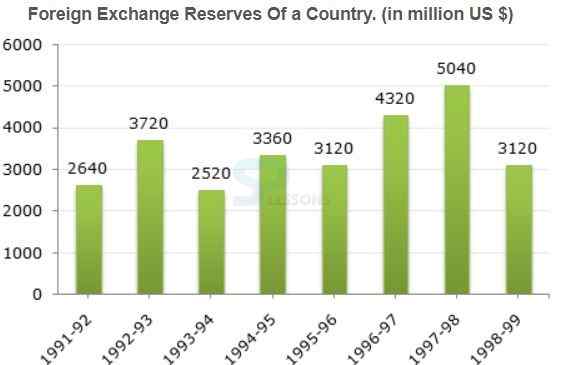
A. 2:6 B. 3:4 C. 3:5 D. 4:4

Answer: Option C Explanation: Average foreign exchange reserves over the given period = 3480 million US $. The country had reserves above 3480 million US $ during the years 1992-93, 1996-97 and 1997-98, i.e., for 3 years and below 3480 million US $ during the years 1991-92, 1993-94, 1994-95, 1995-56 and 1998-99 i.e., for 5 years. Hence, required ratio = 3 : 5.
2. The foreign exchange reserves in 1997-98 was how many times that in 1994-95?

A. 0.7 B. 1.2 C. 1.4 D. 1.5

Answer: Option D Explanation: Required ratio =[latex]\frac{5040}{3360}[/latex]= 1.5.
3. What was the percentage increase in the foreign exchange reserves in 1997-98 over 1993-94?

A. 100 B. 150 C. 200 D. 620

Answer: Option A Explanation: Foreign exchange reserves in 1997 - 1998 = 5040 million US $. Foreign exchange reserves in 1993 - 1994 = 2520 million US $. Therefore Increase = (5040 - 2520) = 2520 US $. Therefore Percentage Increase =([latex]\frac{2520}{2520}[/latex]*100)= 100%