Prepositions
1. Under this heavy load the bridge will give --------

A. in B. out C. way D. away

Answer: Option C
2. The person refused to grovel _________ the feet of his master.

A. on B. about C. at D. by

Answer: Option C
Active Voice and Passive Voice
1. This house ———————- in 1970 by my grandfather.

A. Built B. Was built C. Was build D. Has built

Answer: Option B
2. The robbers ————————- by the police.

A. Have arrested B. Have been arrested C. Was arrested D. Had arrested

Answer: Option B
Joining Sentences
1. It is raining. I will attend the office. (A) Since it is raining ..... (B) Though it is raining ..... (C) It is raining, nevertheless .....

A. Only A B. A and B C. Only B D. B and C E. Only C

Answer: Option D
Explanation: The two sentences are contradictory. Though, although, nevertheless, yet etc. can be used to combine the sentences without changing the meaning conveyed.
2. The police started firing. The mob dispersed immediately. (A) As soon as the police start ..... (B) No sooner did the police start ..... (C) Although the police started .....

A. A and C B. Only B C. A and B D. Only C E. All the three

Answer: Option C
Explanation: The second sentence is the effect of the action stated in the first sentence. It also mentions the time is taken (i.e.) 'immediately'. Hence conjunctions like 'No sooner ... 'then' and 'as soon as' can be used to express the idea precisely.
Spotting Errors
1. To perform this experiment (a) / drop little sugar (b) / into a glass of water(c) / No error (d)

A. to perform this experiment B. drop little sugar C. into a glass of water D. no error

Answer: Option B
2. These display (a) / the (b) / remarkable variety (c) / No error (d)

A. these display B. the C. remarkable variety D. no error

Answer: Option B
Synonyms
1. Upright

A. Honorable B. Horizontal C. Humble D. Supine

Answer: Option A
Explanation: Upright can mean either honorable or vertical; horizontal and supine are both antonyms of upright
2. Reverie

A. Palimpsest B. Phantom C. Daydream D. Curio

Answer: Option C
Explanation: A reverie means the state of being lost in thought or a daydream
Sentence Improvement
1. In summer, days are more warmer than nights.

A. warmer B. warmest C. warmer D. none

Answer: Option C
2. He killed the enemy by his sword.

A. by sword B. by a sword C. with his sword D. none

Answer: Option C
Error Correction (Phrase in colour)
1. Unless I have a peaceful environment, I will be not able In case of preserving our sense of community and common citizenship, we have to keep things like police protection, freedom of education and basic education outside the realm of market, ensuring that they are provided equally to all.

A. In order to preserve B. In order to preserve C. In case to preserve D. In the order of preserving E. No correction required

Answer: Option B
Explanation: When there are two parts of a verb given in a sentence (will be) the negative has to come between the two (i.e) 'will not be'.
2. In case of preserving our sense of community and common citizenship, we have to keep things like police protection, freedom of education and basic education outside the realm of the market, ensuring that they are provided equally to all.

A. In order to preserve B. In order to preserve C. In case to preserve D. In the order of preserving E. No correction required

Answer: Option B
Explanation: 'In case of something' means if something happens the use of which is irrelevant in the given context. 'In order to do something' which means with the purpose or intention of doing or achieving something is most appropriate. Hence the correction is 'In order to preserve'.
Para Completion
1. Self-sufficiency is a way of life in the Democratic Republic of Congo's rural expanses. ---- Yet in these uncompromising conditions, one of Africa's more successful public health programmes is not only surviving but thriving. (A) Even the most accessible of the many villages that dot the dense rain forest get little government support. (B) The idea is to harness people's existing culture of self-help and get subsistence farmers to carry out simple medical tasks which are beyond the capacity of a pathetically inadequate health system. (C) Training and maintaining hundreds of thousands of community workers, to work in the self-help groups involves a lot of patience and money.

A. Only A B. A and B C. A and C D. B and C E. Only C

Answer: Option A
Explanation: The first statement tells that self-sufficiency is a way of life in the rural areas of the Democratic Republic of Congo. Statement A says how self-sufficiency has become a way of life(because they get little government support). The last statement says that despite these 'uncompromising conditions'. (i.e. the lack of government support). Africa's successful public health programme is thriving. Hence statement A forms a logically coherent paragraph with the two given statements.
2. In India, at least in the large swathes of it, except perhaps Kashmir and the north-east, winter brings in a cheer that all look forward to and cherish the experience --- And that to multitudes of sun-persecuted Indians is the most that can be asked for. (A) If you regularly take the Virar-Churchgate fast in Mumbai, the tram in Kolkata, the steaming autos in Chennai and the rough DTC buses in Delhi you will know how mercifully the little bit of weather respite is received by one and all. (B) It doesn't diminish the throbbing crowd, but it lessens their suffering. (C) The fury of an abusive summer has subsided and all things around, things alive and inanimate are in ecstasy.

A. Only A B. A and B C. A and C D. Only C E. All the three

Answer: Option B
Explanation: The first statement of the passage describes how winter brings in cheer to the people living in several parts of India. Statement A says winter brings in a pleasant respite from the sweltering heat. Statement B is a continuation of A and ideally precedes the last statement which sums up the paragraph by saying that to a majority of Indians tormented by the summer heat, winter diminishes their suffering to a large extent. Hence, statement A and B form a coherent paragraph with the two given statements.
Sentence Completion
1. His moral decadence was marked by his ----------- from the ways of integrity and honesty.

A. departure B. obsession C. opprobrium D. declivity

Answer: Option A
2. She had a terrible night caused by an ----------- during her sleep.

A. debility B. incubus C. delusion D. obsession

Answer: Option D
Fill in the blanks
1. _____ under the scourge of violence and militancy for the past 18 years, Jammu and Kashmir has endured widespread death and destruction. (A) Roiling (B) Reeling (C) Shaking (D) Venting (E) Erupting (F) Reeking

A. A and B B. B and C C. B and E D. E and F E. C and E

Answer: Option B
Explanation: It is clear that Jammu and Kashmir are directly taken the brunt of terrorism. Hence it is 'reeling' or 'shaking' under its impact.
2. Jobs in call centers are highly _____ leading to alarming consequences like hypertension, insomnia, coronary malfunctions among young employees. (A) stressful (B) laborious (C) taxing (D) dangerous (E) vexatious (F) distressing

A. A and C B. A and B C. B and D D. D and E E. E and F

Answer: Option A
Explanation: 'Stressful' or 'taxing' jobs lead to diseases like hypertension.
Error Correction (Underlined Part)
1. On my birthday, I distributed sweets between the students of my class.

A. between students of B. among students of C. among the students of D. between the students in E. No correction required

Answer: Option C
Explanation: When more than two people are mentioned 'among' is used and not 'between'. Students should be preceded by 'the' because the reference is to a specific group of students.
2. My friend got his daughter married with an engineer.

A. married to an engineer B. marry an engineer C. marry with an engineer D. married with the engineer E. No correction required

Answer: Option A
Explanation: The preposition that follows married is 'to' and not 'with'.
Passage Completion
Direction (1 - 3): In economics, the term recession generally describes the reduction of a country’s Gross Domestic Product (GDP) for at least two quarters. A recession is…( I )…by rising unemployment, an increase in government borrowing,…( II)…, of share and stock prices, and falling investment. All of these characteristics have effects on people. Some recessions have been anticipated by stock market declines. The real estate market also usually…( III )…before a recession.
1. Choose the best word in place of ( I ) from the given passage

A. imagined B. depict C. shown D. visualized E. characterized

Answer: Option E
2. Choose the best word in place of ( II ) from the given passage

A. Increase B. variance C. more D. decrease E. abundance

Answer: Option D
Substitution
1. A short walk for pleasure or exercise.

A. jog B. stroll C. gallop D. promenade

Answer: Option B
2. A poem of mouring.

A. epic B. sonnet C. lyric D. elegy

Answer: Option D
Idioms and Phrases
1. To lead a dog's life

A. to live happily and successfully B. to have an unhappy life, full of problems or unfair treatment C. to live in a situation where there is a lot of competition D. to be often criticized by others ruthlessly E. to be easily persuaded by others in believing what they say

Answer: Option B
Explanation: 'To lead a dog's life' is to have an unhappy life, with full of problems.
2. To separate the sheep from the goats

A. to short out the old from the new B. to become less shy and more confident C. to annoy someone very much D. to use complicated language that is difficult to understand E. to distinguish good people from bad people

Answer: Option E
Explanation: When a person has the ability to 'separate or sort out the sheep from the goals' it means that the person can distinguish between good and bad people.
Antonyms
1. SMOOTHLY

A. suavely B. calmly C. placidly D. urbanely E. abrasively

Answer: Option E
Explanation: Abrasively (harshly or roughly) is an antonym of smoothly.
2. ASSUAGEMENT

A. distress B. composure C. comfort D. disturbance E. aloofness

Answer: Option A
Explanation: Distress (torment, trouble) is an antonym of assuagement which means to make (an unpleasant feeling) less intense.
Sentence Arrangement
1. I read an advertisement that said (P) posh, air-conditioned (Q) gentlemen of taste (R) are available for (S) fully furnished rooms

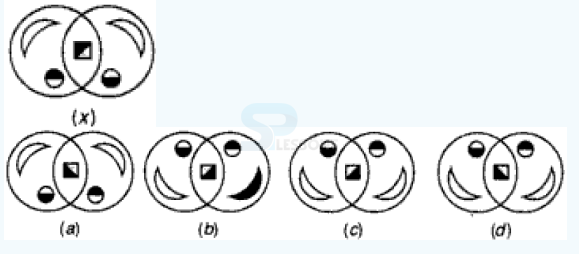
A. PSRQ B. SRPQ C. PSQR D. PQRS

Answer: Option A
2. It is a rule (P) by anyone and admit (Q) that must not (R) of no variation (S) be violated

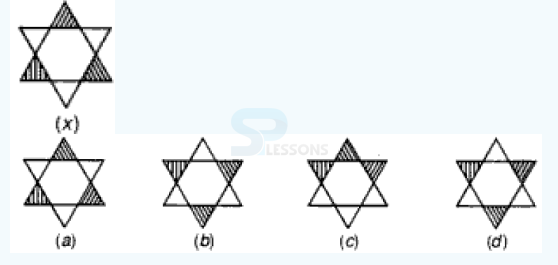
A. QSPR B. PSQR C. QPRS D. RQPS

Answer: Option A

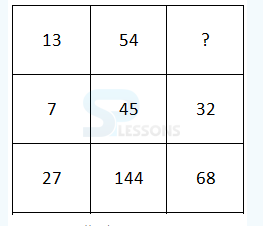
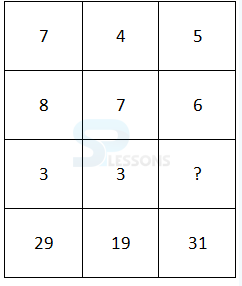
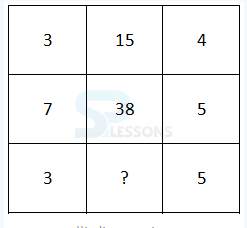
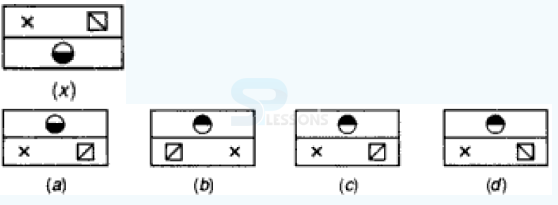
Number Coding
1. In a certain language, CAP is coded as 66, how will PEN be coded

A. 40 B. 66 C. 80 D. 46

Answer: Option D
Explanation: This is an interesting question and you will often get confused. Because even if you try to add the numeric value you won’t get the answer. But here reverse numeric value concept is used. So, in this question, the reverse numeric values are considered and added. C=24, A=26, P=11. 24+26+11=61 and the same logic for PEN. P=11, E=22 and N=13. Answer=46
2. In a certain language, TEARS is coded as 18, so how will be WATER coded

A. 25 B. 22 C. 18 D. 20

Answer: Option B
Explanation: Numeric position of each letter is first added until it reaches to a single digit number and then all the numeric values are added. T=20 so 2+0=2, E=5 A=1 R=18, 1+8=9 S=19, 1+9=10, 1+0=1; 2+5+1+9+1=18
3. If 7 x 5 = VIII ; 5 x 6 = III ; 5 x 3 = VI , then 9 x 4 = ?

A. IX B. II C. VIII D. XXII

Answer: Option A
Explanation: 7 x 5 = 35 ; 3+5 = 8 = VIII in roman numeral format 5 x 6 = 30 ; 3+0 = 3 = III in roman numerals. 9 x 4 = 36 = 3+6 = 9 = IX in roman numerals.
Inserting Correct Mathematical Sign
1. Which one of the following equation will be possible when you interchange the 4 and 5 numbers and + and x signs?

A. 5 x 4 + 10 = 30 B. 10 x 4 + 5 = 50 C. 20 + 5 x 4 = 104 D. 5 + 15 x 4 = 90

Answer: Option C
2. What two signs in the following equation need to be interchanged so that the equation will be mathematically correct? 5 + 3 x 8 – 12 ÷4 = 3

A. – and + B. x and ÷ C. ÷and – D. None of these

Answer: Option C
3. What will be the correct equation when the signs – and ÷and numbers 4 and 8 are interchanged?

A. 4 ÷8 – 2 = 6 B. 6 – 8 ÷4 = -1 C. 4 – 8 ÷6 = 2 D. 8 – 6 ÷4 = 1

Answer: Option A
Odd Man Out
1. Pick the odd one out.

A. May: January B. September: November C. October: April D. January: December E. October: July

Answer: Option C
Explanation: In all options except C, Both the month names are the pair of either 31 or 30 while in option C, they are the pair of 31 – 30.
2. Pick the odd one out.

A. 2: 4 B. 5: 25 C. 10: 100 D. 3: 9 E. 6: 60

Answer: Option E
Explanation: In all options except E, the Second number is Square of the first number.
3. Pick the odd one out.

A. Dog: Kennels B. Rabbit: Burrows C. Lion: Den D. Spider: Spider-Web E. Hen: Coops

Answer: Option D
Explanation: All options having Animals and their homes except for option D (Spider spins a cobweb to live in).
Mutual Relation Problem
1. If (i) M is brother of N; (ii) B is brother of N; (iii) M is brother of D, then which of the following statements is definitely true ?

A. N is the brother of B B. N is the brother of D C. M is the brother of B D. D is the brother of M E. None of these

Answer: Option C.
Explanation: M is the brother of N and B is the brother of N. So, M is the brother of B.
2. Give that: 1. A is the brother of B. 2. C is the brother of A. 3. D is the brother of E. 4. E is the brother of B. Then an uncle of D is

A. A B. B C. C D. E

Answer: Option A.
Explanation: Clearly, D is the brother of E and E is the daughter of B. So, D is the son of B. Also, A is the brother of B. So, A is the uncle of D.
3. A is the son of B. C, B`s sister has a son D and a daughter E. F is the maternal uncle of D. How is A related to D?

A. Cousin B. Nephew C. Uncle D. Brother

Answer: Option A.
Explanation: A is the son of B and D is the son of the sister of B. So, A is the cousin of D.
Number Puzzle
1. Find the missing number?

A. 3 B. 4 C. 5 D. 7

Answer: Option B
Explanation: In the first column, 13 + 7 * 2 = 27. In the second column, 54 + 45 * 2 = 144. Let the missing number in the third column be x. Then, x + 32 * 2 = 68 => x = 4.
2. Find the missing number?

A. 5 B. 4 C. 6 D. 7

Answer: Option A
Explanation: In the first column, 29 - 8 = 7 * 3 = 21. In the second column, 19 - 7 = 4 * 3 = 12. Let the missing number in the third column be x. Then, 31 - 6 = 5 * x => 5x = 25 => x = 5. 3. Find the missing number?

A. 20 B. 18 C. 15 D. 12

Answer: Option B
Explanation: In the first row, 3 * 4 + 3 = 15. In the second row, 7 * 5 + 3 = 38. In the third row, missing number = 3 * 5 + 3 = 18.
Human Relation
1. A woman introduces a man as the son of the brother of her mother. How is the man related to the women?

A. Nephew B. Son C. Cousin D. Uncle E. Grandson

Answer: Option C
Explanation: Brother of mother - uncle; Uncle's son - Cousin.
2. A man said to a lady, "Your mother's husband's sister is my aunt." How is the lady related to the man?

A. Daughter B. Granddaughter C. Mother D. Sister E. Aunt

Answer: Option D
Explanation: Your mother's husband - Your father; your father's sister - Your aunt. So, lady's aunt is man's aunt and therefore lady is man's sister.
3. If Neena says, "Anita's father Raman is the only son of my father-in-law Mahipal", then how is Bindu, who is the sister of Anita, related to Mahipal?

A. Niece B. Daughter C. Wife D. Daughter-in-law E. None of these

Answer: Option E
Explanation: Only son of Neena' s father-in-law Mahipal - Neena's husband. So, Raman is Neena's husband and Anita and Bindu are his daughters. Thus, Bindu is the granddaughter of Mahipal.
Non-Verbal Reasoning
1. Choose the correct water - image of the fig. (x) from the given four figures.

Answer: Option C
2. Choose the correct water - image of the fig. (x) from the given four figures.

Answer: Option D
3. Choose the correct water - image of the fig. (x) from the given four figures.

Answer: Option D
Distance and Direction Sense Test
1. One morning, after sunrise, Vikram and Shailesh were standing in a lawn with their backs towards each other. Vikram's shadow fell exactly towards left-hand side. Which direction was Shailesh facing?

A. East B. West C. North D. South

Answer: Option D
Explanation: Since Vikram's shadow fell towards left, therefore, Vikram is facing North. So, Shailesh standing with his back towards Vikram will be facing South.
2. One evening before sunset two friends Sumit and Mohit were talking to each other face to face. If Mohit's shadow was exactly to his right side, which direction was Sumit facing?

A. North B. South C. West D. Data inadequate E. None of these

Answer: Option B
Explanation: In the evening, the sun is in the west and so the shadows fall towards the east. Now, since Mohit's shadow fell towards the right, therefore, Mohit is facing North. So, Sumit standing face to face with Mohit was facing South.
3. A girl leaves from her home. She first walks 30 m in the North-west direction and then 30 m in South-west direction. Next, she walks 30 m in South-east direction. Finally, she turns towards her house. In which direction is she moving?

A. North-east B. North-west C. South-east D. South-west E. None of these

Answer: Option A
Explanation: The movements of the girl are as shown in figure A to B, B to C, C to D, D to A). Clearly, she is finally moving in the direction DA i.e., North-east.

Dictionary Words
1. Which of the following will come forth in the English dictionary?

A. BARROW B. BARREN C. BELCH D. BARK

Answer: Option C
Explanation: A comes in the dictionary before E. So word starting with BA are followed by the one starting with BE. The first word in the alphabet dictionary will be BARK. The second word will be BARREN. The third word will be BARROW. The fourth word will be BELCH.
2. Which of the given responses would be a dictionary order of the following? 1) Prescription 2) Prescriber 3) Prescutum 4) Prescript

A. (4), (2), (1), (3) B. (2), (4), (1), (3) C. (2), (1), (4), (3) D. (1), (4), (2), (3)

Answer: Option B
Explanation: The given words can be arranged according to the dictionary order as follows: 2) Prescriber, 4) Prescript, 1) Prescription, 3) Prescutum So, the order will be: (2), (4), (1), (3)
3. Which of the following will come third in the English dictionary?

A. RAIN B. READ C. READY D. RAINBOW

Answer: Option B
Explanation: The first word in the dictionary will be RAIN. The second word in the dictionary will be RAINBOW as the first four letters are same and then letter B comes which comes next in the dictionary series. The third word in the dictionary will be READas E from the word read comes next in the series as compared to A in the word RAINBOW. So, READ is the third word which comes in theEnglish dictionary.
Tallest, Youngest Relation
1. There are five friends --- Sachin, Kunal, Mohit, Anuj, and Rohan. Sachin is shorter than Kunal but taller than Rohan. Mohit is the tallest. Anuj is a little shorter than Kunal and little taller than Sachin. If they stand in the order of increasing heights, who will be the second?

A. Anuj B. Sachin C. Rohan D. Kunal E. None of these

Answer: Option B.
Explanation: Let us denote the five boys by the first letter of their names, namely S, K, M, A, and R. Then, R < S < K < M and S < A < K. R < S < A < K < M.
2. In the order of increasing heights i.e. shortest to tallest, Sachin is second. (i). A, B, C, D, and E are five friends. (ii). B is elder to E, but not as tall as C. (iii). C is younger to A and is taller to D and E. (iv). A is taller to D but younger to E. (v). D is elder to A but is shortest in the group. If a selection is to be made among them who would be relatively older and also taller, who among them should be chosen?

A. A B. B C. C D. D E. E

Answer: Option B.
Explanation: In terms of age, we have : E < B, C < A, A < E, A < D So, we have : C < A < E < B < A < D. In terms of height, we have : B < C, D < C, E < C, D < A A and C are youngest so they cannot be selected. D is shorter than two persons A and C. B is shorter than C only and is also relatively older. So, B will be selected. E is younger than B.
3. (i). Six friends P, Q, R, S, T, and U are members of a club and play a different game of Football, Cricket, Tennis, Basketball, Badminton, and Volleyball. (ii). T who is taller than P and S plays Tennis. (iii). The tallest among them play Basketball. (iv). The shorter among them play Volleyball. (v). Q and S neither play Volleyball nor Basketball. (vi). R plays Volleyball. (vii). T is between Q who plays Football and P in order of height. Who among them is taller than R but shorter than P?

A. Q B. T C. U D. Data inadequate E. None of these

Answer: Option E.
Explanation: In terms of height, we have : T > P, T > S, Q > T > P. R plays Volleyball, so R is the shortest. Q plays neither Volleyball nor Basketball. So, Q is not the tallest. Thus, U is the tallest. So, the sequence becomes : U > Q > T > P > S > R. Now, T plays Tennis, U, being tallest, plays Basketball. R plays Volleyball. Q plays Football. Both P and S play either Cricket or Badminton. S is taller than R but shorter than P.
Number Ranking & Time Sequence Test
1. If 3rd December, 1990 is Sunday, what day is 3rd January, 1991?

A. Tuesday B. Wednesday C. Thursday D. Friday

Answer: Option B
Explanation: Clearly, 3rd, 10th, 17th, 24th, 31st December in 1990 are Sundays. So, 1st January 1991 is Monday and 3rd January in 1991 is Wednesday.
2. If the first day of the year(other than the leap year) was Friday, then which was the last day of the year?

A. Monday B. Friday C. Saturday D. Sunday

Answer: Option B
Explanation: If the year is not a leap year, then the last day of the year is the same as the first day.
3. How many days will there be from 26th January 1996 to 15th May 1996(both days included)?

A. 110 B. 111 C. 112 D. 113 E. None of these

Answer: Option B
Explanation: Number of days = (6 + 29 + 31 + 30 + 15) = 111. Note: 1988 is a leap year. So, a number of days in February = 29.
Coding and Decoding
1. In a certain code language if the word SPLENDOR is coded as UFNJPHQJ, then how is the word DISASTER coded in that language ?

A. FRUBUNGJ B. HRUDNPJL C. FRUBNPLH D. HRDLJNLJ E. FRDBNPLH

Answer: Option A
Explanation: Word: S P L E N D O R Pattern: +2 *2 +2 *2 +2 *2 +2 *2 Code: U F N J P H Q J Similarly, the code for DISASTER is FRUBUNGJ.
2. In a certain code language, if the word CERTIFY is coded as BURGIVX, then how is the word ADJACENT coded in that language?

A. ZWQZXVMG B. GMVXZQWZ C. RMVWYJWH D. GMXVWRVZ E. RMKLPRNG

Answer: Option B
Explanation: Word: C E R T I F Y Pattern: The letters in the word are reversed and then their opposite pairs are given as their codes. Code : B U R G I V X Similarly, the code for ADJACENT is GMVXZQWZ.
3. In a certain code language, if the word MISTERY is coded as ZLFGREL, then how is the word PURSUE coded in that language?

A. KFIHFV B. CHFEIR C. CHEFHR D. CHEGIR E. CHIRVS

Answer: Option C
Explanation: Word: M Y S T E R Y Pattern: To each of these letters their corresponding letters from the other half is given. Code: Z L F G R E L Similarly, the code for PURSUE is CHEFHR.
Assign Artificial Values to Mathematical Digit
1. 13. If + means x, ÷ means –, x means ÷ and means +, then— 5 + 12 ÷ 7 – 44 x 2 = ?

A. 75 B. 89 C. 65 D. 82 E. None of these

Answer: Option A
2. If + means ÷, ÷ means –, – means x and x means +, then— 9 + 3 ÷ 5 – 3 x 7 = ?

A. 5 B. 15 C. 25 D. 10 E. None of these

Answer: Option E
3. If 'a' means '÷', 'b' means '+', 'c' means '–' and 'd' means 'x'. then— 11 b 15 c 8 a 4 d 5 = ?

A. 36 B. – 16 C. 26 D. 16 E. None of these

Answer: Option D

Simple Interest
1. A certain sum is invested at simple interest at 18% p.a. for two years instead of investing at 12% p.a. for the same time period. Therefore the interest received is more by Rs. 840. Find the sum?

A. Rs. 7000 B. Rs. 8500 C. Rs. 8000 D. Rs. 7500 E. None of these

Answer: Option A
Explanation: Let the sum be Rs. x. (x * 18 * 2)/100 - (x * 12 * 2)/100 = 840 => 36x/100 - 24x/100 =840 => 12x/100 = 840 => x = 7000.
2. Vijay lent out an amount Rs. 10000 into two parts, one at 8% p.a. and the remaining at 10% p.a. both on simple interest. At the end of the year he received Rs. 890 as total interest. What was the amount he lent out at 8% pa.a?

A. Rs. 6000 B. Rs. 5500 C. Rs. 4500 D. Rs. 5000 E. None of these

Answer: Option B
Explanation: Let the amount lent out at 8% p.a. be Rs. A => (A * 8)/100 + [(10000 - A) * 10]/100 = 890 => A = Rs. 5500.
Height and Distance
1. The actual height of a man is 6 feet but due to the position of Sun he casts a shadow of 4 feet. He is standing next to an electricity tower and notices that it casts a shadow of 36 feet. Find the height of the electricity tower.

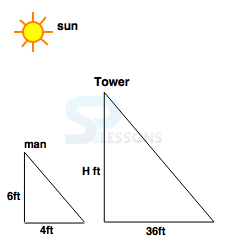
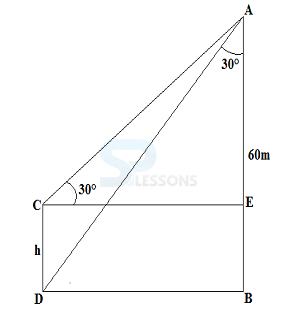
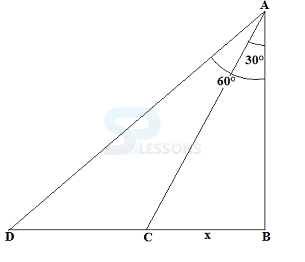
A. 48 ft B. 54 ft C. 63 ft D. 72 ft

Answer: Option B
Explanation: Both the man and tower are near each other and are illuminated by the same sun from the same direction.
So the angle of elevation for the sun is the same for both. So the ratio of object to shadow will be same for all objects. (Proportionality Rule) [latex]\frac {Object height}{Shadow length}[/latex]=[latex]\frac {6}{4}[/latex]=[latex]\frac {H}{36}[/latex] ∴ H = 54 ft = Height of tower.
2. On the two sides of a road are two tall buildings exactly opposite to each other. The height of the taller building is 60 m. If the angle of elevation from the top of the smaller building to the top of the taller one is 30° and the angle of depression from the top of the taller building to the foot of the smaller one is 30°, then find the height of the smaller building.

A. 45 B. 30 C. 40 D. 20

Answer: Option C
Explanation:
Let AB be the taller building of height 60m and CD be the smaller one of height h m. tan30= DB/AB = DB/60 = 1/[latex]\sqrt{3}[/latex] DB = 34.64 m tan30= AE/CE = AE/DB = 1/[latex]\sqrt{3}[/latex] = AE/34.64 AE = 60 – h = 20 h = 40 m
3. A man standing on the terrace of a building watches a car speeding towards him. If at that particular instant the car is 200 m away from the building makes an angle of depression of 60° with the man’s eye and after 8 seconds the angle of depression is 30°, what is the speed of the car?

A. 15 m/s B. 16.67 m/s C. 25 m/s D. Cannot be determined

Answer: Option B
Explanation:
Let AB be the building and the man is standing at A. When the car is 200m away from the building, the angle of depression is 60° tan60 = BD/AB = [latex]\sqrt {3}[/latex] 200/AB = [latex]\sqrt {3}[/latex] AB = 115.47 m tan30° =BC/AB = x/115.47 = 1/[latex]\sqrt {3}[/latex] x = 66.67 Now, the car travels distance CD in 8 seconds CD = BD – BC = 200 – 66.67 = 133.33 m Speed = 133.33/8 = 16.67 m/s
Volume and Surface Area
1. If the length, breadth and the height of a cuboid are in the ratio 6: 5: 4 and if the total surface area is 33300 cm[latex]^{2}[/latex], then length breadth and height in cms, are respectively?

A. 90, 85, 60 B. 85, 75, 60 C. 90, 75, 70 D. 90, 75,60

Answer: Option D
Explanation: Length = 6x Breadth = 5x Height = 4x in cm Therefore, 2(6x × 5x + 5x × 4x + 6x × 4x) = 33300 148x[latex]^{2}[/latex] = 33300 => x[latex]^{2}[/latex] = 33300/148 = 225 => x = 15. Therefore, Length = 90cm, Breadth = 75cm, Height = 60cm 90, 75 , 60 cm
2. A wooden box of dimensions 8m x 7m x 6m is to carry rectangular boxes of dimensions 8cm x 7cm x 6cm. The maximum number of boxes that can be carried in 1 wooden box is?

A. 1200000 B. 1000000 C. 9800000 D. 7500000

Answer: Option B
Explanation: Number of Boxes = [latex]\frac {volume of wooden box in cm ^{3}}{volume of 1 small box}[/latex] =[latex]\frac {800 \times 700 \times 600}{8 \times 7 \times 6}[/latex] = 1000000
Logarithm
1. If log 27 = 1.431, then the value of log 9 is:

A. 0.934 B. 0.945 C. 0.954 D. 0.958

Answer: Option C
Explanation: log 27 = 1.431 log (3[latex]^{3}[/latex] ) = 1.431 3 log 3 = 1.431 log 3 = 0.477 log 9 = log(3[latex]^{2}[/latex] ) = 2 log 3 = (2 x 0.477) = 0.954.
2. If [latex]log_{10}[/latex] 2 = 0.3010, then [latex]log_{2}[/latex] 10 is equal to:

A. [latex]\frac {699}{301}[/latex] B. [latex]\frac {1000}{301}[/latex] C. 0.3010 D. 0.6990

Answer: Option B
Explanation: [latex]log_{2}[/latex] 10 = [latex]\frac {1}{log_{10} 2}[/latex] = [latex]\frac {1}{0.3010}[/latex] = [latex]\frac {10000}{3010}[/latex] = [latex]\frac {1000}{301}[/latex].
Races and Games
1. A can run 22.5 m while B runs 25 m. In a kilometre race B beats A by:

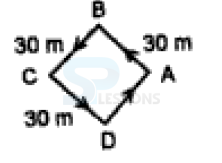
A. 100 m B. 75 m C. 25 m D. 50m

Answer: Option A
Explanation: When B runs 25 m, A runs 45/2 m When B runs 1000 m, A runs (45/2*1/25*1000)m = 900 m. B beats A by 100 m.
2. In a race of 1000 meters, A can beat B by 100 meters, in a race of 800 meters, B can beat C by 100 meters. By how many meters will A beat C in a race of 600 meters?

A. 125.5 meters B. 126.5 meters C. 127.5 meters D. 128.5 meters

Answer: Option C
Explanation: When A runs 1000 meters, B runs 900 meters and when B runs 800 meters, C runs 700 meters. Therefore, when B runs 900 meters, the distance that C runs = (900 x 700)/800 = 6300/8 = 787.5 meters. So, in a race of 1000 meters, A beats C by (1000 - 787.5) = 212.5 meters to C. So, in a race of 600 meters, the number of meters by Which A beats C = (600 x 212.5)/1000 = 127.5 meters.
Simplification
1. Find the value of (25 × (10 + 5) – 15) ÷ 62

A. 20 B. 10 C. 60 D. 0 E. None of these

Answer: Option B
Explanation: Follow BODMAS rule to solve this question, as per the order is given below, Step-1- Parts of an equation enclosed in 'Brackets' must be solved first, and in the bracket, the BODMAS rule must be followed, Step-2- Any mathematical 'Of' or 'Exponent' must be solved next, Step-3-Next, the parts of the equation that contains 'Division' and 'Multiplication' are calculated, Step-4-Last but not least, the parts of the equation that contains 'Addition' and 'Subtraction' should be calculated. The given expression is, (25 × (10 + 5) – 15) ÷ 6[latex]^ {2}[/latex] = (25 × 15 – 15) ÷ 6[latex]^ {2}[/latex] = (375 - 15) ÷ 6[latex]^ {2}[/latex] = 360 ÷ 6[latex]^ {2}[/latex] = 360 ÷ 36 = 10
2. What approximate value will come in place of question mark in the following question? 23.003 × 22.998 + 100.010 =?

A. 630 B. 720 C. 840 D. 520 E. 900

Answer: Option A
Explanation: Solve the given expression taking the nearest approximate value (round off) of the numbers given in the expression – ? = 23.003 × 22.998 + 100.010 ⇒ ? ≈ 23 × 23 + 100 = 629 ⇒ ? ≈ 630
Time and Distance
1. A child goes to school on bike at a speed of 18 km/h. While coming back, he take school bus which runs faster by 27 km/h. What is his average speed for the entire journey?

A. 25.71 km/hr B. 27 km/hr C. 27.39 km/hr D. 31.5km/hr

Answer: Option A
Explanation: Speed = S = [latex]\frac {D}{T}[/latex] Average Speed = [latex]\frac {Total distance traveled}{Total time taken}[/latex] Let the distance between start and end be D Total distance travelled = D+D = 2D The bus runs 27 kmph faster, so its speed is 45 kmph. Total Time = t1+t2 =[latex]\frac {d1}{s1}[/latex] + [latex]\frac {d2}{s2}[/latex] = [latex]\frac {D}{18}[/latex]+[latex]\frac {D}{45}[/latex] ∴ Average speed =[latex]\frac {2D}{D/18 + D/45}[/latex] = 25.71 km/hr
2. In how much time will a train of length 100 m, moving at 36 kmph cross an electric pole?

A. 15 sec B. 22 sec C. 18 sec D. 10 sec

Answer: Option D
Explanation: Convert kmph to mps. 36 kmph = 36 * 5/18 = 10 mps. The distance to be covered is equal to the length of the train. Required time t = d/s = 100/10 = 10 sec.
Chain Rule
1. A trip was organized for 75 travelers for 35 days and the food supply was arranged accordingly. However, some travelers showed up with friends and there were 15 additional people from day one of the trip. Now, for how many days will the food supply last for all these people?

A. 11.25 days B. 29.1 days C. 30 days D. 32 days

Answer: Option B
Explanation: Total people after guests come = 75+15 = 90 75 people x 35 days x 1 = 90 people x ? days x 1 ∴ ? = 29.1 days = Supplies will last for these many days.
2. 3 men can build a stage in 18 days while 6 boys can also do it in 18 days. If 4 men and 4 boys work on the assignment together, how many days will they take to finish it?

A. 4.5 days B. 6 days C. 9 days D. 27 days

Answer: Option C
Explanation: Men = M; Days = D; Time/Hours = T; Work = W M1D1T1W2 = M2D2T2W1 Note that - W2 is on left side and W1 is on right side To complete work in 18 days we need either 3 men or 6 boys. ∴ 1 man = 2 boys Take work done = 1 ∴ 3 men x 18 days x 1 = (4 men + 4 boys) x ? days x 1 Remember - Convert either all men to boys or all boys to men. ∴ 6 boys x 18 days x 1 = (8 boys + 4 boys) x ? days x 1 ∴ ? = 9 days = they will need these many days.
3. A tank has water for 72 trees and can last for 54 days for them. If each tree is given 10% less water than 90 trees can get the water for how many days?

A. 24 days B. 36 days C. 42 days D. 48 days

Answer: Option D
Explanation: Let each tree take T amount of water every day. So 72 trees take 72T water in one day. With 10% reduction, each tree will consume[latex]\frac {90T}{100}[/latex]amount each day So 90 Trees take 90[latex]\frac {90T}{100}[/latex]amount in one day Total water quantity is constant ∴ 72T x 54 = 90 x [latex]\frac {90T}{100}[/latex] x D ∴ D = 48 days = Number of days 90 trees can use the water.
Permutation and Combination
1. A question paper had 10 questions. Each question could only be answered as true(T) of False(F). Each candidate answered all the questions, Yet no two candidates wrote the answers in an identical sequence. How many different sequences of answers are possible?

A. 20 B. 40 C. 512 D. 1024

Answer: Option D
Explanation: Each question can be answered in 2 ways. 10 Questions can be answered = 2[latex]^ {10}[/latex]= 1024 ways.
2. In how many different ways can six players be arranged in a line such that two of them, Ajeet and Mukherjee are never together?

A. 120 B. 240 C. 360 D. 480

Answer: Option D
Explanation: As there are six players, So total ways in which they can be arranged = 6!ways =720. A number of ways in which Ajeet and Mukherjee are together = 5!x2 = 240. Therefore, Number of ways when they don’t remain together = 720 -240 =480.
Surds and Indices
1. Simplify : ([latex]y^ {4b-a }[/latex]. [latex]y^ {4c-b}[/latex] . [latex]y^ {4a-c}[/latex]) / [latex](y^ {a}. y^ {b}. y^ {c})^{3}[/latex]

A. 0 B. 1 C. y D. y[latex]^ {2}[/latex] E. None of these

Answer: Option B
Explanation: ([latex]y^ {4b-a }[/latex]. [latex]y^ {4c-b}[/latex] . [latex]y^ {4a-c}[/latex]) / [latex](y^ {a}. y^ {b}. y^ {c})^{3}[/latex] =([latex]y^ {4b-a }[/latex]. [latex]y^ {4c-b}[/latex] . [latex]y^ {4a-c}[/latex]) / [latex](y^ {a + b + c} )^{3}[/latex] =([latex]y^ {4b-a+4c-b+4a-c}[/latex] / [latex]y ^{3(a + b + c)}[/latex] =[latex]y ^{3(a + b + c)}[/latex] / [latex]y ^{3(a + b + c)}[/latex] = 1.
2. If 3[latex]^ {4m+1}[/latex] = 3[latex]^ {7m-5}[/latex], solve for m.

A. 2 B. 3 C. 1/3 D. 1/2 E. None of these

Answer: Option A
Explanation: 3[latex]^ {4m+1}[/latex] = 3[latex]^ {7m-5}[/latex] equating powers of 3 on both sides, 4m+1 = 7m - 5 => 3m = 6 => m = 2.
3. Identify the greatest numbers: 4[latex]^ {50}[/latex], 2[latex]^ {100}[/latex], 16[latex]^ {25}[/latex]

A. 4[latex]^ {50}[/latex] B. 2[latex]^ {100}[/latex] C. 16[latex]^ {25}[/latex] D. All are equal E. 2[latex]^ {100}[/latex], 16[latex]^ {25}[/latex]

Answer: Option D
Explanation: 4[latex]^ {50}[/latex] = [latex](2 ^{(2)50})[/latex] = 2[latex]^ {100}[/latex] => 16[latex]^ {25}[/latex] = [latex](2 ^{(4)25})[/latex] = 2[latex]^ {100}[/latex] Hence, 4[latex]^ {50}[/latex], 2[latex]^ {100}[/latex] and 16[latex]^ {25}[/latex]are all equal.
Pipes and Cistern
1. A tank is filled by three pipes with uniform flow. The first two pipes operating simultaneously fill the tank in the same during which the tank is filled by the third pipe alone. The second pipe fills the tank 5 hours faster than the first pipe and 4 hours slower than the third pipe. The time required by the first pipe is?

A. 6 hrs B. 10 hrs C. 15 hrs D. 30 hrs

Answer: Option C
Explanation: Suppose, first pipe alone takes x hours to fill the tank. Then, second and third pipes will take (x - 5) and (x - 9) hours respectively to fill the tank. 1/x + 1/(x - 5) = 1/(x - 9) (2x - 5)(x - 9) = x(x - 5) x2 - 18x + 45 = 0 (x- 15)(x - 3) = 0 => x = 15
2. Three taps A, B, and C can fill a tank in 12, 15 and 20 hours respectively. If A is open all the time and B and C are open for one hour each alternately, the tank will be full is?

A. 6 hrs B. 6 2/3 hrs C. 7 hrs D. 7 1/2 hrs

Answer: Option C
Explanation: (A + B)'s 1 hour work = (1/12 + 1/15) = 3/20 (A + C)'s 1 hour work = (1/12 + 1/20) = 2/15 Part filled in 2 hrs = (3/20 + 2/15) = 17/60 Part filled in 6 hrs = 3 * 17/60 = 17/20 Remaining part = 1 - 17/20 = 3/20 Now, it is the turn of A and B and 3/20 part is filled by A and B in 1 hour. Total time taken to fill the tank = (6 + 1) = 7 hrs.
3. One top can fill a cistern in 2 hours and another can empty the cistern in 3 hours. How long will they take to fill the cistern if both the taps are opened?

A. 5 Hours B. 6 Hours C. 7 Hours D. 8 Hours

Answer: Option B
Explanation: Net filling in 1 hour = (1/2 – 1/3) = 1/6 Time is taken to fill the cistern = 6 hours
Boats and Streams
1. A boat covers a distance of 30 km in 2 ½ hours running downstream while returning it covers the same distance in 3 ¾ hours. What is the speed of the boat in still water?

A. 8km/hr B. 12km/hr C. 14 km/hr D. 15 km/hr E. None of these

Answer: Option E
Explanation: Speed downstream = (30 x 2/5)km/hr = 12km/hr Speed upstream = (30 x 4/15) km/hr = 8 km/hr Speed of boat in still water = [latex]\frac {1}{2}[/latex] (12 + 8)km/hr = 10 km/hr
2. The speed of a motorboat is that of the current of water as 36:5. The boat goes along with the current in 5 hours 10 min, it will come back in?

A. 5hrs 50 min B. 6 hours C. 6 hours 50 min D. 12 hours 10 min

Answer: Option C
Explanation: Let the speed of motorboat be 36x km/hr and that of the current of water be 5x km/hr Speed downstream = (36x +5x)km/hr = 41x km/hr Speed upstream = (36 -5x)km/hr = 31x km/hr Distance covered downstream = (41x × 31/6)km Distance upstream = [1271x/6 × 1/31x]hrs = 41/6 hrs = 6hrs 50 min
3. A boat goes 6 km in an hour in still water. It takes thrice as much time in covering the same distance against the current. The speed of the current is?

A. 2 km/hr B. 3km/hr C. 4km/hr D. 5 km/hr

Answer: Option C
Explanation: Speed in still water = 6 km/hr Speed against the current = 6/3 km/hr = 2 km/hr Let the speed of the current be x km/hr 6-x = 2 => x =4 km/hr
Numbers
1. The sum of the two digits of a number is 10. If the number is subtracted from the number obtained by reversing its digits, the result is 54. Find the number?

A. 34 B. 28 C. 12 D. 17

Answer: Option B
Explanation: Any two digit number can be written as (10P + Q), where P is the digit in the tens place and Q is the digit in the units place. P + Q = 10 ----- (1) (10Q + P) - (10P + Q) = 54 9(Q - P) = 54 (Q - P) = 6 ----- (2) Solve (1) and (2) P = 2 and Q = 8 The required number is = 28
2. The numerator of a certain fraction is 8 less than the denominator. If 3 is added to the numerator and 3 is subtracted from the denominator, the fraction becomes 3/4. Find the original fraction?

A. 2/5 B. 7/9 C. 3/11 D. 8/5

Answer: Option C
Explanation: The denominator is P, the numerator will be (P - 8). The fraction will be (P - 8)/P. Adding 3 to the numerator and subtracting 3 from the denominator, (P - 8 + 3)/(P - 3) = 3/4. (P - 5)/(P - 3) = 3/4 P = 20 - 9 => P = 11. The fraction is 3/11.
Partnership
1. Three friends M, N and O stay in a business for 2 months, 5 months and 8 months respectively. They share the profit in the ration 5 : 8 : 9 respectively. Find the ratio of their investment.

A. 7 : 13 : 17 B. 10 : 40 : 72 C. 100 : 64 : 45 D. 110 : 74 : 64

Answer: Option C
Explanation: Since they spend 2 months, 5 months and 8 months in the business respectively, their time period in business is in the ratio of 2: 5: 8. The ratio of investment for M, N, and O is found by =[latex]\frac {5}{2}[/latex]: [latex]\frac {8}{5}[/latex]: [latex]\frac {9}{8}[/latex] Making denominators common by multiplying by 40 ∴ Ratio of Time periods for M, N, and O = [latex]\frac {40 x\times 5}{2}[/latex] : [latex]\frac {40 \times 8}{5}[/latex]: [latex]\frac {40 \times 9}{8}[/latex] = 100 : 64 : 45
2. Armaan and Gubbi started a cafe with Rs. 40000 and Rs. 80000, respectively. Gubbi got married to someone in other town and left after 7 months. But Jayaram immediately replaced her with an investment of Rs. 144000. At the end of the year, the business performed well and registered a profit of Rs. 50600. What is Gubbi’s share in this profit?

A. Rs. 13800 B. Rs. 16100 C. Rs. 16500 D. Rs. 16866.67

Answer: Option B
Explanation: The ratio of Investment x Time = Ratio of Profit ∴ (A's investment x Time) : (B's investment x Time) = Profit of A : Profit of B Total value of investment of Arman, Gubbi and Jayram after 12 months is = Rs. 40000 x 12 months : Rs. 80000 x 7 months : Rs. 144000 x (12-7)months = 480000 : 560000 : 720000 ∴ Profit ratio = 240000 : 280000 : 360000 = 6 : 7 : 9 ∴ Share of Gubbi = [latex]\frac {7}{6+7+9}[/latex] x 50600 = Rs. 16100.
Ratio and Proportion
1. 1600 men have provisions for 28 days in the temple. If after 4 days, 400 men leave the temple, how long will the food last now?

A. 28 days B. 30 days C. 32 days D. 35 days

Answer: Option C
Explanation: 1600 ---- 28 days 1600 ---- 24 1200----? 1600*24 = 1200*x x = 32 days
2. There is food for 760 men for 22 days. How many more men should join after two days so that the same food may last for 19 days more?

A. 60 men B. 40 men C. 25 men D. 20 men

Answer: Option B
Explanation: 760 ---- 22 760 ---- 20 x ----- 19 x*19 = 760*20 = 800 x = 800 - 760 = 40
Problems on H.C.F and L.C.M.
1. Find the greatest 4-digit number exactly divisible by 3, 4 and 5?

A. 9985 B. 9960 C. 9957 D. 9975

Answer: Option B
Explanation: The greatest 4-digit number is 9999. LCM of 3, 4 and 5 is 60. Dividing 9999 with 60, we get remainder 39. Thus, the required number is 9999 - 39 = 9960.
2. Find the greatest number which, while dividing 19, 83 and 67, gives a remainder of 3 in each case?

A. 16 B. 17 C. 18 D. 19

Answer: Option A
Explanation: Subtract the remainder 3 from each of the given numbers: (19-3)=16, (67-3)=64 and (83-3)=80. Now, find the HCF of the results 16, 64 and 80, we get 16. Thus, the greatest number is 16.
Banker’s Discount
1. The banker's discount of a certain sum of money is Rs. 72 and the true discount on the same sum for the same time is Rs. 60. The sum due is:

A. Rs. 360 B. Rs. 432 C. Rs. 540 D. Rs. 1080

Answer: Option A
Explanation: Sum =[latex]\frac {B.D. \times T.D.}{B.D. - T.D.}[/latex] = Rs. [latex]\frac {72 \times 60}{72 - 60}[/latex] = Rs. [latex]\frac {72 \times 60}{12}[/latex] = Rs. 360.
2. The certain worth of a certain sum due sometime hence is Rs. 1600 and the true discount is Rs. 160. The banker's gain is:

A. Rs. 20 B. Rs. 24 C. Rs. 16 D. Rs. 12

Answer: Option C
Explanation: B.G. =[latex]\frac {(T.D.)^{2}}{P.W.}[/latex] = Rs. [latex]\frac {160 \times 160}{1600}[/latex] = Rs. 16.
3. The present worth of a certain bill due sometime hence is Rs. 800 and the true discount is Rs. 36. The banker's discount is:

A. Rs. 37 B. Rs. 37.62 C. Rs. 34.38 D. Rs. 38.98

Answer: Option B
Explanation: B.G. =[latex]\frac {(T.D.)^{2}}{P.W.}[/latex] = Rs. [latex]\frac {36 \times 36}{800}[/latex] = Rs. 1.62 B.D. = (T.D. + B.G.) = Rs. (36 + 1.62) = Rs. 37.62
Compound Interest
1. Rohit says that, an amount became 4 times in 6 years. His friend wanted to know, how many years will it take for the amount to become 64 times, provided the rate of interest remains same?

A. 12 years B. 16 years C. 18 years D. 24 years

Answer: Option C
Explanation: Initial Amount = P. P becomes 4 times in 6 years ∴ P → 4P in 6 years ∴ 4P → 4 x (4P) = 16P in next 6 years. Continuing this, 16P → 4 x (16P) = 64P in next 6 years. So total time to get to 64 times i.e. 64P = 6+6+6 = 18 years.
2. A sum of Rs.4800 is invested at compound interest for three years, the rate of interest is 10% p.a., 20% p.a. and 25% p.a. for the 1st, 2nd and the 3rd years respectively. Find the interest received at the end of the three years.

A. Rs.2520 B. Rs.3120 C. Rs.3320 D. Rs.2760 E. None of these

Answer: Option B
Explanation: Let A be the amount received at the end of the three years. A = 4800[1 + 10/100][1 + 20/100][1 + 25/100] A = (4800 * 11 * 6 * 5)/(10 * 5 * 4) A = Rs.7920 So the interest = 7920 - 4800 = Rs.3120
Area
1. The perimeter of a semi circle is 144 cm then the radius is?

A. 25 cm B. 28 cm C. 30 cm D. 35 cm

Answer: Option B
Explanation: 36/7 r = 144 => r = 28
2. The Length of a plot is four times is breadth. A playground measuring 1200 square meters occupies a third of the total area of the plot. What is the length of the plot in meters?

A. 20 B. 30 C. 60 D. None of these

Answer: Option D
Explanation: Area of the plot = (3x1200)Sq.m = 3600 Sq.m Breadth = x meters and length = 4x meters 4x × x = 3600 or x[latex]^ {2}[/latex] = 900 0r x = 30 Length of plot = 4x = (4×30) m = 120 m
Time and Work
1. A can do a half of certain work in 70 days and B one third of the same in 35 days. They together will do the whole work in.

A. 420 days B. 120 days C. 105 days D. 60 days

Answer: Option D
Explanation: A = 140 days B = 105 days 1/140 + 1/105 = 7/420 = 1/60 =>60 days
2. Anita, Indu, and Geeta can do a piece of work in 18 days, 27 days and 36 days respectively. They start working together. After working for 4 days. Anita goes away and Indu leaves 7 days before the work is finished. Only Geeta remains at work from beginning to end. In how many days was the whole work is done?

A. 16 days B. 17 days C. 18 days D. 19 days

Answer: Option A
Explanation: 4/18 + (x -7)/27 + x/36 = 1 x = 16 days
Allegation or Mixture
1. A body massage oil mix contains 60 liters of Oil A with some liters of Oil B. The rate of Oil A is Rs. 32 per liter while the rate of Oil B is 9 Rs/liter less than Oil A. A shopkeeper sells this oil mix at Rs. 28/liter. How much Oil B should be mixed in the mixture to ensure that there is no profit, no loss in the whole process?

A. 24 litres B. 36 litres C. 48 litres D. 50 litres

Answer: Option C
Explanation: Let Oil B quantity be B liters. Final mixture has '60+B' litres There is no profit no loss So, (Oil 1 quantity * rate) + (Oil 2 quantity * rate) = Mix quantity * rate Oil B is 9 Rs/- cheap. So, the rate of Oil B per litre = 23 Rs/litre ∴ 60 x 32 + B x 23 = (60+B) x 28 ∴ B = 48 litres = Oil B quantity
2. Three vessels contain a milk mixture 30 liter each. When put in a big vessel, they result in a mixture of milk and water in the ratio 2:1. If the ratio is to be reversed to make it 1: 2, how much more water should be added to the mix?

A. 20L B. 40L C. 90L D. 100L

Answer: Option C
Explanation: 3 vessels of 30 litres each = 3 X 30 = 90 ml milk mixture Amount of milk in mixture = [latex]\frac {2}{2+1}[/latex] x 90 = 60L Amount of water = 90-60 = 30L To make milk to water ratio 1:2, we simply need to make water double of milk. By direct observation, we can say that we should have 60L x 2 = 120L water to make the required ratio We already have 30L, we need (120-30) = 90L more water.
Decimal Fraction
1. How many digits will be there to the right of the decimal point in the product of 89.635 and .02218?

A. 5 B. 6 C. 7 D. 8

Answer: Option C
Explanation: Sum of decimal places = 3 + 5 = 8 The last digit in the product(digit at the extreme right) is zero (Since 5 x 8 = 40) Hence, there will be 7 significant digits to the right of the decimal point.
2. 24.39 + 562.093 + 35.96 = ?

A. 622.441 B. 622.243 C. 622.233 D. 622.443

Answer: Option D
Explanation: 24.39 + 562.093 + 35.96 = 622.443
3. What decimal of an hour is a second?

A. 0.00027 B. 0.00025 C. 0.00026 D. 0.00024

Answer: Option A
Explanation: 1 hour = 60 minutes = 3600 seconds Hence, required decimal = 1/3600 = 0.00027
Probability
1. Ramesh throws two dices simultaneously. What is the probability that the sum of numbers on top faces in a throw is 9?

A. [latex]\frac {1}{12}[/latex] B. [latex]\frac {1}{9}[/latex] C. [latex]\frac {1}{3}[/latex] D. [latex]\frac {2}{9}[/latex]

Answer: Option B
2. India is playing 3 sets of badminton matches against Japan at Singapore. The probability of India winning the three sets is 1/7, 1/5, 3/4. What is the probability of winning at least 1 set of the game?

A. [latex]\frac {7}{24}[/latex] B. [latex]\frac {23}{24}[/latex] C. [latex]\frac {23}{35}[/latex] D. [latex]\frac {29}{35}[/latex]

Answer: Option D
3. If I roll two dices simultaneously, what is the probability of getting a sum less than 13?

A. [latex]\frac {1}{2}[/latex] B. 0.75 C. 1 D. 1.25

Answer: Option C
Average
1. Find the average price of the goods he has bought.

A. Rs. 5.5 B. Rs. 6.05 C. Rs. 8.25 D. Rs. 9

Answer: Option B
Explanation: Average =[latex]\frac {Sum of Observations}{Number of Observations}[/latex] Average = [latex]\frac {Total cost of pencils + Total cost of pens + Total cost of sharpeners}{Total pencils + Total pens + Total sharpeners}[/latex] ∴ Average price = [latex]\frac {(35 \times 4)+(30 \times 11)+(25 \times 3)}{35+30+25}[/latex] = Rs. 6.05
2. If I do not consider the earnings from selling the six seater dining table, my average falls by Rs 2000/-. What is the cost of six seater dining table if average earnings from selling 11 four-seater dining tables and one six-seater dining table are Rs 18000?

A. Rs. 29000 B. Rs. 30000 C. Rs. 36000 D. Rs. 40000

Answer: Option D
Explanation: Without six seater dining table, there are 11 four-seater dining tables. Average = 18000 - 2000 = 16000. Total cost of 11 small dining tables = 11 x 16000 = Rs. 176000 With big dining table, total cost of 12 tables = 12 x 18000 = Rs. 216000 Cost of six seater dining table = 216000 - 176000 = Rs. 40000
3. In an exam, the average marks for 80 students of Class V are 35. The average of marks in section A of the class is 55 while the average of marks in section B is 30. Find the number of students in Class V B.

A. 45 B. 50 C. 64 D. 70

Answer: Option C
Explanation: Total students i.e. Section A + Section B = 80 Section A = 80 - Section B = 80 - B ∴ 80 x 35 = B x 30 + (80 - B) x 55 2800 = 30B + 4400 - 55B 1600 = 25 B B = 64 ∴ B = 64 = Number of students in Class V B.
Stocks and Share
1. A man sells Rs.5000, 12 % stock at 156 and uinvests the proceeds parity in 8 % stock at 90 and 9 % stock at 108. He hereby increases his income by Rs. 70. How much of the proceeds were invested in eac

A. 4000 B. 4200 C. 4002 D. 4020

Answer: Option B
2. The cash realized on selling a 14% stock is Rs.106.25, brokerage being 1/4% is

A. 123 B. 106 C. 100 D. 156

Answer: Option B
3. A invested some money in 10% stock at 96. If B wants to invest in an equally good 12% stock, he must purchase a stock worth of :

A. Rs.80 B. Rs.115.20 C. Rs.120 D. Rs.125.40

Answer: Option B
Square Root and Cube Root
1. Find the smallest number by which 1780 must be added to make the sum a perfect square.

A. 46 B. 49 C. 69 D. 72

Answer: Option C
Explanation: Step 1: Find the square root of 1780 [latex]\sqrt {1780}[/latex] = 42.190 Take the square of the next number after 42 i.e. 43. Step 2: Find the least number Subtract given number from 43[latex]^{2}[/latex] 43[latex]^{2}[/latex] – 1780 = Number to be added 1849 – 1780 = 69 Hence, the number to be added is 69.
2. If [latex]\sqrt {5}[/latex] = 2.236, then find the value of [latex]\sqrt {320}[/latex] –[latex]\frac {1}{5}[/latex] [latex]\sqrt {125 }[/latex] – [latex]\sqrt {180}[/latex]

A. 4.236 B. 2.236 C. 3.346 D. 1.566

Answer: Option B
Explanation: = [latex]\sqrt {320}[/latex] – (1/5)[latex]\sqrt {125}[/latex] – [latex]\sqrt {180}[/latex] = [latex]\sqrt {64 \times 5 }[/latex] – (1/5) [latex]\sqrt {25 \times 5}[/latex] – [latex]\sqrt { 36 \times 5}[/latex] = 8[latex]\sqrt {5}[/latex] – (1/5)5[latex]\sqrt {5}[/latex] – 6[latex]\sqrt {5}[/latex] = 8[latex]\sqrt {5}[/latex] – (1 + 6)[latex]\sqrt {5}[/latex] = [latex]\sqrt {5}[/latex] Value of[latex]\sqrt {5}[/latex] = 2.236
3. Find the least number by which 1470 must be divided to get a number which is a perfect square.

A. 6 B. 9 C. 12 D. 30

Answer: Option D
Explanation: This numerical can be easily solved, if we solve it by considering the options. Divide 1470 by each option and find out the number which is a perfect square. [latex]\frac {1470}{6}[/latex] = 245, [latex]\frac {1470}{9}[/latex] = 163.33, [latex]\frac {1470}{12}[/latex]=122.5, [latex]\frac {1470}{30}[/latex] = 49 Hence, it is clear that the correct option is D = 30
Problems on Ages
1. A person was asked to state his age in years. His reply was, "Take my age three years hence, multiply it by 3 and then subtract three times my age three years ago and you will know how old I am." What was the age of the person?

A. 18 years B. 20 years C. 24 years D. 32 years

Answer: Option A
Explanation: Let the present age of the person be x years. Then, 3(x + 3) - 3(x - 3) = x 3x + 9 - 3x + 9 = x => x = 18
2. The sum of the ages of a son and father is 56 years after four years the age of the father will be three times that of the son. Their ages respectively are:

A. 12years, 44 years B. 16years, 42years C. 16years, 48years D. 18years, 36 years

Answer: Option A
Explanation: Present ages of son and father are x years (56 -x)years (56- x +4) = 3(x + 4) or 4x =48 or x = 12 Ages are 12 years, 44 years
3. In 10 years, A will be twice as old as B was 10 years ago. If A is now 9 years older than B, the present age of B is

A. 19 Years B. 29 Years C. 39 Years D. 49 Years

Answer: Option C
Explanation: Present ages of B and A be x years and (x +9) years (x +9 +10) = 2 (x -10) or x =39