Arithmetic
1. The ratio between the speeds of two trains is 7 : 8. If the second train runs 400 kms in 4 hours, then the speed of the first train is:

A. 70 km/hr B. 75 km/hr C. 84 km/hr D. 87.5 km/hr

Answer: Option (D)
Explanation: Let the speed of two trains be 7x and 8x km/hr. Then, 8x = 400/4 = 100 x = 100/8 = 12.5 Speed of first train = (7 x 12.5) km/hr = 87.5 km/hr.
2. A man on tour travels first 160 km at 64 km/hr and the next 160 km at 80 km/hr. The average speed for the first 320 km of the tour is:

A. 35.55 km/hr B. 36 km/hr C. 71.11 km/hr D. 71 km/hr

Answer: Option (C)
Explanation: Total time taken = (160/64 + 160/80 hrs. = 9/2 hrs. Average speed = (320 x 2/9) km/hr = 71.11 km/hr.
3. In covering a distance of 30 km, Celine takes 2 hours more than Sam. If Celine doubles his speed, then he would take 1 hour less than Sam. Celine's speed is:

A. 5 kmph B. 6 kmph C. 6.25 kmph D. 7.5 kmph

Answer: Option (A)
Explanation: Let Celine's speed be x km/hr. Then, 30/x - 30/2x = 3 6x = 30 x = 5 km/hr.
4. A farmer traveled a distance of 61 km in 9 hours. He travelled partly on foot @ 4 km/hr and partly on bicycle @ 9 km/hr. The distance traveled on foot is:

A. 14 km B. 15 km C. 16 km D. 17 km

Answer: Option (C)
Explanation: Let the distance traveled on foot be x km. Then, distance travelled on bicycle = (61 -x) km. So, x/4 + (61 -x)/9 = 9 9x + 4(61 -x) = 9 x 36 5x = 80 x = 16 km.
5. It takes eight hours for a 600 km journey if 120 km is done by train and the rest by car. It takes 20 minutes more if 200 km is done by train and the rest by car. The ratio of the speed of the train to that of the cars is:

A. 2: 3 B. 3: 2 C. 3: 4 D. 4: 3

Answer: Option (C)
Explanation: Let the speed of the train be 'x' km/hr and that of the car be 'y' km/hr. Then, 120/x + 480/y = 8 => 1/x + 4/y = 1/15......(i) And, 200/x + 400/y = 25/3 => 1/x + 2/y = 1/24.....(ii) solving (i) and (ii), we get : x = 60 and y = 80 The ratio of speeds = 60: 80 = 3 : 4.
Algebra
1. Find the greatest number that will divide 43, 91 and 183 so as to leave the same remainder in each case.

A. 4 B. 7 C. 9 D. 13

Answer: Option (A)
Explanation: Required number = H.C.F. of (91 - 43), (183 - 91) and (183 - 43) = H.C.F. of 48, 92 and 140 = 4.
2. The H.C.F. of two numbers is 23 and the other two factors of their L.C.M. are 13 and 14. The larger of the two numbers is:

A. 276 B. 299 C. 322 D. 345

Answer: Option (C)
Explanation: Clearly, the numbers are (23 x 13) and (23 x 14). Larger number = (23 x 14) = 322.
3. Six bells commence tolling together and toll at intervals of 2, 4, 6, 8 10 and 12 seconds respectively. In 30 minutes, how many times do they toll together?

A. 4 B. 10 C. 15 D. 16

Answer: Option (D)
Explanation: L.C.M. of 2, 4, 6, 8, 10, 12 is 120. So, the bells will toll together after every 120 seconds(2 minutes). In 30 minutes, they will toll together 30/2 + 1 = 16 times.
4. Let N be the greatest number that will divide 1305, 4665 and 6905, leaving the same remainder in each case. Then the sum of the digits in N is:

A. 4 B. 5 C. 6 D. 8

Answer: Option (A)
Explanation: N = H.C.F. of (4665 - 1305), (6905 - 4665) and (6905 - 1305) = H.C.F. of 3360, 2240 and 5600 = 1120. Sum of digits in N = ( 1 + 1 + 2 + 0 ) = 4.
5. Three number are in the ratio of 3: 4: 5 and their L.C.M. is 2400. Their H.C.F. is:

A. 40 B. 80 C. 120 D. 200

Answer: Option (A)
Explanation: Let the numbers be 3x, 4x and 5x. Then, their L.C.M. = 60x. So, 60x = 2400 or x = 40. The numbers are (3 x 40), (4 x 40) and (5 x 40). Hence, the required H.C.F. = 40.
Trigonometry
1. Find the simplest numerical value of 3 (sin x – cos x)4+ 4 (sin6x + cos6x) +6 (sin x + cos x)2

A. 12 B. 10 C. 21 D. 13

Answer: Option (D)
2. If cosq + secq = 2, then cos5q + sec5q= ?

A. 1 B. 2 C. –1 D. –2

Answer: Option (B)
3. 2 (sin6q + cos6q) – 3 (sin4q + cos4q) + 1 = ?

A. 1 B. 0 C. –1 D. 2

Answer: Option (B)
4. To a man standing at the midpoint of the line joining the feet of two vertical poles of the same height. the angle of elevation of the tip of each pole is 300. When the man advances a distance of 40 metres towards one pole, the angle of elevation of the tip of this pole is 60°. What is the distance between the two poles?

A. 120 metre B. 110 metre C. 130 metre D. 115 metre

Answer: Option (A)
5. [latex]\frac{sin A - sin B}{cos A + cos B}[/latex] + [latex]\frac{cos A - cos B}{sin A + sin B}[/latex]

A. 1 B. cos A C. sin A D. 0

Answer: Option (D)
Geometry
1. In any triangle PQR, PS is the internal bisector of ÐQPR and PT ^ QR then ÐTPS = ?

A. ÐQ – ÐR B. (ÐQ + ÐR) C. (ÐQ – ÐR) D. ÐQ + ÐR

Answer: Option (C)
2. PQRS is a cyclic quadrilateral. The bisectors of the angles ÐP and ÐR meet the circle ABCD at A and B respectively. If the radius of the circle be r units, then AB =?

A. r B. 2r C. 3r D. 4r

Answer: Option (B)
3. In D ABC, a line parallel to BC intersects AB and AC at D and E. If AE = 3 AD, find the ratio BD: EC.

A. 1: 3 B. 1: 2 C. 2: 3 D. 3: 2

Answer: Option (A)
4. Two circles whose radii are 10 cm and 8 cm, intersect each other and their common chord is 12 cm long. What is the distance between their centres?

A. 11.27 cm B. 12.29 cm C. 12.27 cm D. 13.29 cm

Answer: Option (D)
5. If the ratio of a number of sides of two regular polygons is 2 : 3 and the ratio of their interior angles be 6: 7, find the number of sides of the two polygons.

A. 6 and 7 B. 8 and 9 C. 6 and 9 D. 6 and 8

Answer: Option (C)
Mensuration
1. Find the area of a parallelogram with base 24 cm and height 16 cm.

A. 262 cm[latex]^{2}[/latex] B. 384 cm[latex]^{2}[/latex] C. 192 cm[latex]^{2}[/latex] D. 131 cm[latex]^{2}[/latex] E. None of these

Answer: Option (B)
Explanation: Area of a parallelogram = base * height = 24 * 16 = 384 cm[latex]^{2}[/latex]
2. The ratio of the length and the breadth of a rectangle is 4 : 3 and the area of the rectangle is 6912 sq cm. Find the ratio of the breadth and the area of the rectangle?

A. 1: 96 B. 1: 48 C. 1: 84 D. 1: 68 E. None of these

Answer: Option (A)
Explanation: Let the length and the breadth of the rectangle be 4x cm and 3x respectively. (4x)(3x) = 6912 12x[latex]^{2}[/latex] = 6912 x[latex]^{2}[/latex] = 576 = 4 * 144 = 2[latex]^{2}[/latex] * 12[latex]^{2}[/latex] (x > 0) => x = 2 * 12 = 24 Ratio of the breadth and the areas = 3x : 12x[latex]^{2}[/latex] = 1 : 4x = 1: 96.
3. An order was placed for the supply of a carpet whose breadth was 6 m and length was 1.44 times the breadth. What is the cost of a carpet whose length and breadth are 40% more and 25% more respectively than the first carpet? Given that the ratio of carpet is Rs. 45 per sq m?

A. Rs. 3642.40 B. Rs. 3868.80 C. Rs. 4216.20 D. Rs. 4082.40 E. None of these

Answer: Option (D)
Explanation: Length of the first carpet = (1.44)(6) = 8.64 cm Area of the second carpet = 8.64(1 + 40/100) 6 (1 + 25/100) = 51.84(1.4)(5/4) sq m = (12.96)(7) sq m Cost of the second carpet = (45)(12.96 * 7) = 315 (13 - 0.04) = 4095 - 12.6 = Rs. 4082.40
4. The area of a square is equal to five times the area of a rectangle of dimensions 125 cm * 64 cm. What is the perimeter of the square?

A. 600 cm B. 800 cm C. 400 cm D. 1000 cm E. None of these

Answer: Option (B)
Explanation: Area of the square = s * s = 5(125 * 64) => s = 25 * 8 = 200 cm Perimeter of the square = 4 * 200 = 800 cm.
5. A cube of side one meter length is cut into small cubes of side 10 cm each. How many such small cubes can be obtained?

A. 10 B. 100 C. 1000 D. 10000 E. None of these

Answer: Option (C)
Explanation: Along one edge, the number of small cubes that can be cut = 100/10 = 10 Along each edge 10 cubes can be cut. (Along length, breadth and height). Total number of small cubes that can be cut = 10 * 10 * 10 = 1000.
Statistics

1. What is the percentage change in the overall sales turnover of the five companies together between 2001 - 2002 and 2002 - 2003?

A. 17.21 % B. 14.68 % C. 12.67 % D. 21.24 %

Answer: Option (B)
Explanation: The required answer is 100 - percentage value of the fraction (Absolute change/first year's value).
2. What is the absolute change in overall sales turnover of the five companies together between 2001 - 2002 and 2002 - 2003?

A. 712.43 B. 142.48 C. 683.53 D. None of these

Answer: Option (A)
Explanation: The absolute value of the difference between the sum of the turnover of the five companies for 2001 - 2002 and 2002 - 2003.
3. Which of the companies shows the maximum percentage difference in sales turnover between the two years?

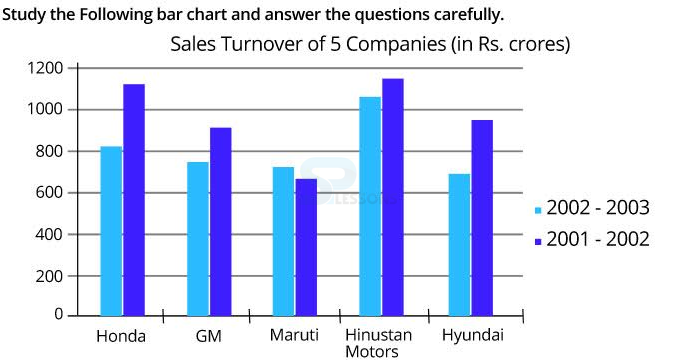
A. Honda B. GM C. Hyundai D. Maruti

Answer: Option (C)
Explanation: Hyundai with 25.25 % is marginally higher than Honda with 24.5 %.
4. What should have been the sales turnover of GM in 2002 - 2003 to have shown an excess of the same quantum over 2001 - 2002 as shown by the sales turnover of Maruti?

A. 953.76 B. 963.76 C. 952.76 D. 962.76

Answer: Option (D)
Explanation: GM should have increased its sales turnover by Rs.49.13 crore. Hence, the answer is 913.63 + 49.13 = 962.76.
5. What is the approximate difference between the average sales turnover of all the companies put together between the years 2001 - 2002 and 2002 - 2003?

A. 133.45 B. 142.48 C. 117.6 D. None of these

Answer: Option (B)
Explanation: Difference between the sum of the two years divided by 5.