Introduction
Introduction
 Pattern
Pattern
Scheme of Preliminary Examination for recruitment to the post of Upper Division Clerk (UDC) in ESIC is as follows:
- Preliminary Examination is an objective test.
- The test will have four sections.
- The overall test duration is for 1 hour (No separate sectional duration).
- The test contain 200 questions with Maximum of 200 marks.
- Phase –I Preliminary Examination is qualifying in nature and marks will not be reckoned for final merit.
- The candidates will be shortlisted for Phase-II in the ratio of 1:10 i.e. about 10 times the number of vacancies in each category on the basis of their performance in Phase - I.
| Name of the Test (Objective Tests) | No. of Qs. | Max. Marks | Duration | Version |
|---|---|---|---|---|
| General Intelligence and Reasoning | 25 | 50 | 1 hour | Bilingual |
| General Awareness | 25 | 50 | Bilingual | |
| Quantitative Aptitude | 25 | 50 | Bilingual | |
| English Comprehension | 25 | 50 | English | |
| Total | 100 | 200 |
 Syllabus
Syllabus
[Click Here] for ESIC UDC Quantitative Aptitude Syllabus
 Samples
Samples
Partnership
1. Sumit and Ravi started a business by investing Rs 85000 and 15000 respectively. In what ratio the profit earned after 2 years be divided between Sumit and Ravi respectively.
- A. 17:1
B. 17:2
C. 17:3
D. 17:4
- A. 10500, 13500, 19500
B. 10500, 13500, 18500
C. 10500, 13500, 17500
D. 10500, 13500, 16500
- A. 3:7:5
B. 6:10:5
C. 6:10:7
D. 6:7:5
- A. Rs 9600
B. Rs 8500
C. Rs 8450
D. Rs 8400
- A. 4
B. 5
C. 6
D. 7
- A. 1000
B. 1500
C. 2000
D. 2500
- A. 1%
B. 2%
C. 3%
D. 4%
- A. 8
B. 9
C. 10
D. 11
- A. 9%
B. 10%
C. 11%
D. 12%
- A. 750
B. 700
C. 650
D. 600
- A. 400
B. 410
C. 390
D. 380
- A. 0%
B. 5%
C. 7.5%
D. 10%
- A. Rs. 9025.20
B. Rs. 9200
C. Rs. 9600
D. Rs. 9560
- A. gains Rs. 55
B. gains Rs. 50
C. loses Rs. 30
D. gains Rs. 30
- A. Gopal gains Rs.3.33
B. Ajay gains Rs.3.33
C. Ajay loses Rs.16.67
D. Gopal loses Rs.16.67
- A. 36
B. 37
C. 38
D. 39
- A. 50
B. 55
C. 60
D. 65
- A. 35
B. 45
C. 55
D. 65
- A. 37
B. 37.8
C. 39
D. 39.8
- A. 40
B. 35
C. 45
D. 55
- A. 3 days
B. 4 days
C. 5 days
D. 6 days
- A. 19 days
B. 10 days
C. 20 days
D. 12 days
- A. Rs.600
B. Rs.450
C. Rs.300
D. Rs.500
- A. 21 days
B. 15 days
C. 12 days
D. 9 days
- A. 1:2
B. 2:1
C. 2:3
D. 6:5
- A. 120
B. 720
C. 4320
D. 2160
E. None of these
- A. 10080
B. 4989600
C. 120960
D. None of these
- A. 360
B. 480
C. 720
D. 5040
E. None of these
- A. 810
B. 1440
C. 2880
D. 50400
E. 5760
- A. 72
B. 144
C. 360
D. 720
E. None of these
- A. 40
B. 50
C. 60
D. 70
- A. 120, 130
B. 140,280
C. 240,280
D. 100,120
- A. 46
B. 48
C. 64
D. 84
- A. 4/12
B. 3/13
C. 5/12
D. 11/7
- A. 20
B. 50
C. 60
D. 40
- A. 3 : 9;
B. 6 : 10;
C. 9 : 21;
D. 18 : 49
- A. 5 : 7;
B. 7 : 12;
C. 5 : 12;
D. none of these
- A. 4: 3;
B. 3: 4;
C. 4: 5;
D. none of these
- A. 49;
B. 72;
C. 63;
D. 42
- A. 1 : 15;
B. 15 : 10;
C. 10 : 15;
E. 15 : 1
- A. 2
B. 3
C. 4
D. 5
- A. 2985400
B. 1492700
C. 7463500
D. 7483500
- A. 936
B. 39987
C. 2343
D. 36
- A. 415591
B. 6472
C. 3364
D. 413976
- A. 72
B. 72.5
C. 62.5
D. none of these
- A. 3/5
B. 3/4
C. 3/2
D. 3/7
- A. 7/1150
B. 7/1175
C. 7/1250
D. 7/1275
- A. 5/8 < 7/12 < 3/4< 13/16
B. 5/8 < 7/12 < 13/16 < 3/4
C. 5/8 < 3/4< 13/16 < 7/12
D. 7/12 < 5/8 < 3/4< 13/16
- A. 6791.59775
B. 6891.59775
C. 6891.59675
D. 5891.59775
- A. 5000
B. 500
C. 50000
D. 50
- A. 80%
B. 90%
C. 10%
D. 50%
- A. 1/12
B. 1/8
C. 1/6
D. 1/4
- A. Rs.500
B. Rs.750
C. Rs.1250
D. Rs.1500
- A. Rs. 14,000
B. Rs. 12,000
C. Rs. 30,000
D. Rs. 40,000
- A. 20%
B. 18%
C. 15%
D. 12%
- A. 70 km/hr
B. 75 km/hr
C. 84 km/hr
D. 87.5 km/hr
- A. 35.55 km/hr
B. 36 km/hr
C. 71.11 km/hr
D. 71 km/hr
- A. 5 kmph
B. 6 kmph
C. 6.25 kmph
D. 7.5 kmph
- A. 14 km
B. 15 km
C. 16 km
D. 17 km
- A. 300 kmph
B. 360 kmph
C. 600 kmph
D. 720 kmph
- A. 225 cm[latex]^ {2}[/latex]
B. 275 cm[latex]^ {2}[/latex]
C. 285 cm[latex]^ {2}[/latex]
D. 315 cm[latex]^ {2}[/latex]
E. None of these
- A. 1: 96
B. 1: 48
C. 1: 84
D. 1: 68
E. None of these
- A. 27 m
B. 24 m
C. 18 m
D. 21 m
E. None of these
- A. Rs. 3642.40
B. Rs. 3868.80
C. Rs. 4216.20
D. Rs. 4082.40
E. None of these
- A. 600 cm
B. 800 cm
C. 400 cm
D. 1000 cm
E. None of these
- A. 906 km
B. 960 m
C. 960 km
D. 966 km
- A. 10 sec
B. 11 sec
C. 12 sec
D. 14 sec
- A. 90 kmph
B. 108 kmph
C. 120 kmph
D. 88 kmph
- A. 10 min
B. 11 min
C. 12 min
D. 13 min
- A. 300 km
B. 400 km
C. 600 km
D. None
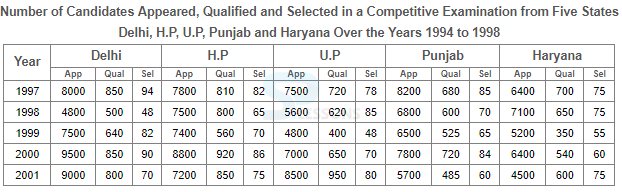
- A. Delhi
B. H.P
C. U.P
D. Punjab
- A. 1997
B. 1998
C. 1999
D. 2000
- A. Delhi
B. H.P
C. U.P
D. Punjab
- A. 79.5%
B. 81%
C. 84.5%
D. 88.5%
- A. 10%
B. 11%
C. 12%
D. 13%
- A. 16 4/3%
B. 100/3%
C. 16%
D. 16 2/3%
- A. 18%
B. 9%
C. 15%
D. 6%
- A. Rs.357
B. Rs.340
C. Rs.342
D. Rs.338
- A. 3%
B. 4%
C. 5%
D. 6%
- A. 25%
B. 30%
C. 31.6%
D. 33.5%
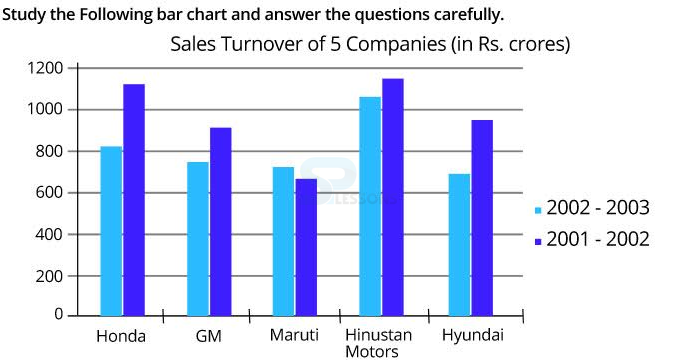
- A. M
B. N
C. P
D. Q
E. R
- A. 12% loss
B. 10% loss
C. 10% profit
D. 12% profit
E. There was no loss or profit
- A. 5% profit
B. 6.5% profit
C. 4% loss
D. 7% loss
E. 10% profit
- A. 28.28
B. 30.30
C. 32.32
D. 34.34
E. 36.36
- A. 35.75
B. 37.25
C. 38.5
D. 41.25
E. 42.75
- A. 7
B. 10
C. 12
D. 13
- A. 20
B. 22
C. 23
D. 26
- A. 22
B. 24
C. 25
D. 26
- A. 12
B. 14
C. 27
D. 53
- A. 14
B. 15
C. 21
D. 23
- A. 4 years
B. 8 years
C. 10 years
D. None of these
- A. 14 years
B. 19 years
C. 33 years
D. 38 years
- A. 7
B. 8
C. 9
D. 10
E. 11
- A. 14 years
B. 18 years
C. 20 years
D. 22 years
- A. 12 years
B. 14 years
C. 18 years
D. 20 years
- A. 7 liters
B. 15 liters
C. 10 liters
D. 9 liters
- A. Rs. 13.50
B. Rs. 14.50
C. Rs. 15.50
D. Rs. 16.50
E. None of these
- A. 80%
B. 100%
C. 84%
D. 87.5%
E. None of these
- A. 40
B. 44
C. 48
D. 52
E. None of these
- A. 2
B. 8
C. 4
D. 5
E. None of these
- A. 19 1/7 min
B. 15 1/7 min
C. 17 1/7 min
D. 7 1/7 min
- A. 9 min
B. 10 min
C. 11 min
D. 12 min
- A. 480 liters
B. 600 liters
C. 720 liters
D. 800 liters
- A. 7 min
B. 13 min
C. 23 min
D. 9 min
- A. 2 hrs 15 min
B. 4 hrs 24 min
C. 5 hrs
D. 3 hrs
- A. 256, 289
B. 252, 279
C. 262, 289
D. 272, 292
- A. 324
B. 369
C. 144
D. 342
- A. 213444
B. 214344
C. 214434
D. 231444
- A. 13.41
B. 20.46
C. 21.66
D. 22.35
- A. 57
B. 67
C. 77
D. 87
- A. [latex]\frac{1}{2}[/latex]
B. [latex]\frac{2}{5}[/latex]
C. [latex]\frac{8}{15}[/latex]
D. [latex]\frac{9}{20}[/latex]
- A. [latex]\frac{1}{3}[/latex]
B. [latex]\frac{3}{4}[/latex]
C. [latex]\frac{7}{19}[/latex]
D. [latex]\frac{8}{21}[/latex]
E. [latex]\frac{9}{21}[/latex]
- A. [latex]\frac{3}{4}[/latex]
B. [latex]\frac{1}{4}[/latex]
C. [latex]\frac{3}{8}[/latex]
D. [latex]\frac{7}{8}[/latex]
- A. [latex]\frac{1}{2}[/latex]
B. [latex]\frac{3}{4}[/latex]
C. [latex]\frac{3}{8}[/latex]
D. [latex]\frac{5}{16}[/latex]
- A. [latex]\frac{1}{10}[/latex]
B. [latex]\frac{2}{5}[/latex]
C. [latex]\frac{2}{7}[/latex]
D. [latex]\frac{5}{7}[/latex]